There are two electric dipoles P1 & P2 kept in two different uniform electric fields E1 & E2 resp. Find ratio of maximum torques acting on both dipoles it is given that P1P2=12 & E1E2=13
44621
44713
44774
4
To solve the problem of finding the ratio of maximum torques acting on two electric dipoles P1 and P2 in uniform electric fields E1 and E2, we can follow these steps:
Step 1: Understand the formula for torque
The torque τ acting on an electric dipole in an electric field is given by the formula:
τ=P⋅E⋅sinθ
where:
- P is the electric dipole moment,
- E is the electric field strength,
- θ is the angle between the dipole moment and the electric field.
Step 2: Determine the maximum torque
The maximum torque occurs when sinθ=1 (i.e., when θ=90∘). Therefore, the maximum torque τmax can be expressed as:
τmax=P⋅E
Step 3: Write the expressions for maximum torques
For dipole P1 in electric field E1:
τmax,1=P1⋅E1
For dipole P2 in electric field E2:
τmax,2=P2⋅E2
Step 4: Find the ratio of maximum torques
We need to find the ratio τmax,1τmax,2:
τmax,1τmax,2=P1⋅E1P2⋅E2
Step 5: Substitute the given ratios
We are given:
P1P2=12andE1E2=13
Now substituting these ratios into the torque ratio:
τmax,1τmax,2=P1P2⋅E1E2=12⋅13=16
Step 6: Final ratio of maximum torques
Thus, the ratio of maximum torques acting on the two dipoles is:
τmax,1τmax,2=16
Conclusion
The final answer is:
τmax,1:τmax,2=1:6
The torque τ acting on an electric dipole in an electric field is given by the formula:
τ=P⋅E⋅sinθ
where:
- P is the electric dipole moment,
- E is the electric field strength,
- θ is the angle between the dipole moment and the electric field.
The maximum torque occurs when sinθ=1 (i.e., when θ=90∘). Therefore, the maximum torque τmax can be expressed as:
τmax=P⋅E
For dipole P1 in electric field E1:
τmax,1=P1⋅E1
τmax,2=P2⋅E2
We need to find the ratio τmax,1τmax,2:
τmax,1τmax,2=P1⋅E1P2⋅E2
We are given:
P1P2=12andE1E2=13
Now substituting these ratios into the torque ratio:
τmax,1τmax,2=P1P2⋅E1E2=12⋅13=16
Thus, the ratio of maximum torques acting on the two dipoles is:
τmax,1τmax,2=16
The final answer is:
τmax,1:τmax,2=1:6
Topper's Solved these Questions
Similar Questions
Knowledge Check
The torque τ acting on an electric dipole of dipole momtn →pin an electric field →E is
A→τ=→p.→EB→τ=→p×→EC→τ=p.→ED→τ=→pEAn electric dipole of dipole moment p is placed in a uniform external electric field E. Then, the
Atoeque experienced by the dipole is E×pBtorque is zero, if p is perpendicular to ECtorque is maximum, if p is perpendicular to EDpotential energy is maximum, if p is parallel to EA dipole of dipole moment →P is placed in uniform electric field →E, then torque acting on it is given by
A→τ=→P.→EB→τ=→P×→EC→τ=→P+→ED→τ=→P−→E
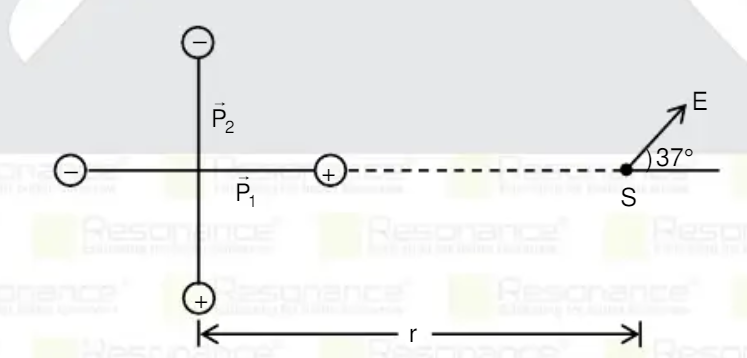
Two electric dipole −→P1 and −→P2 are kept as shown in figure. Net electric field at point S is E makes an angle 370 with −→P1 then find ratio of −→P1 and −→P2
View SolutionIf an electric dipole of moment p is placed in an electric field E .the torque acting on the dipole will be ".................
View SolutionDerive an expression for the work done in rotating an electric dipole of dipole moment 'p' in a uniform electric field 'E' from an orientation θ1 to θ2.
View SolutionAn electric dipole of dipole moment p is aligned parallel to a uniform electric field E. the energy required to rotate the dipole by 90∘ is
View SolutionElectric dipole of moment →p=pˆi is kept at a point (x, y) in an electric field →E=4xy2ˆi+4x2yˆj. Find the magnitude of force acting on the dipole.
View Solution
