A gas of degree of freedom 8 at a certain temperature expands isobarically and does work 1500J. Find absorbed heat
a.5500J
b.6500J
c.7500J
d.8500J
To solve the problem, we need to find the absorbed heat Q by the gas during an isobaric expansion where the gas does work W=1500J and has a degree of freedom f=8.
1. Understanding the Process:
In an isobaric process (constant pressure), the first law of thermodynamics states:
Q=ΔU+W
where Q is the heat absorbed, ΔU is the change in internal energy, and W is the work done by the gas.
2. Change in Internal Energy:
The change in internal energy for an ideal gas is given by:
ΔU=f2nRΔT
where f is the degree of freedom, n is the number of moles, R is the universal gas constant, and ΔT is the change in temperature.
3. Work Done:
The work done by the gas during an isobaric process can also be expressed as:
W=PΔV=nRΔT
where P is the pressure and ΔV is the change in volume.
4. Substituting Work into the First Law:
We can substitute W into the first law equation:
Q=ΔU+W=f2nRΔT+nRΔT
This simplifies to:
Q=nRΔT(f2+1)
5. Calculating the Factor:
Given f=8:
f2+1=82+1=4+1=5
Thus, we have:
Q=nRΔT⋅5
6. Relating Work to Temperature Change:
From the work equation W=nRΔT, we can express nRΔT in terms of work:
nRΔT=W
Therefore, substituting this into the equation for Q:
Q=5W
7. Substituting the Given Work:
We know W=1500J:
Q=5×1500=7500J
Final Answer:
The absorbed heat Q is 7500J.
In an isobaric process (constant pressure), the first law of thermodynamics states:
Q=ΔU+W
where Q is the heat absorbed, ΔU is the change in internal energy, and W is the work done by the gas.
The change in internal energy for an ideal gas is given by:
ΔU=f2nRΔT
where f is the degree of freedom, n is the number of moles, R is the universal gas constant, and ΔT is the change in temperature.
The work done by the gas during an isobaric process can also be expressed as:
W=PΔV=nRΔT
where P is the pressure and ΔV is the change in volume.
We can substitute W into the first law equation:
Q=ΔU+W=f2nRΔT+nRΔT
This simplifies to:
Q=nRΔT(f2+1)
Given f=8:
f2+1=82+1=4+1=5
Thus, we have:
Q=nRΔT⋅5
From the work equation W=nRΔT, we can express nRΔT in terms of work:
nRΔT=W
Therefore, substituting this into the equation for Q:
Q=5W
We know W=1500J:
Q=5×1500=7500J
The absorbed heat Q is 7500J.
Topper's Solved these Questions
Similar Questions
Five moles of an ideal monoatomic gas with an initial temperature of 127∘C expand and in the process absorb 1200J of heat and do 2100J of work. What is the final temperature of the gas?
View SolutionA gas with 3 degrees of freedom performs 45J of work while expanding at constant pressure. The heat absorbed by it is _____J
View Solution
Knowledge Check
If a gas has n degrees of freedom ratio of specific heats of gas is
A1+n2B1+1nC1+n2D1+2nIf a gas has 5 degrees of freedom ratio of specific heats of gas 5
A5B53C32D75A piston filled with 0.04 mol of an ideal gas expands reversibly from 50.0mL to 375mL at a constant temperature of 37.0∘C. As it does so, it absorbs 208J of heat. The value of q and w for the process will be:
(R=8.314J/molK)(ln7.5=2.01)Aq=+208J,W=−208JBq=−208J,W=−208JCq=−208J,W=+208JDq=+208J,W=+208J
An ideal gas expands isothermally along AB and does 700J of work. How much heat does the gas exchange along AB? The gas then expands adiabarically along BC and does 400J of work. When the gas returns to A along CA, it exhausts 100J of heat to the surroundings. How much work is done on the gas along this path?
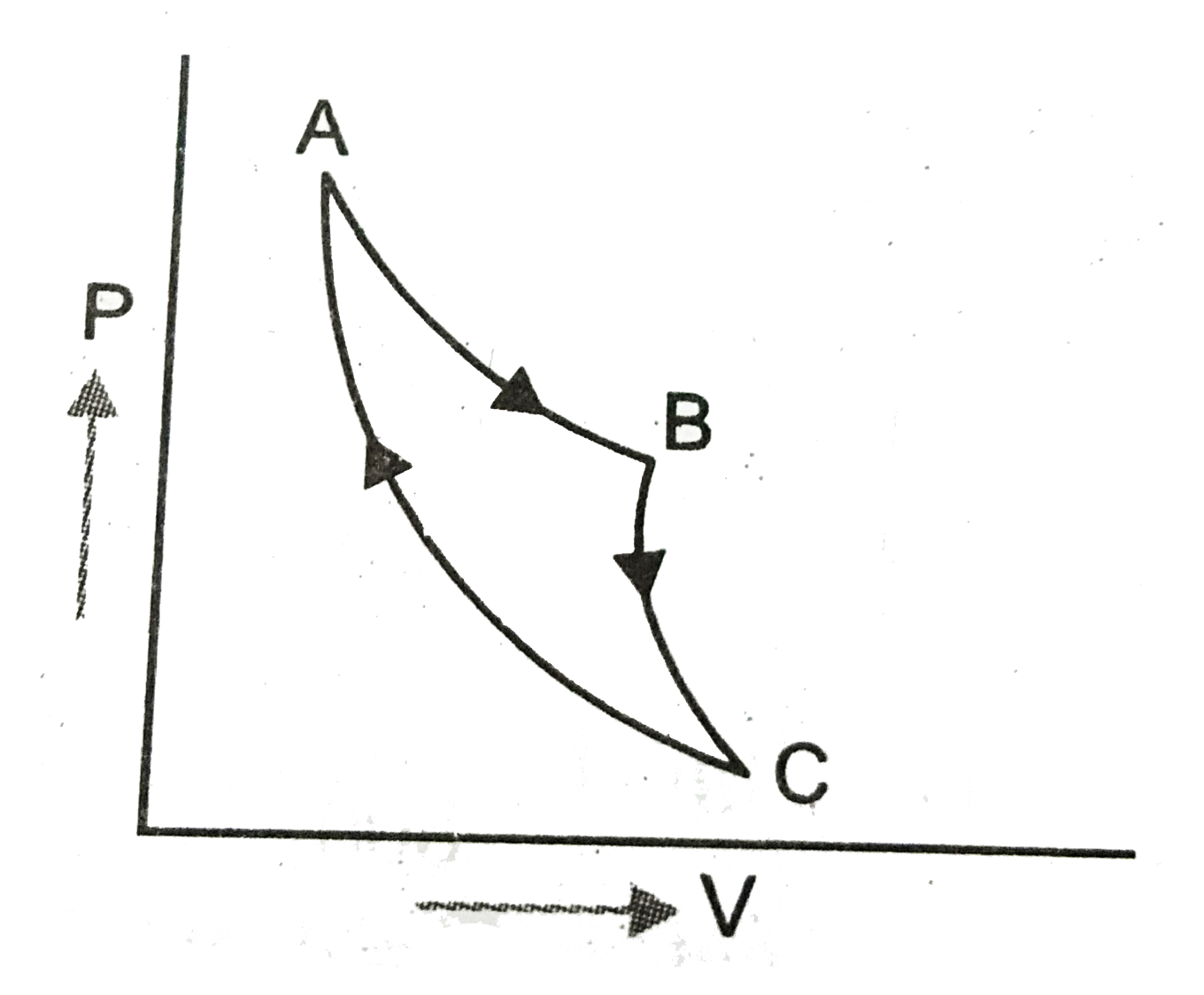
View SolutionAn ideal gas consisting of N−atomic molecules is expanded isobarically. Assuming that all degrees of freedom (translational, rotational, and vibrational) of the molecules are excited, find what fraction of heat transferred to the gas in this process is spend to perform the work of expansion. Now high is this fraction in the case of monatomic gas ?
View SolutionFive moles of an ideal monoatomic gas with an initial temperature of 150∘C expand and in the process absorb 1500 J of heat and does 2500 J of work. The final temperature of the gas in .∘C is (ideal gas constant R=8.314JK−1mol−1)
View SolutionThe degrees of freedom of a triatomic gas is
View SolutionTotal degrees of freedom of one molecule of a diatomic gas at normal temperature is
View Solution
