Assertions (A): All trigonometric functions have their inverses over their respective domains.
Reason (R) : The inverse of tan−1x exists for some x∈R
Both Assertion (A) and Reason (R) are true and Reason (R) is the correct explanation of the Assertion (A).
Both Assertion (A) and Reason (R) are true , but Reason (R) is not the correct explanation of the Assertion (A).
Assertion (A) is true, but Reason (R) is false.
Assertion (A) is false, but Reason (R) is true.
To solve the given question, we need to analyze both the assertion (A) and the reason (R) provided.
Step 1: Analyze the Assertion (A)
The assertion states: "All trigonometric functions have their inverses over their respective domains."
- Explanation: Trigonometric functions such as sine, cosine, and tangent are periodic functions, meaning they repeat their values in regular intervals. Because of this periodic nature, they are not one-to-one functions over their entire domain, which is necessary for a function to have an inverse. Therefore, the assertion is false.
Step 2: Analyze the Reason (R)
The reason states: "The inverse of tan−1x exists for some x∈R."
- Explanation: The function tan−1x (or arctan) is indeed a well-defined function that exists for all real numbers (x ∈ ℝ). The arctangent function is one-to-one and covers all real numbers, which means it has an inverse. Therefore, the reason is true.
Step 3: Conclusion
Since the assertion (A) is false and the reason (R) is true, we can conclude that:
- Assertion (A) is false.
- Reason (R) is true.
Thus, the correct option would be the one that indicates that the assertion is false and the reason is true.
Final Answer
The assertion is false, and the reason is true.
The assertion states: "All trigonometric functions have their inverses over their respective domains."
The reason states: "The inverse of tan−1x exists for some x∈R."
Since the assertion (A) is false and the reason (R) is true, we can conclude that:
- Reason (R) is true.
The assertion is false, and the reason is true.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The inverse of the function loge x is
A10xB10−xCexDe−xv34
Ax=y1y∈5,y>0Bx=y∈5,y>0Cx=yt∈5,y<0Dx=y∈y,y>0A condition for a function y=f(x) to have an inverse is that it should be
Adefined for all xBcontinuous for all xCstrictly monotone and continuous in the domainDan even function
Find the inverse to the function y=3x+5.
View SolutionDomain/range graphs for inverse trigonometric functions
View SolutionAre the following functions invertible in their respective domains ? If so, find the inverse in each case :
(i) f(x) = x + 1
(ii) f(x)=x−1x+1,x≠−1.
View SolutionThe function whose graph is the reflection about the line x+y=0 of the inverse function f−1 of a function f(x) is
View SolutionWhile defining inverse trigonometric functions , a new system is followed where co-domains has been redefined as follows :
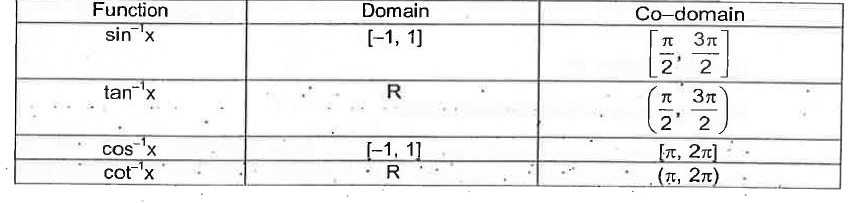
sin−1(−x) is equal to
View Solution
