In a pool at an aquarium, a dolphin jumps out of the water travelling at 20cm per second. Its heigth above water level after t second is given by h=20t−16t2.

Based on the above, answer the following questions:
(i)Find zeroes of polynomial p(t)=20t−16t2
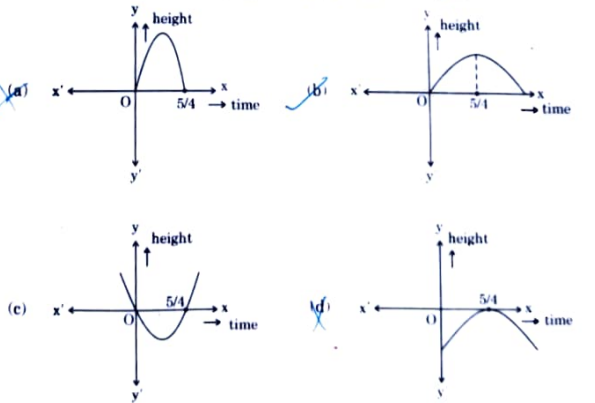
(ii)Which of the following types of graph represents p(t)?
(iii) (a) What would be the value of h at t=32? Interpret the result.
(iii) (b) How much distance has the dolphin covered before hitting the water level again ?
Step by step video solution for In a pool at an aquarium, a dolphin jumps out of the water travelling at 20cm per second. Its heigth above water level after t second is given by h=20t-16t^2.Based on the above, answer the following questions:(i)Find zeroes of polynomial p(t)=20t-16t^2(ii)Which of the following types of graph represents p(t)?(iii) (a) What would be the value of h at t=3/2? Interpret the result.(iii) (b) How much distance has the dolphin covered before hitting the water level again ? by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
The distance travelled s in metres by a particle in t second is given by s=t3+2t2+t. The speed of the particle after 1s will be
A8cms−1B6cms−1C2cms−1DNone of theseIf the distance 's' metres travelled by a particle in t seconds is given by s=t3−3t2, then the acceleration is zero (in m/s) is
A3B−2C−3D2The distance s (in cm) travelled by a particle in t seconds is given by, s=t3+2t2+t. The speed of the particle after 1s will be :
A2cm/sB8cm/sC6cm/sDnone of these
The distance f(t) in metres ived by a particle travelling in a straight line in t seconds is given by f(t)=t2+3t+4. Find the speed of the particle at the end of 2 seconds.
View SolutionThe equation of motion of a missile are x = 3t, y = -4t, z = t, where the time ‘t’ is given in seconds, and the distance is measured in kilometres.

Based on the above answer the following:
What is the path of the missile?
View SolutionThe distance s (in cm) travelled by a particle in t seconds is given by, s=t3+2t2+t. The speed of the particle after 1s will be :
View SolutionWhich of the following graphs represents zero order if
A→P
At t=0⇒[A]0
At t=t⇒[A]t
View SolutionThe distance covered by a particle in t second is given by x=3+8t−4t2. After 1s its velocity will be
View Solution