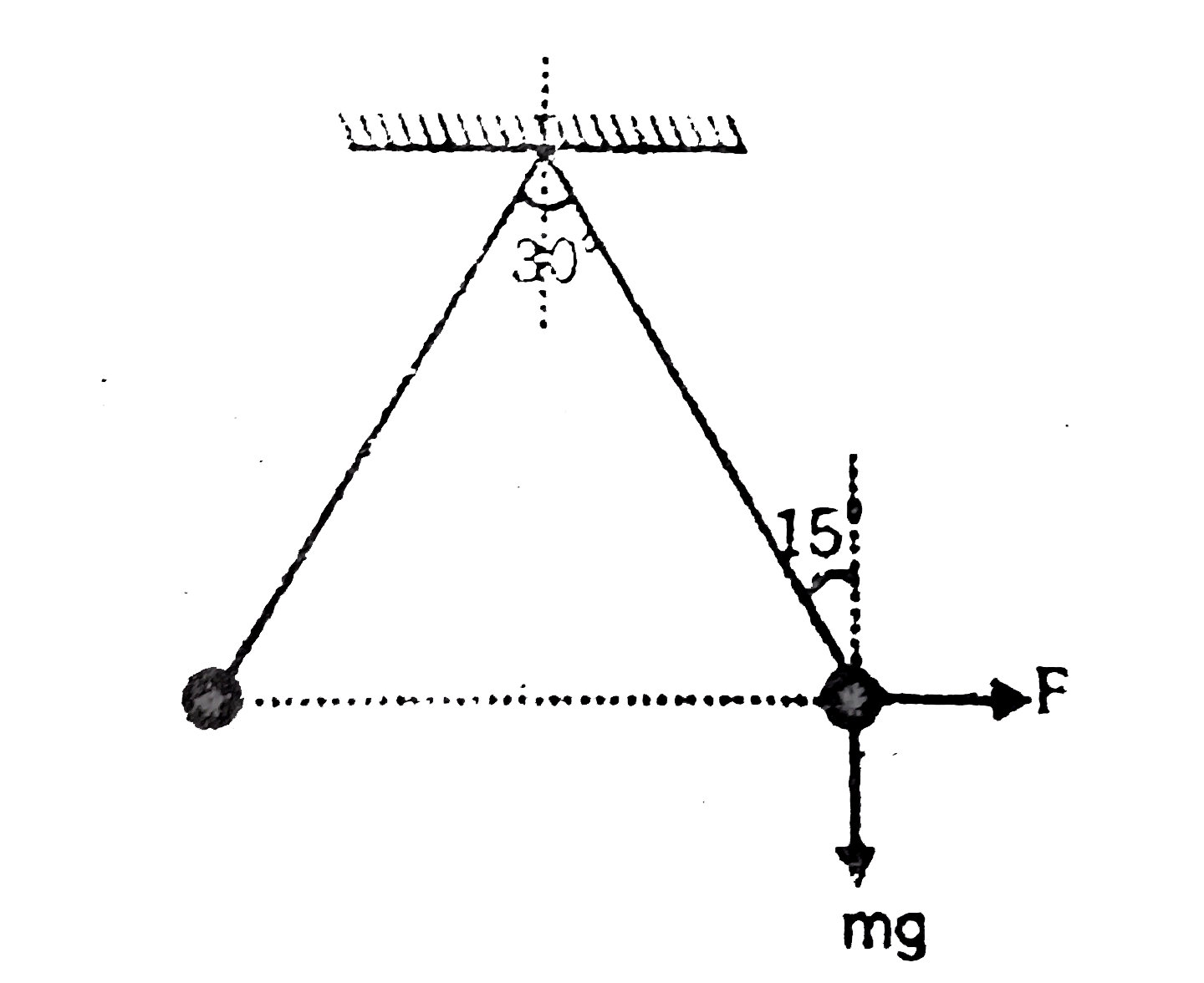
Two identical charged spheres are suspended by strings of equal lengths. The strings make an angle θ with each other. When suspended in water the angle remains the same. If density of the material of the sphere is 1.5 g/cc, the dielectric constant of water will be ________ (Take density of water = 1 g/cc)
The correct Answer is:3
To solve the problem, we need to find the dielectric constant of water based on the given conditions. Here's a step-by-step solution:
Step 1: Understand the Forces Acting on the Spheres
When the charged spheres are suspended, they experience two main forces:
1. The gravitational force acting downwards (weight).
2. The electric force acting horizontally due to the repulsion between the identical charges on the spheres.
Step 2: Set Up the Force Balance
Let:
- ρ = density of the material of the sphere = 1.5 g/cc
- ρw = density of water = 1 g/cc
- Vg = volume of the sphere
- FE = electric force between the spheres
- Fg = weight of the sphere = ρ⋅Vg⋅g
The angle θ between the strings can be expressed in terms of the forces:
tan(θ)=FEFg
Step 3: Analyze the Situation in Air and Water
When the spheres are suspended in air, the force balance can be written as:
tan(θ)=FEρ⋅Vg⋅g
When the spheres are submerged in water, the effective weight of the spheres changes due to the buoyant force:
F′g=ρ⋅Vg⋅g−ρw⋅Vg⋅g=(ρ−ρw)⋅Vg⋅g
The angle remains the same, so we have:
tan(θ)=FE(ρ−ρw)⋅Vg⋅g
Step 4: Set the Two Equations Equal
Since tan(θ) is the same in both cases, we can set the two equations equal to each other:
FEρ⋅Vg⋅g=FE(ρ−ρw)⋅Vg⋅g
Step 5: Simplify the Equation
Cancelling FE and Vg⋅g from both sides gives:
1ρ=1ρ−ρw
Step 6: Substitute Known Values
Substituting ρ=1.5 g/cc and ρw=1 g/cc:
11.5=11.5−1
Step 7: Solve for Dielectric Constant K
From the above equation, we can express the electric force in terms of the dielectric constant K:
K=ρρ−ρw
Substituting the values:
K=1.51.5−1=1.50.5=3
Conclusion
Thus, the dielectric constant of water is:
3
When the charged spheres are suspended, they experience two main forces:
1. The gravitational force acting downwards (weight).
2. The electric force acting horizontally due to the repulsion between the identical charges on the spheres.
Let:
- ρ = density of the material of the sphere = 1.5 g/cc
- ρw = density of water = 1 g/cc
- Vg = volume of the sphere
- FE = electric force between the spheres
- Fg = weight of the sphere = ρ⋅Vg⋅g
tan(θ)=FEFg
When the spheres are suspended in air, the force balance can be written as:
tan(θ)=FEρ⋅Vg⋅g
F′g=ρ⋅Vg⋅g−ρw⋅Vg⋅g=(ρ−ρw)⋅Vg⋅g
tan(θ)=FE(ρ−ρw)⋅Vg⋅g
Since tan(θ) is the same in both cases, we can set the two equations equal to each other:
FEρ⋅Vg⋅g=FE(ρ−ρw)⋅Vg⋅g
Cancelling FE and Vg⋅g from both sides gives:
1ρ=1ρ−ρw
Substituting ρ=1.5 g/cc and ρw=1 g/cc:
11.5=11.5−1
From the above equation, we can express the electric force in terms of the dielectric constant K:
K=ρρ−ρw
Substituting the values:
K=1.51.5−1=1.50.5=3
Thus, the dielectric constant of water is:
3
Topper's Solved these Questions
Similar Questions
Two identically charged spheres are suspended by strings of equal length. The strings make an angle of 30∘ with each other. When suspended in a liquid of density 0.8g/cc the angle remains same. What is the dielectric constant of liquid. Density of sphere=1.6g/cc
 View Solution
View SolutionIf degree of dissociation of pure water at 100∘C is 1.8×10−8 , then the dissociation constant of water will be: (Density of H2O = 1 g/cc)
View Solution
Knowledge Check
- Question 1 - Select One
Two identical charged spheres are suspended by strings of equal lengths. The strings make an angle of 30∘ with each other. When suspended in a liquid of density 0.8gcm−3, the angle remains the same. If density of the material of the sphere is 1.6gcm−3, the dielectric constant of the liquid is
A(a) 4B(b) 3C(c) 2D(d) 1 - Question 2 - Select One
Two identical charged spheres of material density ρ, suspended from the same point by inextensible strings of equal length make an angle θ between the string. When suspended in a liquid of density σ the angle θ remains the same. The dielectric constant K of the liquid is
Aρρ−σBρ−σρCρρ+σDρ+σρ - Question 3 - Select One
Two identical charged spheres are suspended in air from a ceiling using strings of equal length. The point of suspension is common to both the spheres. Neglect the density of air. The density of material of spheres is 2g/cm3.
Both spheres repel each other and the strings are inclined at a certain angle with the vertical when the system is in equilibrium. Now both the sphere are dipped into a liquid of specific gravity 1 and dielectric constant K, but angle that the strings are making with the vertical remains unchanged. Let T1 be the tension in both the strings when the spheres are in air and T2, be the tension in strings when spores are dipped in liquid. Let F1 be the electrostatic force between charges when they were kept in air and F2, be the electrostatic force when spheres are dipped in liquid.
The magnitude of F2/F1 isA3B2C1/2D1/3
Two identical ball having mass density ρ , mass m and charge q are suspended from a common point by two insulating massless strings of equal length. At equilibrium each string makes an angle theta with the vertical. Now both the balls are immersed in a fluid. At equilibrium, theta remains same. If the mass density of liquid is σ then dielectric constant of liquid will be
View SolutionTwo identical point charges q of mass m each are suspended through strings of equal length l from a common point of suspension. Find the charge q if angle subtended by the strings with the vertical is θ, when point charges are in a state of equilibrium.
View SolutionTwo identical charged spheres are suspended in air from a ceiling using strings of equal length. The point of suspension is common to both the spheres. Neglect the density of air. The density of material of spheres is 2g/cm3.
Both spheres repel each other and the strings are inclined at a certain angle with the vertical when the system is in equilibrium. Now both the sphere are dipped into a liquid of specific gravity 1 and dielectric constant K, but angle that the strings are making with the vertical remains unchanged. Let T1 be the tension in both the strings when the spheres are in air and T2, be the tension in strings when spores are dipped in liquid. Let F1 be the electrostatic force between charges when they were kept in air and F2, be the electrostatic force when spheres are dipped in liquid.
The magnitude of T2/T1 isView SolutionTwo small identical balls are suspened from a common point by two identical strings. When they are given identical charges, they move apar and the strings make with the vertical an angle of q. Now the system is immersed in a liquid and as a result the angle q does not change. If r is the density of the material of the balls and s is the density of the liquid, the dielectric constant of the liquid is
View SolutionDensity of water is less than 1g/c.c ______
View Solution

