For Q. 25 find the minimum constant force to be applied to body of mass m1 in order to shift body of mass m2. Take m1=m2=10kg,μ=0.5
F.x=μm1gx−12kx2=0 ...(i)
But Kx=μ2g. (for just moving body of mass m2)
Put the value of K in (i) to get F.
F.x −μm1gx−12kx2=0
But kx=μm2g (for moving body of mass m2)
Fx−μm1gx−12(μm2gx)x2=0
F=μm1g+12μm2g
=μ(m1+m22)g
=0.5(10+102)10
=75N
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-A (OBJECTIVE TYPE QUESTIONS (ONE OPTIONISCORRECT)
60 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-B (SUBJECTIVE TYPE QUESTIONS) (ONE OPTIONS IS CORRECT)
44 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - IILUSTRATION
2 Videos
- WAVESBOOK - AAKASH INSTITUTECHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
Two blocks of masses m1=10kg and m2=20kg are connected by a spring of stiffness k = 200 N/m. The coefficient of friction between the blocks and the fixed horizontal surface is μ=0.1. Find the minimum constant horizontal force F (in Newton) to be applied to m1 in order to slide the mass m2. (Take g=10m/s2)
View SolutionTwo blocks of masses m1=10kg and m2=20kg are connected by a spring of stiffness k = 200 N/m. The coefficient of friction between the blocks and the fixed horizontal surface is μ=0.1. Find the minimum constant horizontal force F (in Newton) to be applied to m1 in order to slide the mass m2. (Take g=10m/s2)
View Solution
Knowledge Check
Two block of masses m1 and m2 connected by a light spring rest on a horizontal plane. The coefficient of friction between the block and the surface is equal to μ. What minimum constant force has to be applied in the horizontal direction to the block of mass m1 in order to shift the other block?
A8 NB15 NC10 ND25 NTwo blocks of masses m1 and m2 are connected by a spring having spring constant K. Initially , the spring is at its natural length. The coefficient of friction between the blocks and the surface is μ. What minimum constant force has to be applied in the horizontal direction to the block of mass m1, in order to shift the other block ?
AF=μ(m1+m22)gBF=μ(m12+m2)gCF=μ(m21+m222)gDF=μ2(m21+m22)g
Two bodies of masses m1andm2 are connected by a non-deformed light speing and lie on a horizontal plane. Coefficient of friction between the surface and the blocks is μ. Find an expression for the work done by the various foeces. Force F is applied horzontally to body of mass m1 in order to shift body of mass m2.
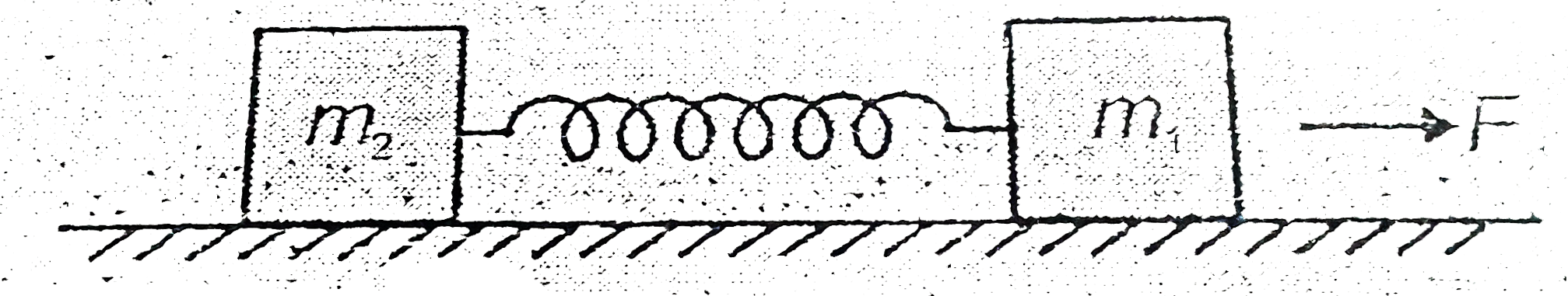
View SolutionTwo bodies of masses m1andm2 are connected by a non-deformed light speing and lie on a horizontal plane. Coefficient of friction between the surface and the blocks is μ. Find an expression for the work done by the various foeces. Force F is applied horzontally to body of mass m1 in order to shift body of mass m2.

View SolutionTwo blocks of masses M1 and M2 connected by a light spring rest on a horizontal plane . The coefficient of friction between the blocks and the surface is equal to μ . The minimum constant force that has to be applied in the horizontal direction to the block of mass M1 in order to shift the other block is (M1+M2α)μg , then α is :
View SolutionTwo blocks of masses m1 and m2 are connected by a spring of stiffness k. The coefficient of friction between the blocks and the surface is μ. Find the minimum constant force F to be applied to m1 in order to just slide the mass m2.
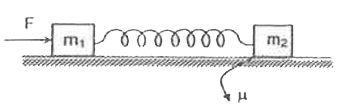
View SolutionTwo blocks of masses m1 and m2 are connected by a spring of stiffness k. The coefficient of friction between the blocks and the surface is μ. Find the minimum constant force F to be applied to m1 in order to just slide the mass m2.

View SolutionTwo block of masses m1 and m2 connected by a light spring rest on a horixontal plane. The cofficient of friction between the block and the surface is equal to μ. What minimum constant force has to be applied in the horizontal direction to the block of mass m1 in order to shift the other block?
View Solution
