Plot the corresponding reference circle for given SHM, indicate the initial (t=0) position of the paritcle, the radius of the circle , and the angular speed of the rotating paritcle. Consider anticlockwise direction for rotation. x=−3sin(2t+π4) (Express in the form , x= Acos ( omega t + phi)).
x=−3sin(2t+π4)
=3xos(π2+(2t+π4)) as cos(90∘+θ)=−sinθ
=3cos(2t+3π4)
Comparing with x=Acos(ωt+ϕ) ,we get
A=3,ω=2,ϕ=π2+π4=3π4
The reference circle is as shown in figure.
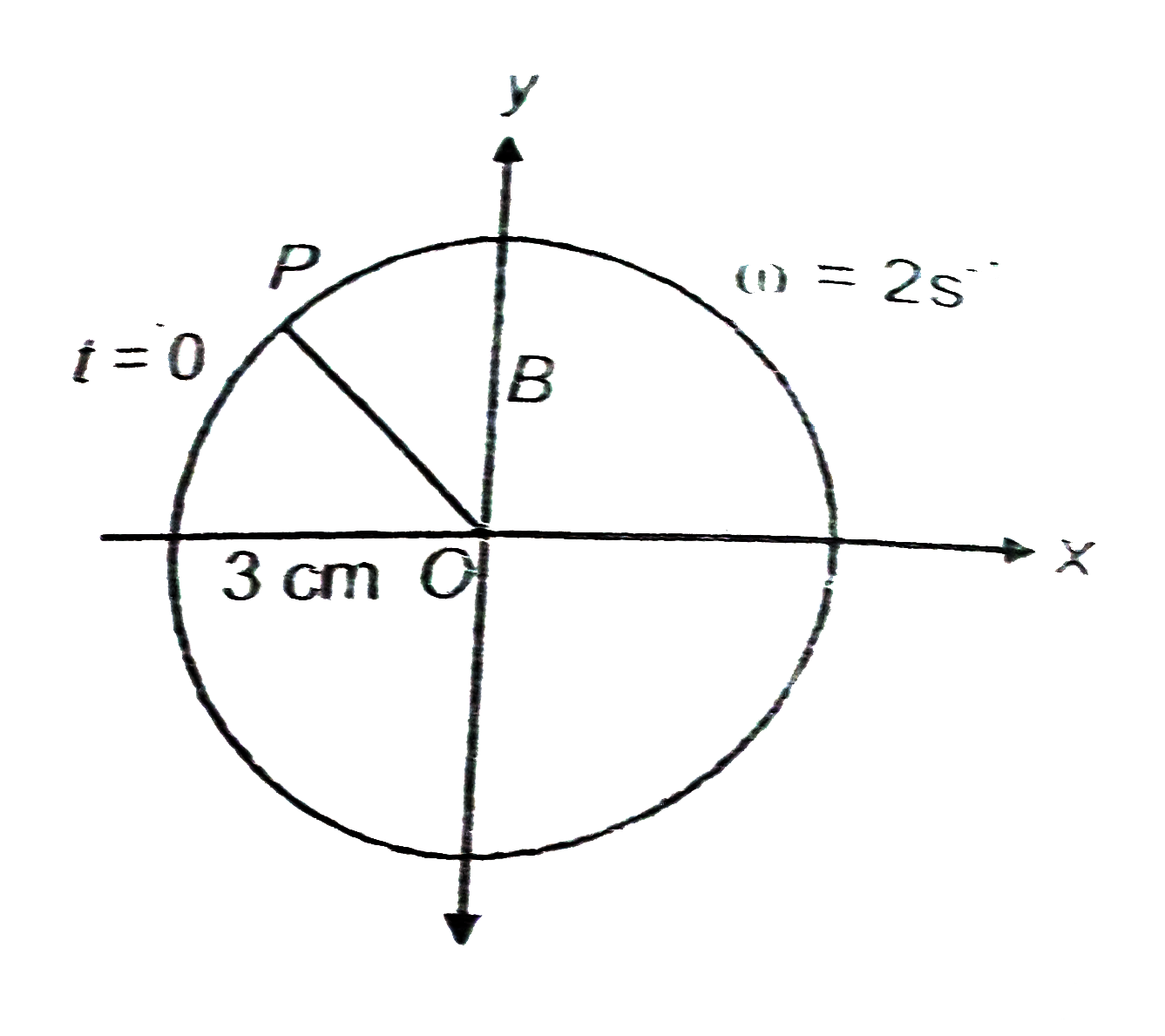
Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - Try Yourself
86 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTECHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT ( SECTION -A)
58 Videos
- NUCLEIBOOK - AAKASH INSTITUTECHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTECHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
5 Videos
Similar Questions
Plot the corresponding reference circle for given SHM x=2cos(π3−t) .Indicate the initial (t−0) position of the particle , the radius of the circle and the angular speed of the rotating particle . Consider anticlockwise direction for rotation.
Hint : x=2cos(π3−t)=2cos(t−π3) as cos(−θ)=cosθ . Compare with x=Acos(ωt+ϕ)
View SolutionPlot the corresponding reference circle for each of the following simple hoarmonic motions. Indicate the intial(t=0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case :(x is in cm and t is in s ).
(a) x=−2sin(3t+π/3) (b)x=cos(π/6−t) (c) x=3sin(2πt+π/4) (d) x=2cosπt
View Solution
Knowledge Check
The displacement of a particle in S.H.M. is given by x=Bsin(ωt+α). The initial position (at time t=0), of the particle is the initial phase angle if the angular frequency of the particle is πrad/s ?
Aπ2Bπ4Cπ6Dπ5Two particles A,B are moving on two concentric circles of radii R1) and R2 with equal angular speed ω. Att=0,their positions and direction fo motion are shown in the figure :
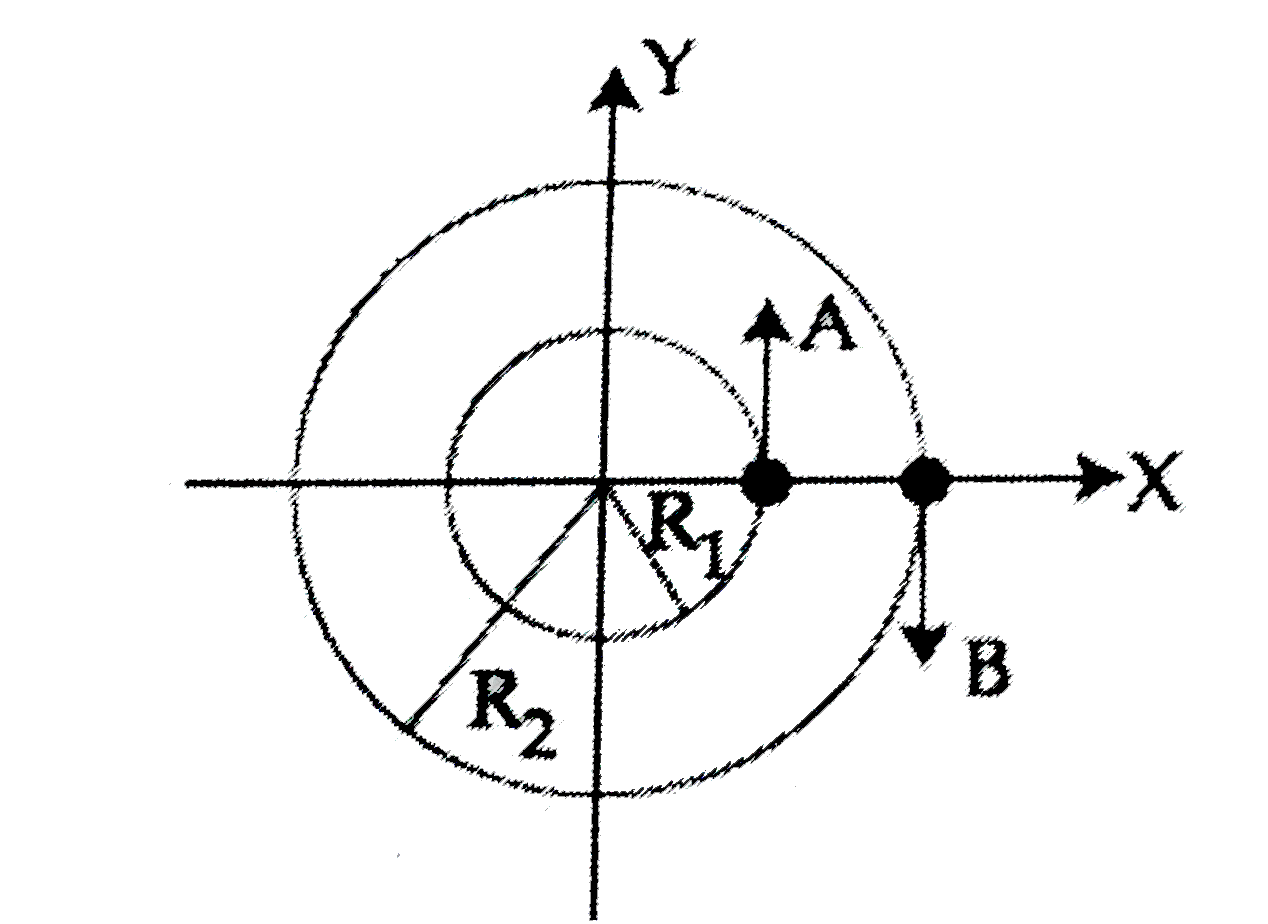 Aω(R1+R2)ˆiB−ω(R1+R2)ˆiCω(R2−R1)ˆiDω(R1−R2)ˆi
Aω(R1+R2)ˆiB−ω(R1+R2)ˆiCω(R2−R1)ˆiDω(R1−R2)ˆiTwo particles A,B are moving on two concentric circles of radii R1) and R2 with equal angular speed ω. Att=0,their positions and direction fo motion are shown in the figure :
 Aω(R1+R2)ˆiB−ω(R1+R2)ˆiCω(R2−R1)ˆiDω(R1−R2)ˆi
Aω(R1+R2)ˆiB−ω(R1+R2)ˆiCω(R2−R1)ˆiDω(R1−R2)ˆi
The corresponding reference circle for a given SHM has been shown in the figure. Given π=7π4 . Write the corresponding equation of SHM. Consider anticlockwise direction for rotation.
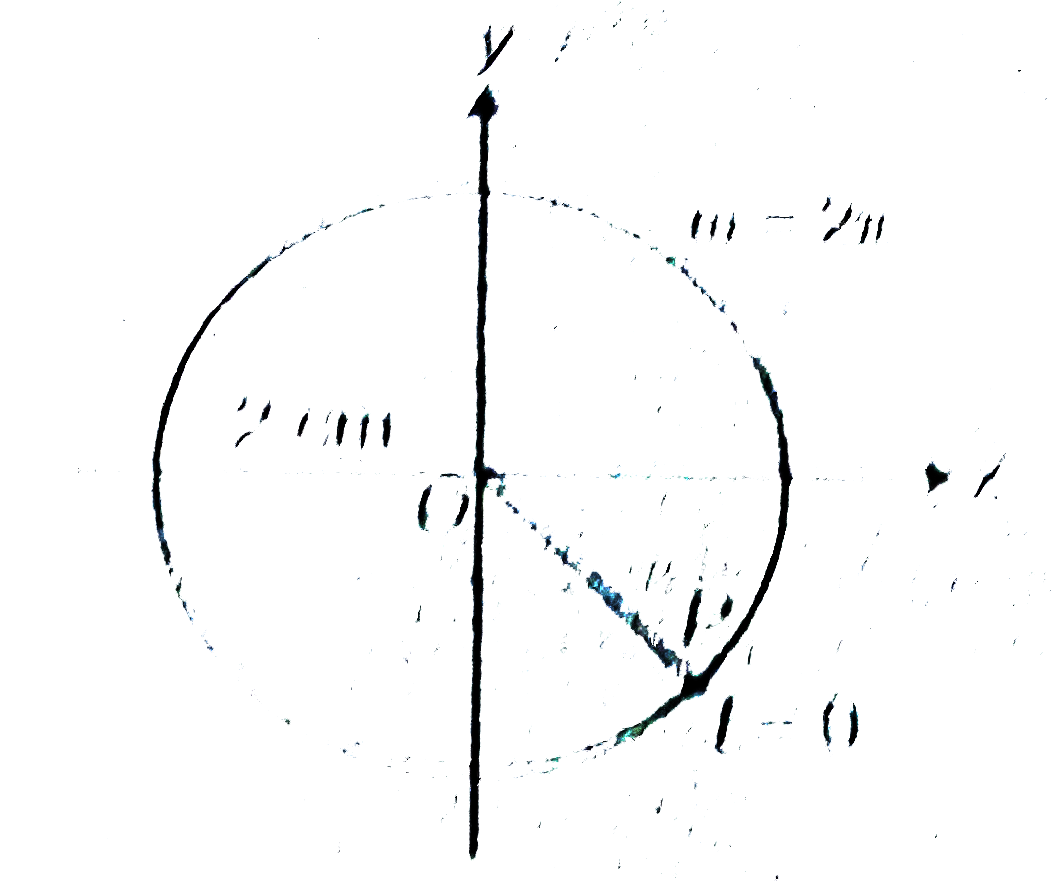
Hint :x=2cos(2πt+7π4)
2cos(3π2+(2πt+π4))
View SolutionA particle is rotating in circle with uniform speed as shown. The angular momentum of the particle w.r.t. origin is :-

View SolutionA disc of radius R start at time t=0 moving along the positive X-axis with linear speed b and angular speed ω. Find the x and y-coordinates of the bottommost point at any time t.
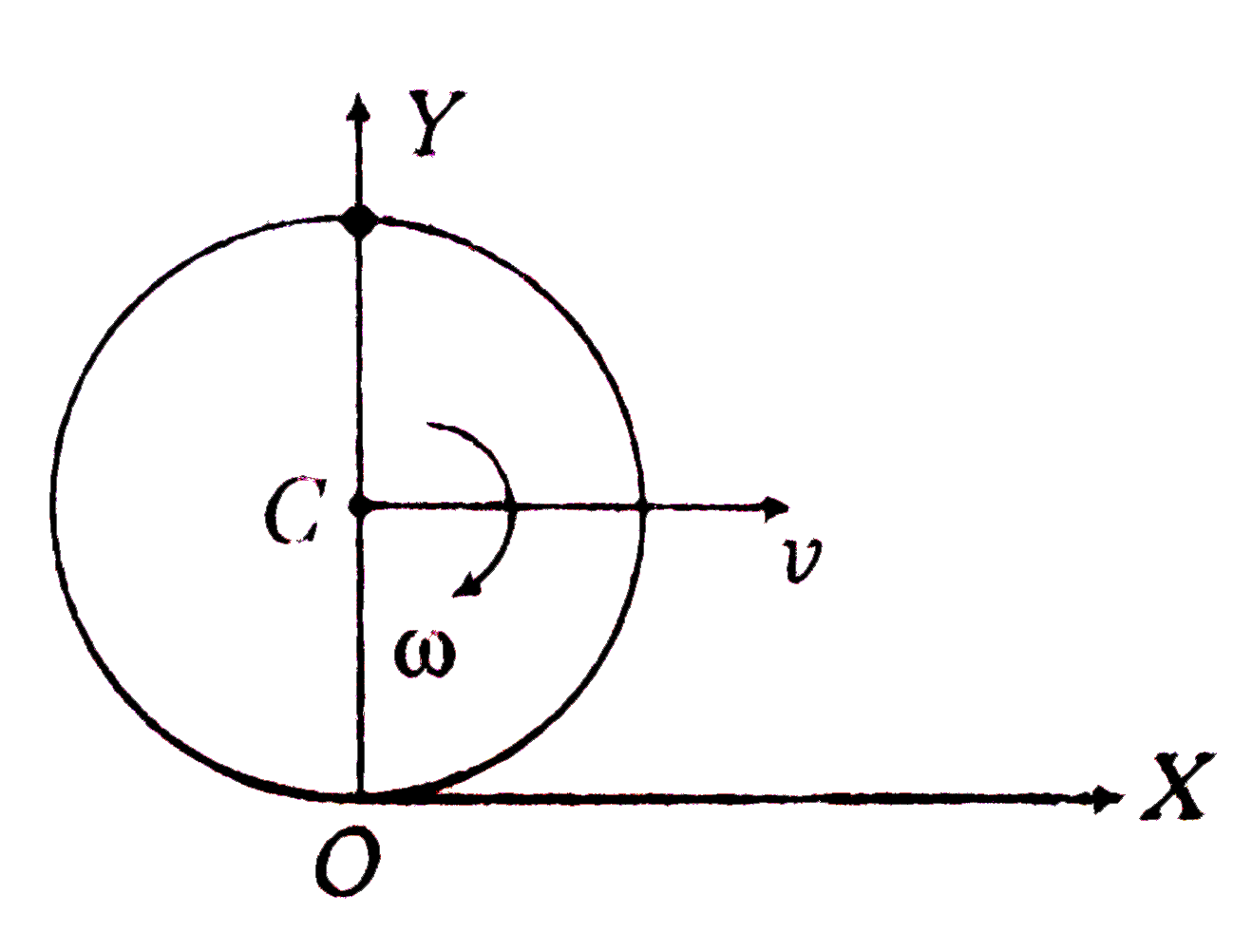
View SolutionFigure shows the circular motion of a particle. The radius of the circle, the period, sense of revolution and the initial position are indicated in the · figure. The simple harmonic motion of the X-projection of the radius vector of the rotating particle Pis
View SolutionA uniform rod of length l is rotating with constant angular speed ω as shown in figure. Choose the graph which correctly shows the variation of T with x.
_2019_CBT7_E01_017_Q01.png)
View Solution
