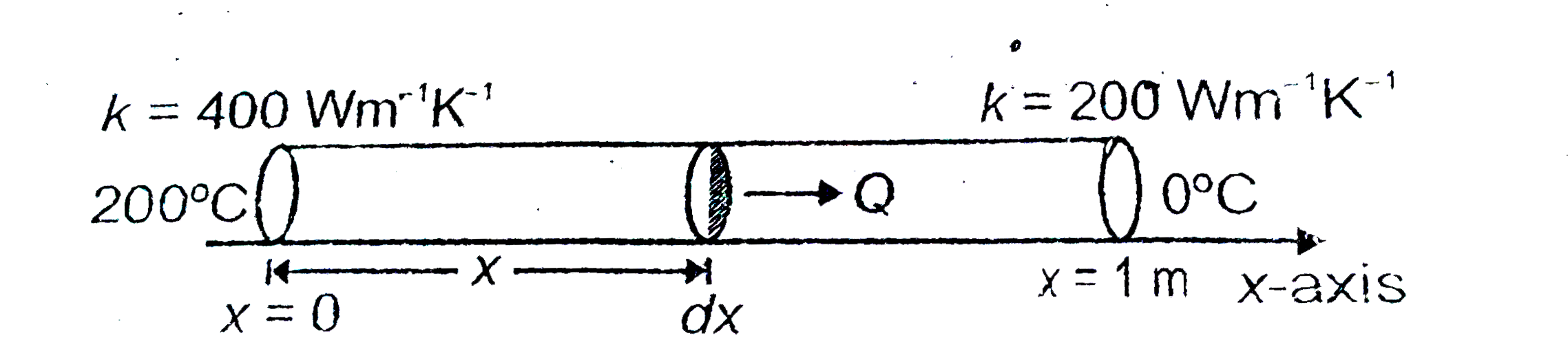
Due to a spatial variation in purity, the thermal conductivity of a metal bar (cross sectional area $4 \times 10^{- 4} m^{2}$ , length 1m) decreases ineraly along its length from 400 $W m^{- 1} K^{- 1}$ at one end, to 200 $W m^{- 1} K^{- 1}$ at the other. Calculate the rate at which heat flows through the bar if the hot end is maintained at $200^{\circ} C$ and the cold end at $0^{\circ} C$

Video Solution

Text Solution

Verified by Experts

As the thermal coductivity is not same throughout the thickness, you cannot apply fourier's law of for the metal bar as a whole. Let one end of the bar be at x=0 and the bar is oriented along x-axis. As the conductivity decreases linearly from 400 $W m^{- 1} K^{- 1}$ to 200 $W m^{- 1} K^{- 1}$ , the conductivity at the cross-section which of thickness dx will be
$Q = - k A \frac{d T}{d x} = - (400 - 200 x) \times 4 \times 10^{- 4} \frac{d T}{d x}$
$\Rightarrow - d T = \frac{Q d x}{4 \times 10^{- 4} (400 - 200 x)} \Rightarrow {(200)}^{0} \int d T = \frac{Q}{4 \times 10^{- 4}} {[ln (400 - 200 x)]}_{0}^{1} \times \frac{1}{(- 200)}$
$\Rightarrow - (0 - 200) = \frac{Q}{4 \times 10^{- 4} \times 200} ln (2)$
or $Q = \frac{200 \times 4 \times 10^{- 4} \times 200}{ln 2} = 23 . ¯ ¯¯ ¯ 08 W$

Updated on:21/07/2023

Three metal rods of same length and same cross - sectional area are connected in parallel. If their conductivities area 70, 110, 180 $W m^{- 1} K^{- 1}$ respectively, the effective conductivity (in $W m^{- 1} K^{- 1})$ of the combination is
A90
B260
C130
D360
The metal rods of same length and same area of cross-section are connected in series. If their condcutivites are $120 W m^{- 1} and 240 W m^{- 1} K^{- 1}$ the effective conductivity of the combination is
A $150 W m^{- 1} K^{- 1}$
B $160 W m^{- 1} K^{- 1}$
C $180 W m^{- 1} K^{- 1}$
D $80 W m^{- 1} K^{- 1}$