A metal rod of cross sectional area 1.0cm2 is being heated at one end. At one time , the temperature gradient is 5.0∘Ccm−1 at cross section A and is 2.5∘Ccm−1 at cross section B. calculate the rate at which the temperature is increasing in the part AB of the rod. The heat capacity of the part AB=0.40J∘C−1 , thermal conductivity of the material of the rod. K=200Wm−1C−1 . Neglect any loss of heat to the atmosphere.
The correct Answer is:A, B, C
The rate of heat flow per sec
dQAdt=KAd(θA)dl
The rate of heat flow per sec
dQBdt=KAd(θB)dl
the part of heat is absorbed by the rod.Qt=msΔθdt
where dθdt= Rate of tempareture variation .
⇒msΔθdt=KAd(θA)dl−KAd(θB)dl,
⇒msdθdt=KA(d(θA)dl−d(θB)dl)
⇒0.4dθdt=200×2.5×10−4
(5−2.5)∘C/cm.
⇒dθdt=200×2.5×10−40.4×10−2
=1250×10−2=12.50Cs.
Topper's Solved these Questions
- HEAT TRANSFERBOOK - HC VERMACHAPTER - HEAT TRANSFEREXERCISE - EXERCIESE
1 Video
- HEAT TRANSFERBOOK - HC VERMACHAPTER - HEAT TRANSFEREXERCISE - Objective 2
6 Videos
- HEAT AND TEMPERATUREBOOK - HC VERMACHAPTER - HEAT AND TEMPERATUREEXERCISE - Exercise
4 Videos
- INTRODUCTION TO PHYSICSBOOK - HC VERMACHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Exercises
19 Videos
Similar Questions
Find the rate of heat flow through a cross section of the rod shown in figure (θ2>θ1 . Thermal conductivity of the material of the rod is K.

View SolutionA rod of length1m having cross sections area 0.75m^(2), conducts heat at 6000J/sec.Then the temperature difference across the rod isK=200Wm^(-1)k^(-1)
View Solution
Knowledge Check
A metal rod of area of cross section A has length L and coefficient of thermal conductivity K the thermal resistance of the rod is .
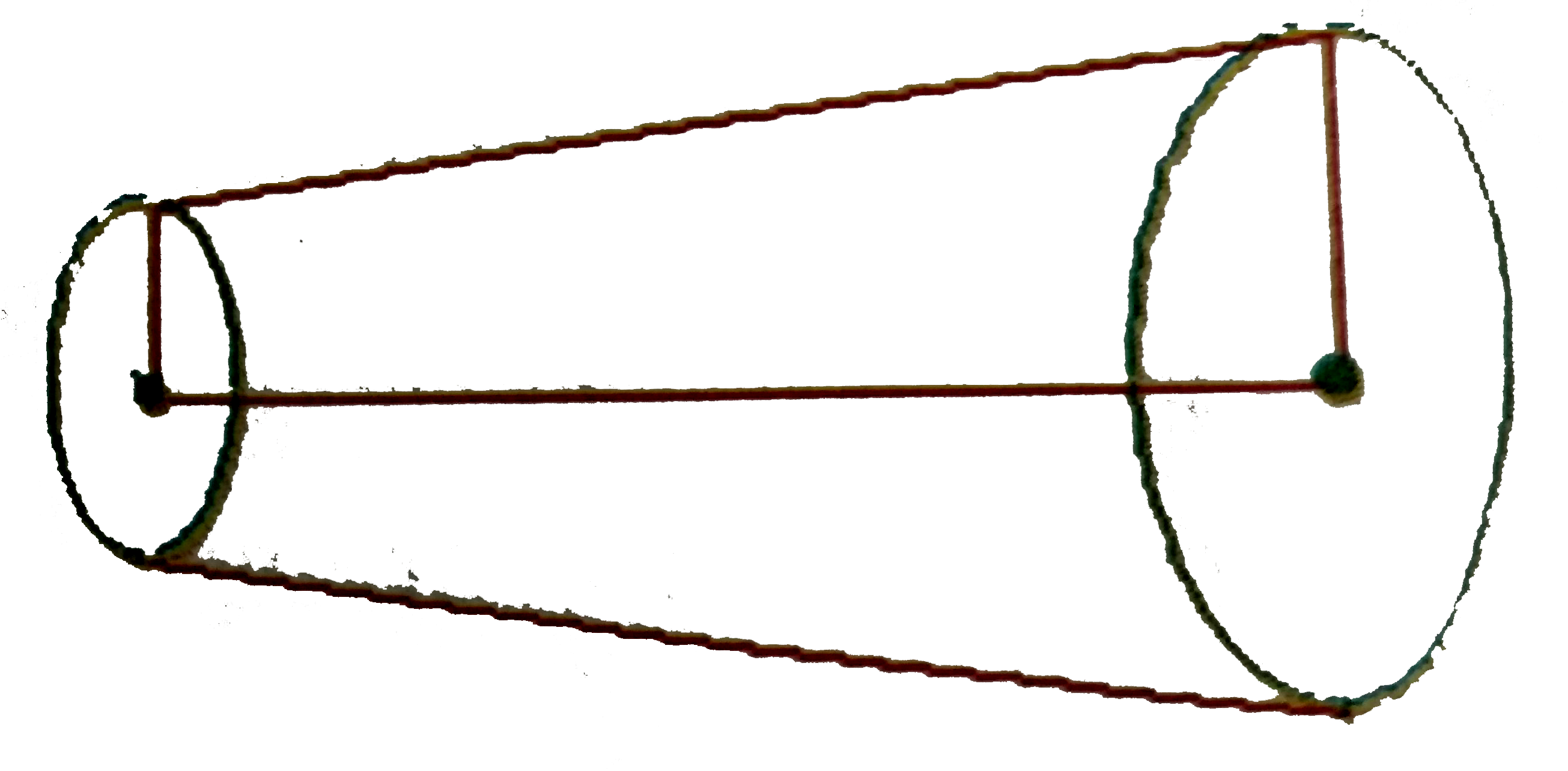
ALKABKLLCKALDAKLAn irregular rod of same uniform material as shown in is conducting heat at a steady rate. The temperature gradient at varoius sections versus area of cross section graph will be
 .A
.A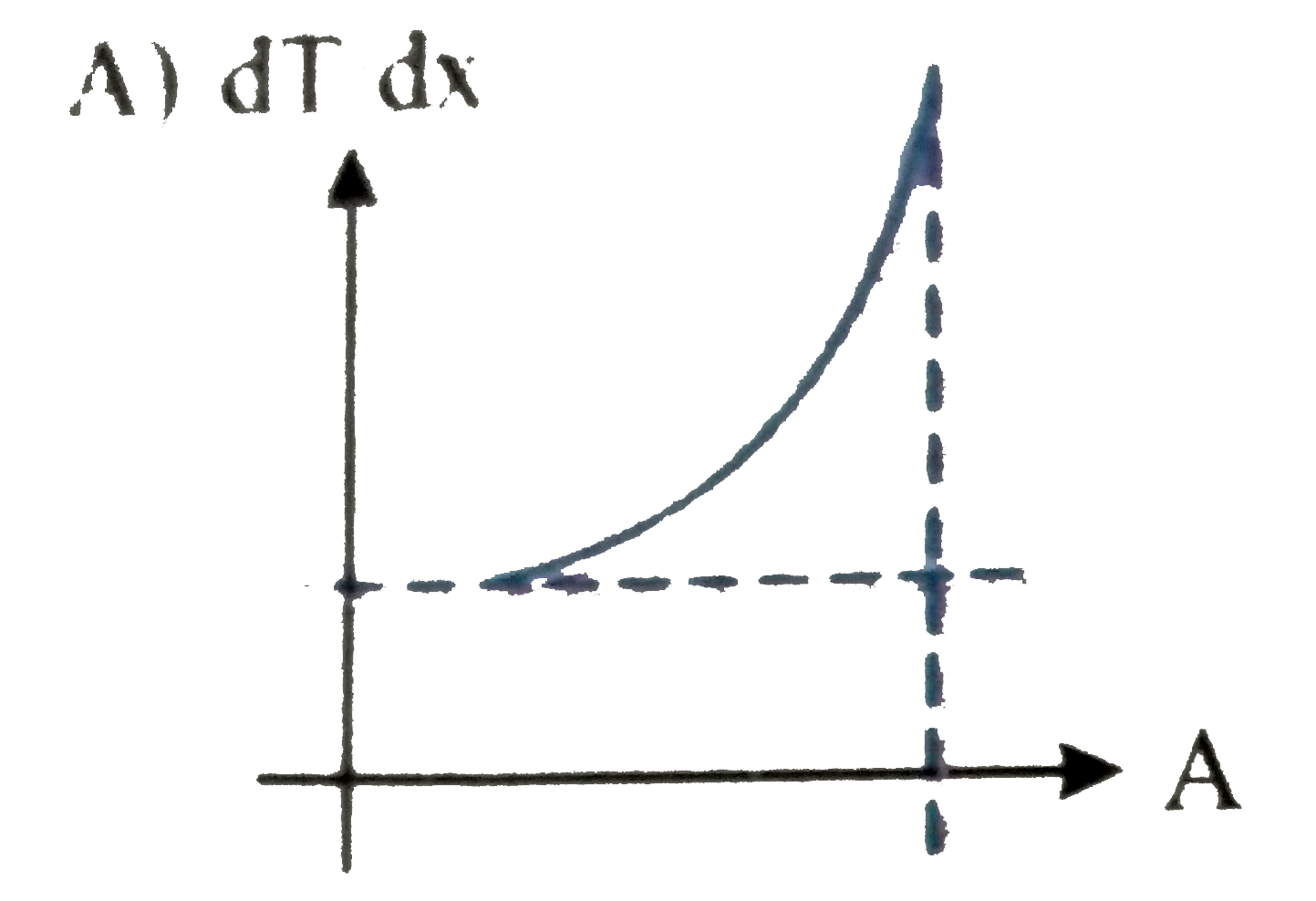 B
B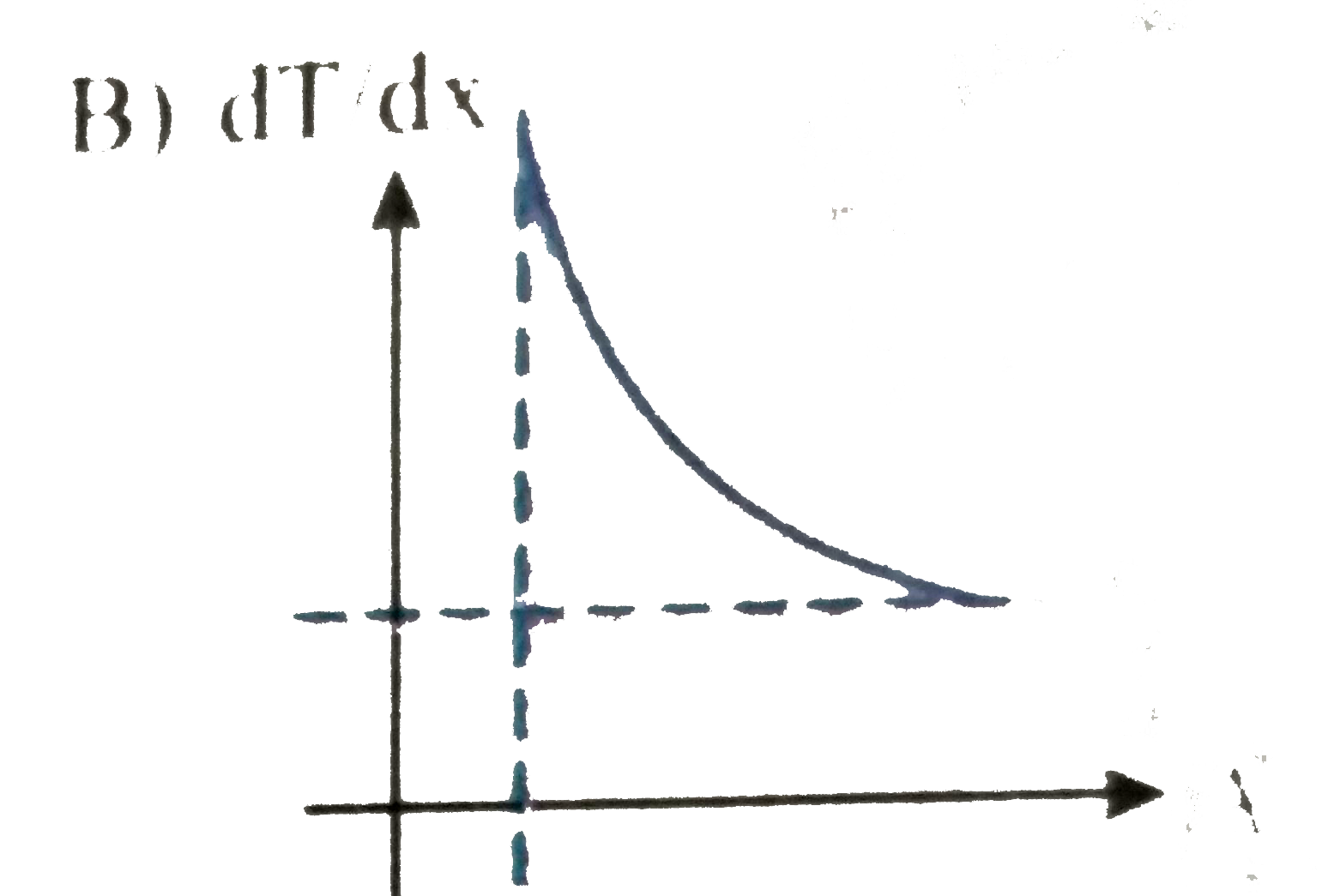 C
C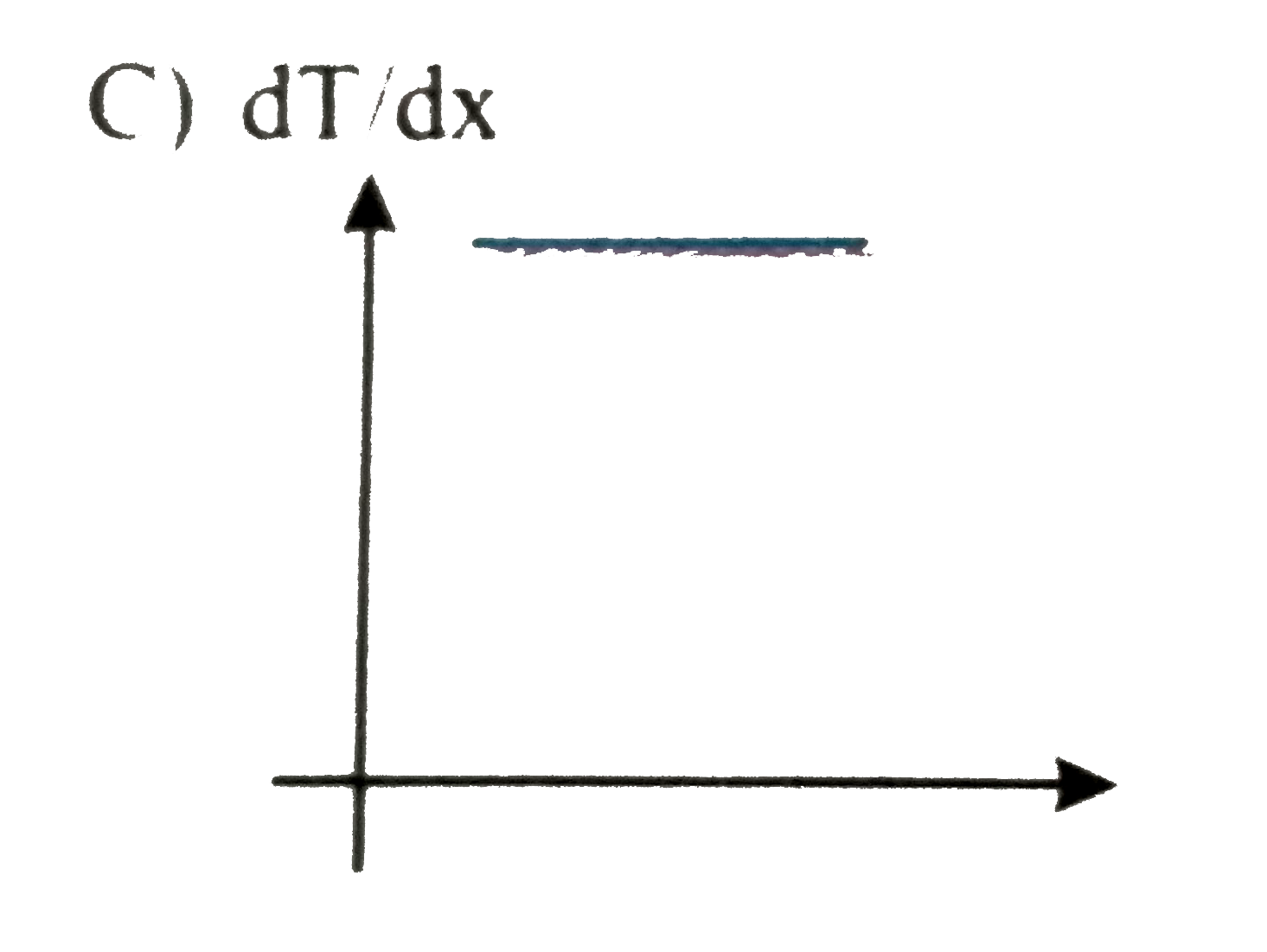 D
D
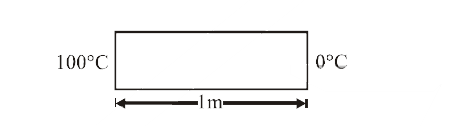
A rod of 1m length and area of cross-section 1cm2 is connected across two heat reservoirs at temperature 100∘C and 0∘C as shown. The heat flow per second through the rod in steady state will be [Thermal conductivity of material of rod =0.09kilocalm−1s−1('∘C)]
 A9×10−4kilocal/secB9kilocal/secC0.09kilocal/secD9×10−6kilocal/sec
A9×10−4kilocal/secB9kilocal/secC0.09kilocal/secD9×10−6kilocal/sec
A rod of 1 m length and area of cross-section 1cm2 is connected across two heat reservoirs at temperatures 100∘Cand0∘C as shown. The heat flow per second through the rod in steady state will be [Thermal conductivity of material of rod = 0.09 kilocal m−1s−1(∘C)]
View SolutionFind the thermal resistance of an aluminium rod of length 20cm and area of cross section 1cm2 . The heat current is along the length of the rod. Thermal conductivity of aluminium =200Wm−1K−1 .
View SolutionOne end of a metal rod of length 1.0 m and area of cross section 100cm2 is maintained at . 100∘C. If the other end of the rod is maintained at 0∘C , the quantity of heat transmitted through the rod per minute is (Coefficient of thermal conductivity of material of rod =100W/m−K)
View SolutionAn irregular rod of same uniform material as shown in figure is conducting heat at a steady rate. The temperature gradient at various sections versus area of cross section graph will be
_S01_094_Q01.png)
View Solutionmeter rod of area of cross section 4mc2 with K=0.5calg−1C−1 is observed that at steady state 360 cal of heat flows per minute The temperature gradient along the rod is .
View Solution