Points X and Y are on sides BC and CD of square ABCD,as shown in the figure. The lengths of XY, AX and AY are 3,4 and 5 respectively.Then the side length of the square ABCD is : a. $\frac{15}{\sqrt{7}}$ b. $\frac{16}{\sqrt{7}}$ c. $\frac{13}{\sqrt{7}}$ d. none of these

Video Solution

Text Solution

Verified by Experts

AD=CD, AD=DY+CY
$5 Cos θ = 5 sin θ + 3 cos (90 + θ - ϕ)$
$5 cos θ = 5 sin θ + 3 cos (θ + 37)$
$5 cos θ = 5 sin θ + 3 (\frac{4}{5} cos θ = \frac{3}{5} sin θ)$
$5 cos θ - \frac{12}{5} cos θ = 5 sin θ = \frac{9}{5} sin θ$
$\frac{13}{5} cos θ = \frac{16}{5} sin θ$
$tan θ = \frac{13}{16}$
$cos θ = \frac{16}{20.6}$
$a = 5 cos θ = \frac{16 \cdot 5}{20.6} = 3.88$ .

Updated on:21/07/2023

|

Class 10 MATHS REAL NUMBERS

Similar Questions

Knowledge Check

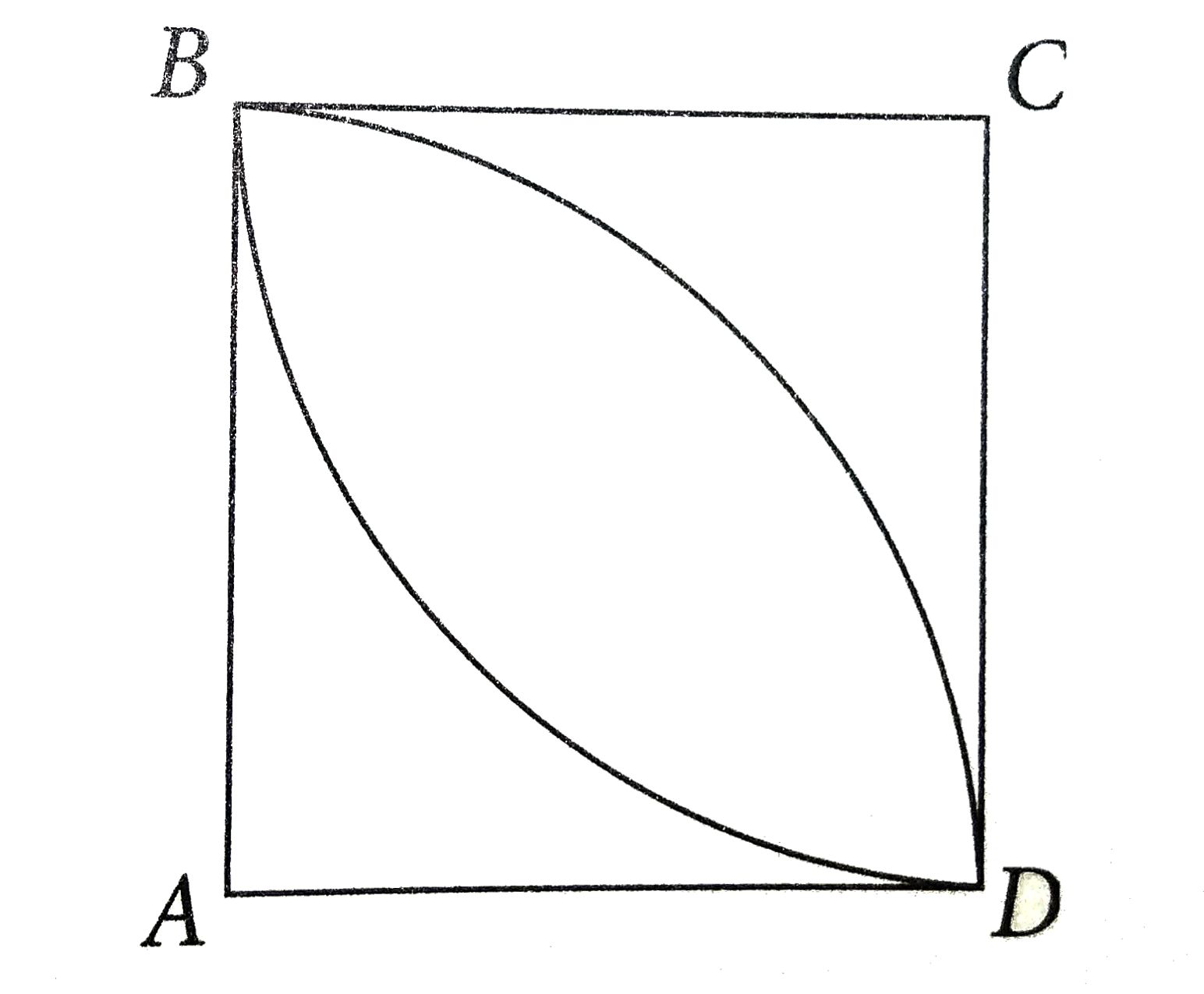
In the figure above, ABCD is a square with sides of 4. What is the length of arc BD?
A $8 π$
B $4 π$
C $2 π$
D $π$

Recommended Questions

Home
Ask Doubt
Profile