Given many parallelograms, prove that a circle can be inscribed only in a rhombus.
Step by step video solution for Given many parallelograms, prove that a circle can be inscribed only in a rhombus. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023समान प्रश्न
If the sides of a parallelogram touch a circle prove that the parallelogram is a rhombus.
View SolutionIf all the side of a parallelogram touch a circle, show that the parallelogram is a rhombus. OR Prove that a parallelogram circumscribing a circle is a rhombus.
View SolutionIf all the side of a parallelogram touch a circle, show that the parallelogram is a rhombus. OR Prove that a parallelogram circumscribing a circle is a rhombus.
View SolutionIf a parallelogram circumscribes a circle then prove that it must be a rhombus.
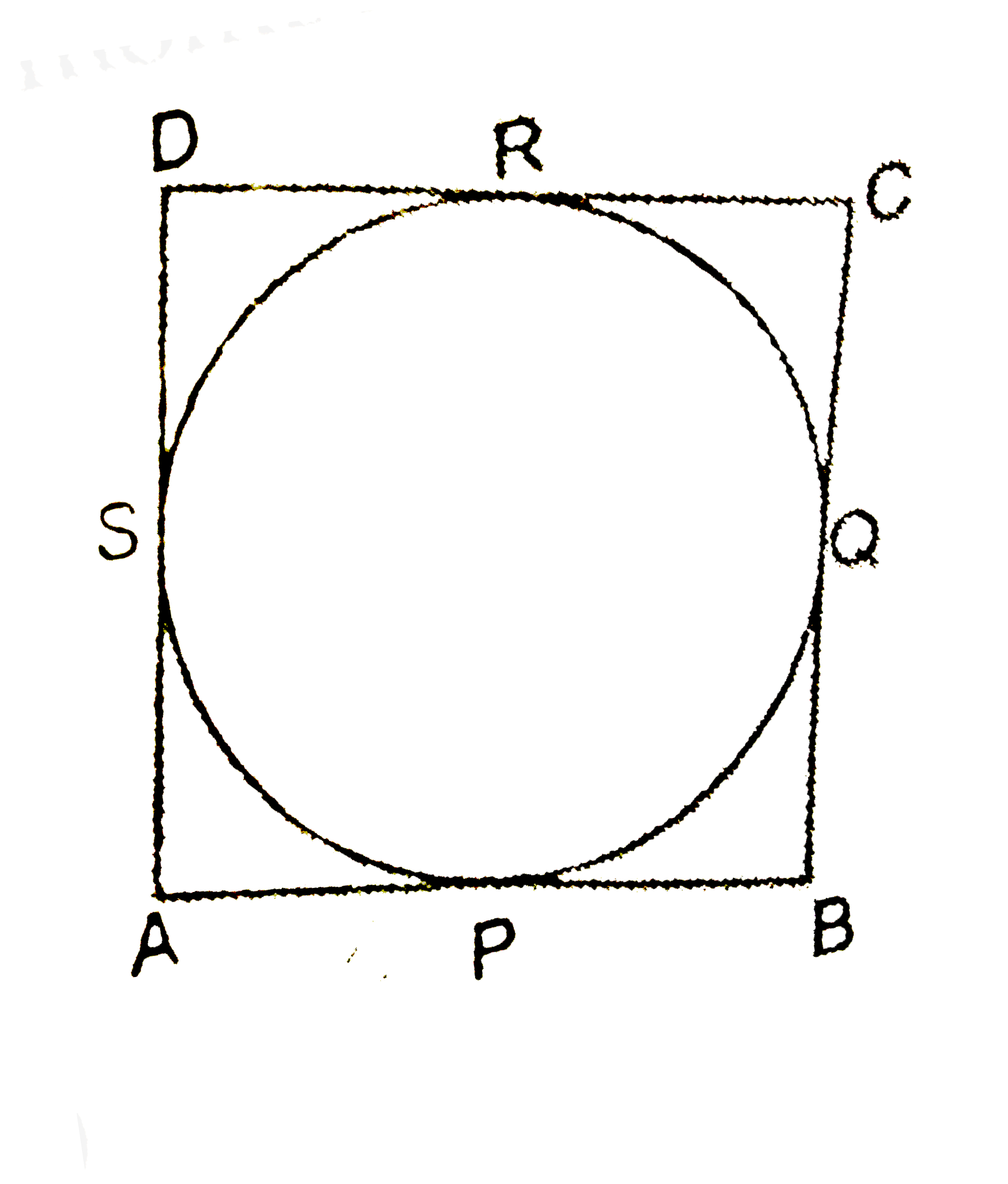
View SolutionIf a parallelogram circumscribes a circle then prove that it must be a rhombus.

View SolutionIn a parallelogram ABCD, prove that it is a rhombus, if diagonals bisect each other at 90∘
View SolutionProve that :
the rhombus , inscribed in a circle is a square.
View SolutionProve that the parallelogram circumscribing a circle is a rhombus.
View SolutionProve that the parallelogram circumscribing a circle is a rhombus.
View SolutionProve that the parallelogram circumscribing a circle is a rhombus.
View Solution
