Question

If the sides of a parallelogram touch a circle prove that the parallelogram is a rhombus.

Explanation

To prove that if the sides of a parallelogram touch a circle, then the parallelogram is a rhombus, we can follow these steps:

Step 1: Understand the configuration
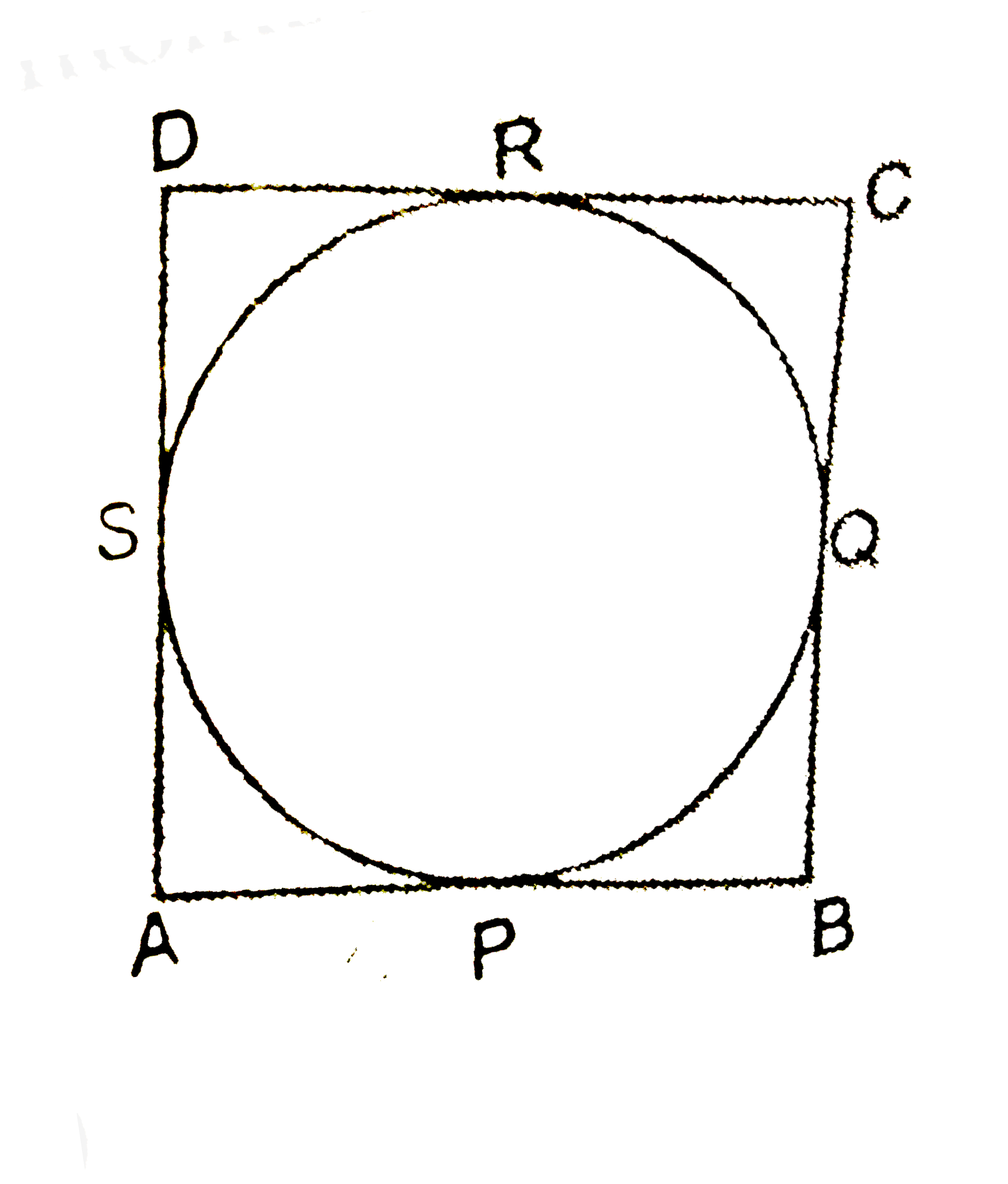
Let ABCD be a parallelogram with a circle inscribed in it, meaning that the sides AB, BC, CD, and DA are tangent to the circle at points P, Q, R, and S respectively.

Step 2: Use the property of tangents
From point A, the segments AP and AS are tangents to the circle. By the property of tangents from a point to a circle, we know that the lengths of tangents drawn from a point outside the circle to the points of tangency are equal. Therefore, we can write:
- AP = AS (1)

Step 3: Apply the same property for other points
Similarly, we can apply the same property to the other vertices of the parallelogram:
- From point B: BP = BQ (2)
- From point C: CR = CQ (3)
- From point D: DR = DS (4)

Step 4: Add the equations
Now, we can add all four equations together:
AP + BP + CR + DR = AS + BQ + CQ + DS

Step 5: Substitute the sides of the parallelogram
Since AB = AP + BP, CD = CR + DR, AD = AS + DS, and BC = BQ + CQ, we can rewrite the equation as:
AB + CD = AS + BQ + CQ + DS

Step 6: Rearranging the equation
We can rearrange the equation to show that:
AB + CD = AD + BC

Step 7: Use properties of parallelograms
In a parallelogram, opposite sides are equal. Therefore, we have:
AB = CD and AD = BC.

Step 8: Conclude the proof
From the equations derived, we can conclude:
AB = BC = CD = DA.
This means all sides of the parallelogram are equal, which is the definition of a rhombus.

Final Conclusion
Thus, we have proved that if the sides of a parallelogram touch a circle, then the parallelogram is a rhombus.

---