Find the coordinates of the point which divides the line segment joining the points (1, 5, 3) and
(-2, 3, 4) in the ration 3:4 (i) internally, and (ii) externally.
(i) Let R(x, y, z) be the required point which divides line segment joining A(1, 5, 3) and B(-2, 3, 4) internally in the ratio 3:4.
∴x=3×(−2)+4×13+4=−27
y=3×3+4×53+4=297
z=3×4+4×53+4=247
Thus, the required point is (−27,297,247).
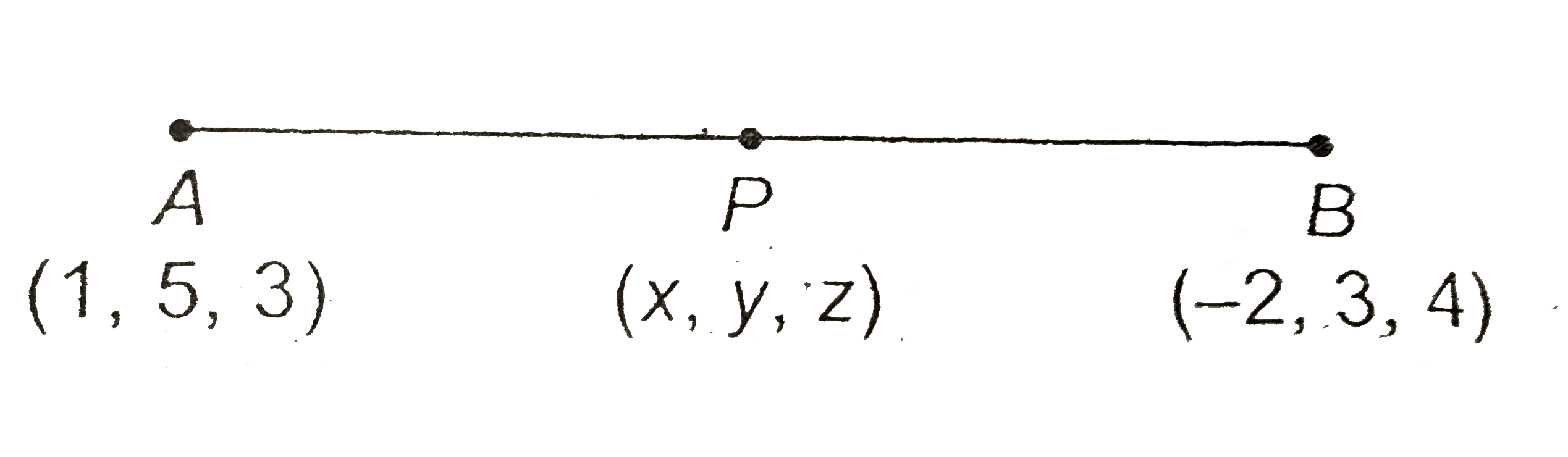
(ii) Let P(x, y, z) be the required point which divides the line segment joining A(1, 5, 3) and
B(-2, 3, 4) externally in the ratio 3:4 then
∴x=3×(−2)−4×13−4=10
y= (3xx3-4xx1)/(3-4) =11<br>x=3×4−4×13−4=0
∴ The required point is (10, 11, 0).
Topper's Solved these Questions
- THREE DIMENSIONAL GEOMETRY BOOK - AAKASH INSTITUTECHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - Illustration
4 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - AAKASH INSTITUTECHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - TRY YOURSELF
97 Videos
- STRAIGHT LINESBOOK - AAKASH INSTITUTECHAPTER - STRAIGHT LINESEXERCISE - SECTION-J (AAKASH CHALLENGERS QUESTIONS)
5 Videos
- TRIGNOMETRIC FUNCTIONS BOOK - AAKASH INSTITUTECHAPTER - TRIGNOMETRIC FUNCTIONS EXERCISE - Section - J (Akash Challengers Question)
15 Videos
Similar Questions
Find the coordinates of the point which divies the line segment joining the points (1, 3, 5) and
(-2, 1, 0) in the ratio 1 : 2 internally.
View SolutionFind the coordinates of the point which divides the line segment joining the points (1,−2,3) and (3,4,−5) in the ratio 2:3 (1) internally, and (2)externally
View Solution
Knowledge Check
Find the coordinates of the point which divides the line segment joining the points A(5,−2) and B(9,6) in the ratio 3:1
A(8,4)B(2,4)C(7,−4)D(7,5)
Find the coordinates of the point which divides the line segment joining the points A(4, -3) and B(9, 7) in the ratio: 3:2.
View SolutionFind the coordinates of the point which divides the line segments joining the points (6,3) and (−4,5) in the ratio 3:2 (i) internally and (ii) externally.
View SolutionFind the coordinates of the point which divides the line segment joining the points (4, 3) and (8, 5)in the ratio 3 : 1 internally.
View SolutionFind the coordinates of the point which divides the line segment joining the points (4, -3) and (8,5) in the ratio 3:1 internally.
View SolutionFind the coordinates of the points which divides the line segment joining the points (6, 3) and (−4, 5) in the ratio 3 :2 internally.
View SolutionFind the co-ordinates of the point which divides the line segment joining the points (2,3,-4) and (4,-1,2) in the ratio (i) 2 : 3 internally, (ii) 4 : 3 externally.
View SolutionFind the co-ordinates of the point which divides the line segment joining the points (4,-3) and (8,5) in the ratio 1:3 internally
View Solution