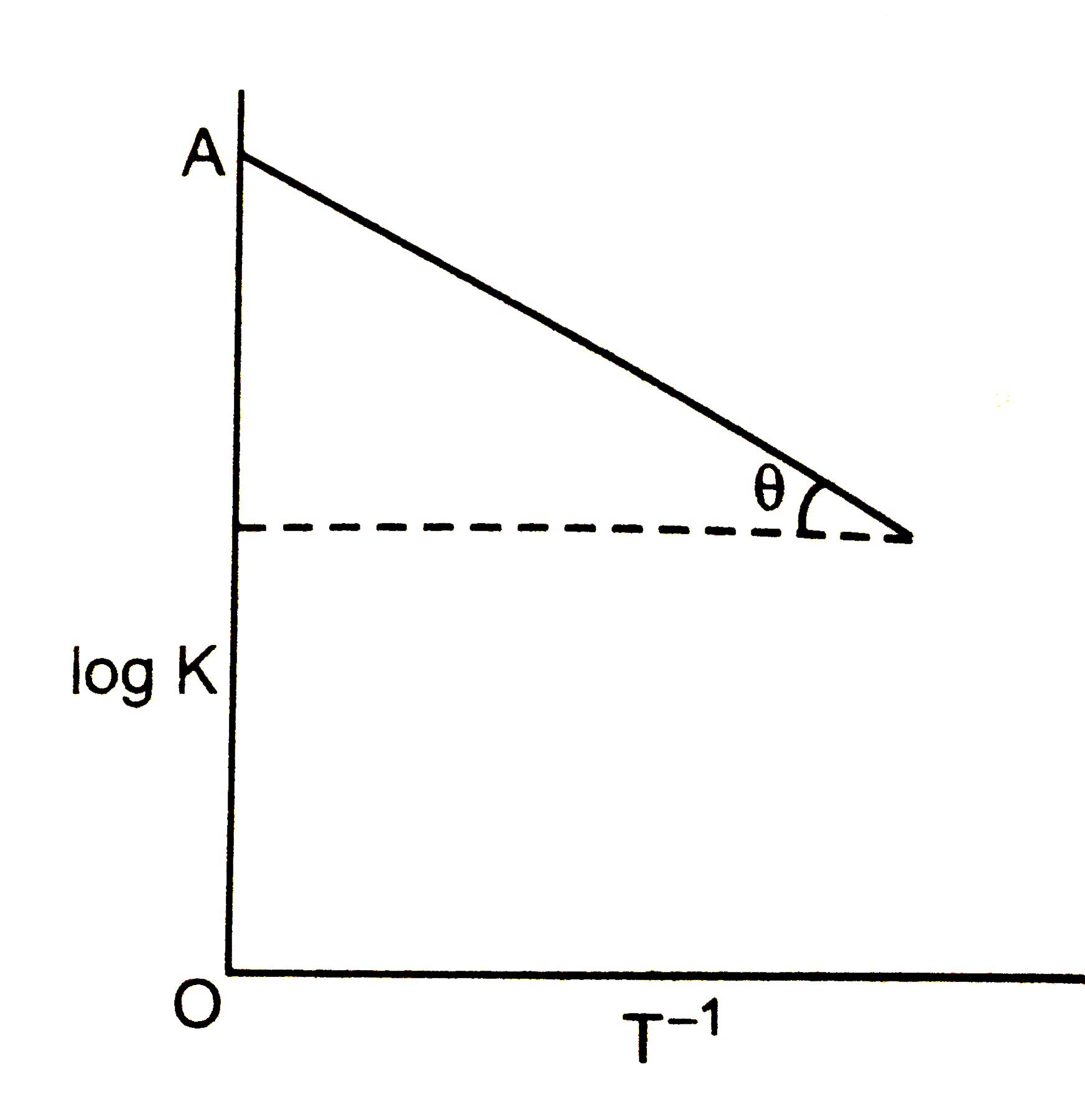
For a hypothetical reactionP(g) + Q(g) hArr R(g) + S(g) , " a graph between log K and " T^(-1) " is a straight line as hsown in the fig. in which " theta = tan^(-1) 0*5 and OA = 10. " Assuming "Delta H^(@) " is independent of temperature , calculate the equilibrium constant of the reaction at 298 K and 798 K respectively.
Effect of temperature on equilibrium constant K is given by van't Hoff equation , viz.
logK=−ΔH∘2⋅303RT+C where C = constant of integration
Thus , a plot of log or 1T, i.e., T−1 is a straight line with slope = ΔH∘2⋅303R and intercept = C
∴C=10and slope =tanθ=0⋅5=ΔH∘2⋅303×8⋅314orΔH∘=9⋅574 J mol−1
∴logK=10−9⋅5742⋅303×8⋅314×298=9⋅9827
or K= Antlog (9⋅9827)=9⋅96×109
As ΔH∘ is independent of temperature , K will also be independent of temperature . Hence , K wil be same at 298 K and 798 K.
Topper's Solved these Questions
- EQUILIBRIUM IN PHYSICAL AND CHEMICAL PROCESSESBOOK - PRADEEPCHAPTER - EQUILIBRIUM IN PHYSICAL AND CHEMICAL PROCESSESEXERCISE - TEST YOUR GRIP (MULTIPLE CHOICE QUESTIONS)
15 Videos
- EQUILIBRIUM IN PHYSICAL AND CHEMICAL PROCESSESBOOK - PRADEEPCHAPTER - EQUILIBRIUM IN PHYSICAL AND CHEMICAL PROCESSESEXERCISE - TEST YOUR GRIP (II. FILL IN THE BLANKS)
16 Videos
- EQUILIBRIUM IN PHYSICAL AND CHEMICAL PROCESSESBOOK - PRADEEPCHAPTER - EQUILIBRIUM IN PHYSICAL AND CHEMICAL PROCESSESEXERCISE - CURIOSITY QUESTIONS
4 Videos
- EQUILIBRIUMBOOK - PRADEEPCHAPTER - EQUILIBRIUMEXERCISE - Competition Focus (VIII. Assertion-Reason Type Questions)
16 Videos
- HYDROCARBONSBOOK - PRADEEPCHAPTER - HYDROCARBONSEXERCISE - Competition Focus (JEE(main and advanced)/Medical Entrance) VIII. ASSERTION - REASON TYPE QUESTIONS
31 Videos
Similar Questions
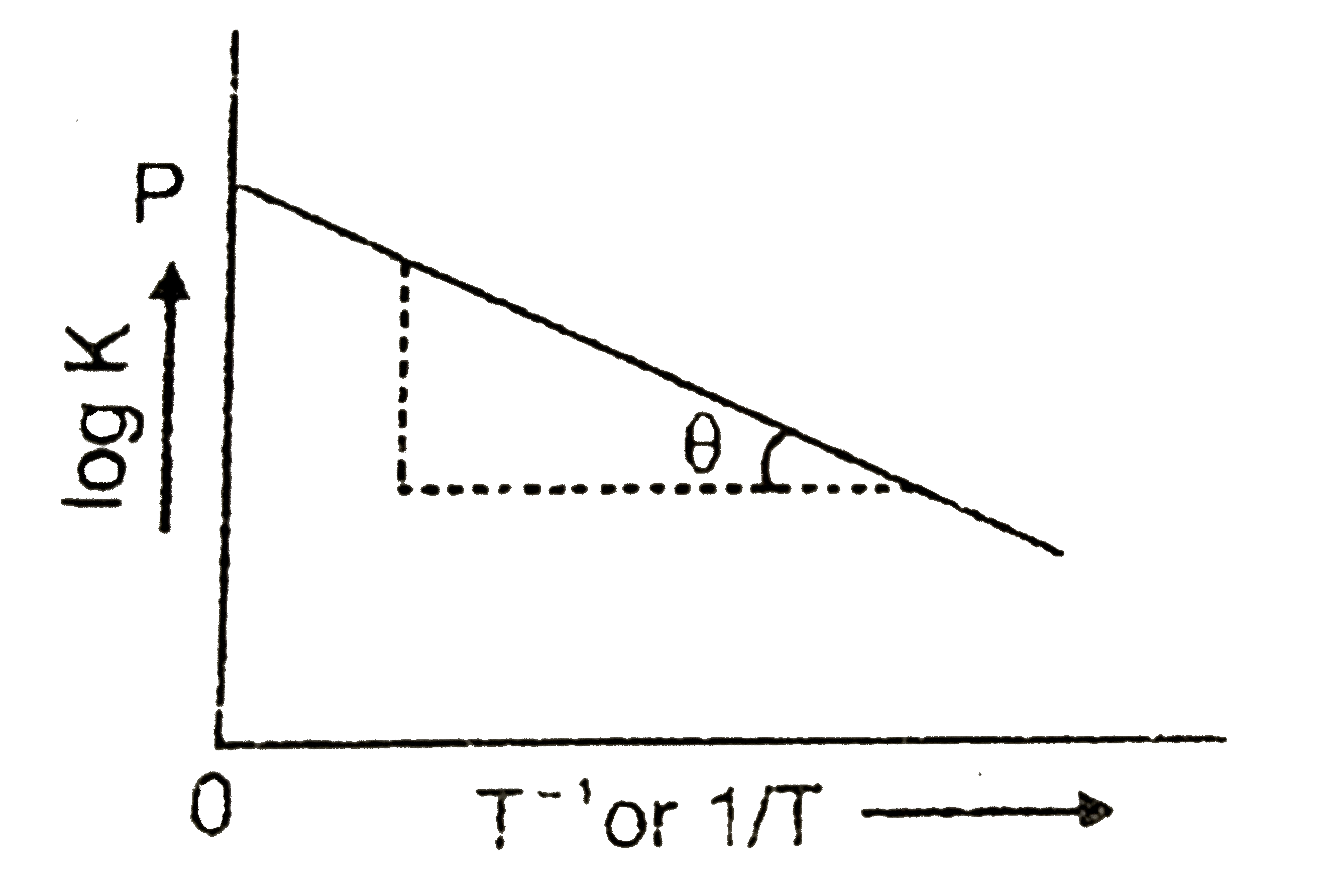
Variation of equiibrium constant K with temperature T is given by van 't Holf equation,
logK=logA−ΔH∘2.303RT
A graph between log K and T−1 was a straight line as shown in the figure and having θ=tan−1(0.5)andOP=10. Calculate:
(a) ΔH∘ (standard heat of reaction)when T=300K,
(b) A (pre-exponetial factor),
(c) Equilibrium constant K at 300K,
(d) Kat900KifΔH∘ is independent of temperature.
View SolutionUnit of equilibrium constant Kp for the reaction PCl5(g)⇔PCl3(g)+Cl2(g) is
View Solution
Knowledge Check
For a hypothetical reaction: A(g)+B(g)⇔C(g)+D(g) a graph between log Keq and T− is striaght line as follows, where θ=tan−10.5 and OA=1.0 (S.I. units) Assuming ΔH∘ is independent of temperature, the equilibrium constant at 298 K will be:
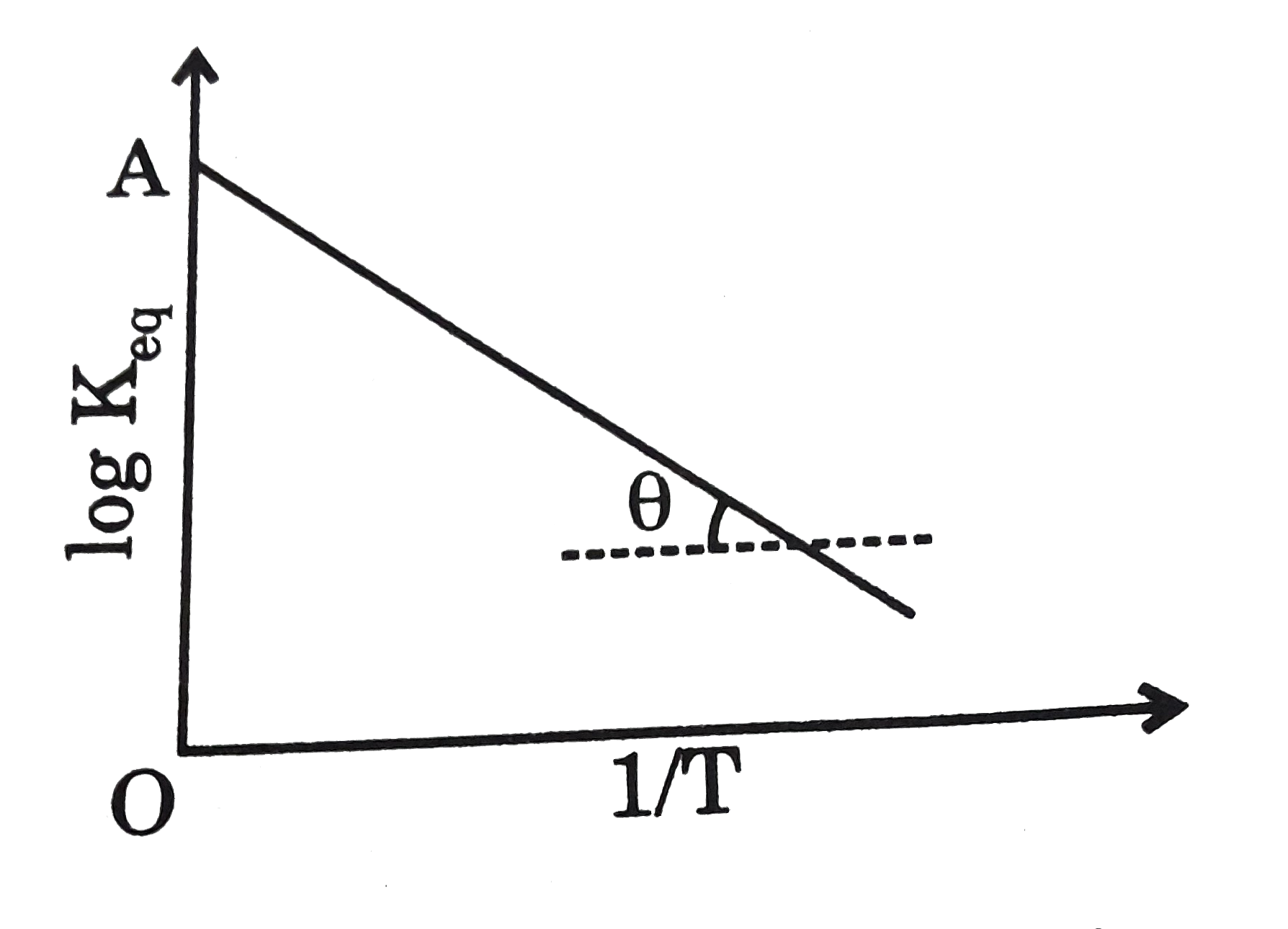 A10.002×109B3.79×108C7.96×109D9.96×109
A10.002×109B3.79×108C7.96×109D9.96×109The equilibrium constant Kp for the reaction H2(g)+I2(g)⇔2HI(g) changes if:
Athe total pressure changesBa catalyst is usedCthe amounts of H2andI2 changeDthe temperature changesFor the reaction H2(g)+I2(g)⇔2HI(g), the equilibrium constant Kp Changes with
Atotal pressureBcatalystCthe amount of H2andI2 presentDtemperature
The equilibrium constant Kp for the reaction H2(g)+I2(g)⇔2HI(g) changes if:
View SolutionGraph between logk and 1/T [k rate constant (s−1) and T and the temperature (K)] is a straight line with OX=5,θ=tan−1(1/2.303). Hence −Ea will be

View SolutionΔHΘ and ΔSΘ for the reaction:
Br2(l)+CI2(g)⇔2BrCI(g)
at 298K are 29.3kJmol−1 and 104.1JK−1mol−1, respectively. Calculate the equilibrium constant for the reaction.
View SolutionGraph between logk and 1/T [k rate constant (s−1) and T and the temperature (K)] is a straight line with OX=5,θ=tan−1(1/2.303). Hence −Ea will be

View SolutionGraph between logk and 1/T [k rate constant (s−1) and T and the temperature (K)] is a straight line with OX=5,θ=tan−1(1/2.303). Hence −Ea will be

View Solution
