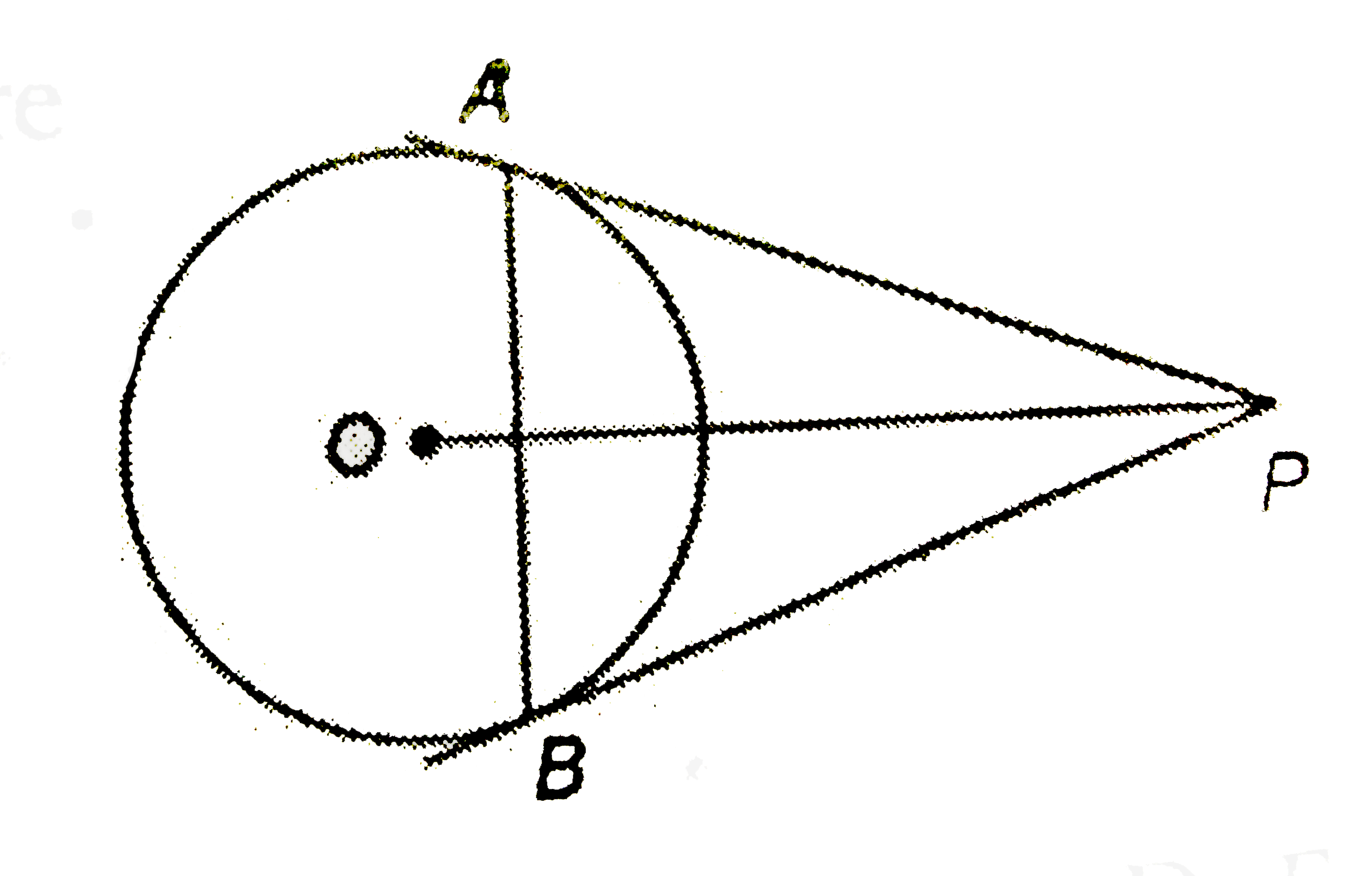
From a point P two tangents are drawn to a circle with center o. if OP = diameter of the circle, show △APB is equilateral.
In △OAP
OP=2r
Sinθ=OAOP=r2r=12
sinθ=300
∠OPA=30o
similarly,
∠OPB=30o
∠APB=∠OPA+∠OPB
=30⊕30o
=60o
In△PAB
∠PAB=∠PBA
∠PAB+∠PBA+∠APB=180o
2∠PAB=120o
∠PAB=60o
so, ∠PBA=60o
and∠APB=60o
so, △APB is an equilateral triangle
সম্পর্কিত প্রশ্ন
জ্ঞান পরীক্ষা করুন
From a point P two tangents PA and PB are drawn to a circle with centre O.if OP is equal to diameter of the circle then ∠APB is
A60∘B45∘C90∘D30∘From a point P, two tangents PA and PB are drawn to a circle with centre O. If OP is equal to diameter of the circle, then ∠APBis
A45∘B90∘C30∘D60∘
From a point P , two tangents PA and PB are drawn to a circle with centre O . If OP= diameter of the circle, show that △APB is equilateral.
View SolutionFrom a point P, two tangents PA and PB are drawn to a circle with centre O and radius r. If OP=2r, show that △APB is equilateral.
View SolutionFrom a point P, two tangents PA and PB are drawn to a circle with centre O and radius r. If OP=2r, show that △APB is equilateral.

View SolutionPA and PB are tangents to a circle with centre O from point P. OP is equal to the diameter of the circle. Prove that ABP is equilateral triangle,
View SolutionFrom a point P, two tangents PT and PS are drawn to a circle with center O.Such that ∠SPT=120∘. Prove that OP=2PS.
View SolutionFrom a point P, two tangents PT and PS are drawn to a circle with centre O such that ∠SPT=120∘ Prove that OP = 2PS.
View Solution