Two radius of a circle are inclined at 130∘. Tangents are drawn at the anti points of the tangents formed from the radii to from a quadrilateral. Expain the special quadrilateral formed.
RD=RA
QC=QD
PC=PB
AS=BS
PCOB is similar to ODRA
RD=PB
RA=PC
QR⊥BD&PS⊥BD
QR∣∣PS
QP∣∣RS
PQ=QR=RS=PS
∠PQS~∠QSR
QSQS=AOOR
PQRS is a rhombus.
Similar Questions
Two radii of a circle are inclined at 130∘. Tangents are drawn at the end points of the diameters formed from the radii to form a quadrilateral. Explain the special quadrilateral formed.
View SolutionA) The radius of a circle is 5 cm. At this point The tangent is drawn if the length of the tangent is 12 cm. Find the distance from the center of the circle to the point.
View Solution
Knowledge Check
....... Tangents can be drawn from a point outside a circle.
AZeroBTwoCOneDInfiniteTangents are drawn to the ellipse x29+y25 at the end of the latus rectum. The area of the quadrilateral so formed is
A27B272C274D2755x2+y2−4x−2y−11=0 is a circle to which tangents are drawn from the point (4, 5) which form a quadrilateral with a pair of radii. The area of this quadrilateral in sq. units is
A2B4C6D8
Two tangents PQ and PR are drawn from an external point to a circle with centre O. Prove that QORP is cyclic quadrilateral.
View SolutionTheorem: The length of two tangents drawn from an external point to a circle are equal.
View Solution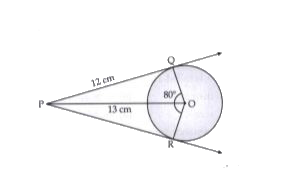
A point P is 13 cm from the centre of the circle. The two tangent PQ and PR are drawn from the point P, The length of the tangent drawn from P to the circle is 12 cm.
Find the radius of the circle.
View SolutionChoose the CORRECT option about the two tangents drawn to the circle from the external point?
1. The tangents subtend equal angles at the centre of the circle.
2. The tangents drawn from the external point to a circle are equal in length.
3. The tangents are equally inclined to the segment which joins the centre to that point.
View SolutionMaximum number of tangents drawn from a point on the circle is :
View Solution
