If (mi,1mi),i=1,2,3,4 are four distinct points on a circle, show that m1m2m3m4=1
To show that m1m2m3m4=1 given that the points (mi,1mi) for i=1,2,3,4 are distinct points on a circle, we can follow these steps:
Step 1: Equation of the Circle
Assume the equation of the circle is given by:
x2+y2+2gx+2fy+c=0
where g,f,c are constants.
Step 2: Substitute the Points
Substituting the point (mi,1mi) into the circle's equation:
(mi)2+(1mi)2+2g(mi)+2f(1mi)+c=0
This simplifies to:
m2i+1m2i+2gmi+2fmi+c=0
Step 3: Clear the Denominator
Multiply through by m2i to eliminate the fraction:
m4i+1+2gm3i+2fmi+cm2i=0
Rearranging gives us:
m4i+2gm3i+cm2i+2fmi+1=0
Step 4: Polynomial Form
This equation can be viewed as a polynomial in mi:
m4i+2gm3i+cm2i+2fmi+1=0
Let P(m)=m4+2gm3+cm2+2fm+1.
Step 5: Roots of the Polynomial
The points (m1,1m1),(m2,1m2),(m3,1m3),(m4,1m4) imply that m1,m2,m3,m4 are the roots of the polynomial P(m)=0.
Step 6: Product of the Roots
From Vieta's formulas, the product of the roots of a polynomial axn+bxn−1+...+k=0 is given by:
(−1)nka
For our polynomial P(m):
- The degree n=4 (since the highest power is m4).
- The constant term k=1.
- The leading coefficient a=1.
Thus, the product of the roots m1m2m3m4 is:
m1m2m3m4=(−1)411=1
Conclusion
Therefore, we conclude that:
m1m2m3m4=1
---
Assume the equation of the circle is given by:
x2+y2+2gx+2fy+c=0
where g,f,c are constants.
Substituting the point (mi,1mi) into the circle's equation:
(mi)2+(1mi)2+2g(mi)+2f(1mi)+c=0
This simplifies to:
m2i+1m2i+2gmi+2fmi+c=0
Multiply through by m2i to eliminate the fraction:
m4i+1+2gm3i+2fmi+cm2i=0
Rearranging gives us:
m4i+2gm3i+cm2i+2fmi+1=0
This equation can be viewed as a polynomial in mi:
m4i+2gm3i+cm2i+2fmi+1=0
Let P(m)=m4+2gm3+cm2+2fm+1.
The points (m1,1m1),(m2,1m2),(m3,1m3),(m4,1m4) imply that m1,m2,m3,m4 are the roots of the polynomial P(m)=0.
From Vieta's formulas, the product of the roots of a polynomial axn+bxn−1+...+k=0 is given by:
(−1)nka
For our polynomial P(m):
- The degree n=4 (since the highest power is m4).
- The constant term k=1.
- The leading coefficient a=1.
m1m2m3m4=(−1)411=1
Therefore, we conclude that:
m1m2m3m4=1
Similar Questions
Knowledge Check
Particles of masses m1 and m2 are at a fixed distance apart. If the gravitational field strength at m1 and m2 are →I1 and →I2 respectively. Then,
Am1→I1+m2→I2=0Bm1→I2+m2→I1=0Cm1→I1−m2→I2=0Dm1→I2−m2→I1=0Four particle of masses m1=2m,m2=4m,m3=mandm4 are placed at four corners of a square. What should be the value of m4 so that the centres of mass of all the four particle are exactly at the centre of the square ?
 A3 mB5 mC8 mDnone of these
A3 mB5 mC8 mDnone of theseIf m1 and m2 are the slopes of tangents to the circle x2+y2=4 from the point (3,2), then m1−m2 is equal to
A512B125C32D0
ABCD is a square of length a, a∈N, a > 1. Let L1,L2,L3... be points on BC such that BL1=L1L2=L2L3=....1 and M1,M2,M3,....be points on CD such that CM1=M1M2=M2M3=...=1. Then a−1∑n=1((ALn)2+(LnMn)2) is equal to :
View SolutionFind the value of m
m−13−m−24=1
View SolutionLet li,mi,ni;i=1,2,3 be the direction cosines of three mutually perpendicular lines referred to an orthogonal cartesian co–ordinate system, then prove that A=⎡⎢⎣l1m1n1l2m2n2l3m3n3⎤⎥⎦is an orthogonal matrix.
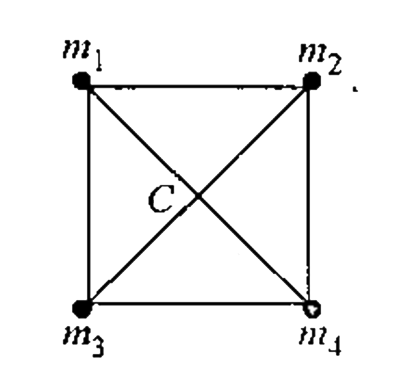
View SolutionFour particle of masses m1=2kg,m2=4kg,m3=1kg and m4 are placed at four corners of a square as shown in figure. Can mass of m4 be adjusted I such a way that the centre of mass of system will be at the centre of the square C.
View SolutionIf m+1m−2=4, find
m2+m+1=?
View Solution
