The area bounded between the parabolas x2=y4andx2=9y and the straight line y=2 is (1) 20√2 (2) 10√23 (3) 20√23 (4) 10√2
Video Solution
|
Answer
Step by step video & image solution for The area bounded between the parabolas x^2=y/4"and"x^2=9y and the straight line y""=""2 is (1) 20sqrt(2) (2) (10sqrt(2))/3 (3) (20sqrt(2))/3 (4) 10sqrt(2) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
The area bounded between the parabola x2=y4 and x2=9y and the straight line y=2 is
A20√2B10√23C20√23D10√2 - Question 2 - Select One
The graph of straight line y=√3x+2√3 is :
A B
B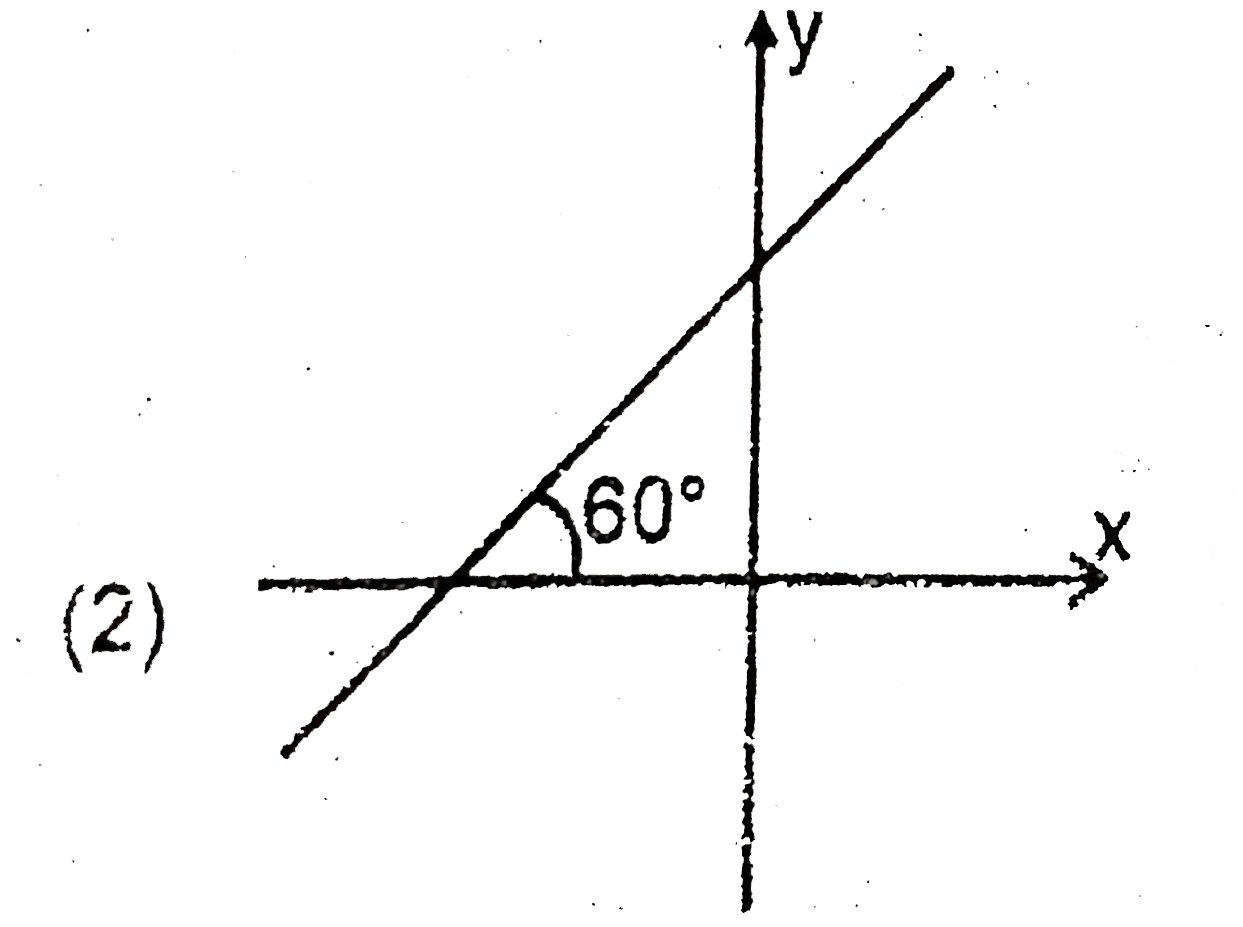 C
C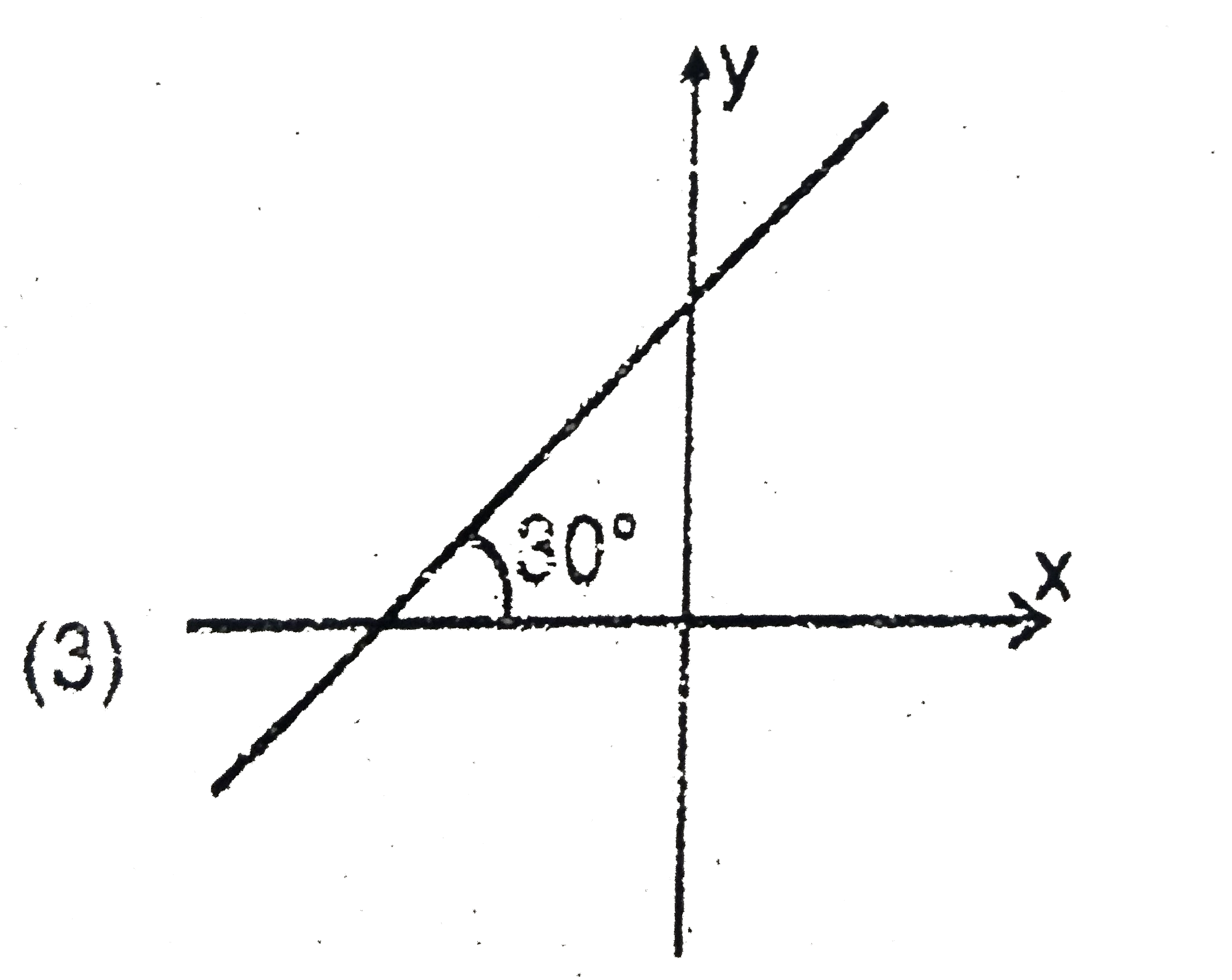 D
D
Area bounded between the curves y=√4−x2 and y2=3|x| in square units is
View SolutionThe area bounded by the curves x2+y2=4, |y|=√3x and y - axis, is
View SolutionThe area included between the parabolas y2=4ax(x+a)andy2=4b(b−x)is83√ab(a+b).True False
View Solution2√x+3√y=2 and 4√x−9√y=−1
View SolutionThe shortest distance between line y-x=1 and curve x=y2 is : (1) √34 (2) 3√28 (3) 83√2 (4) 4√3
View SolutionThe area bounded by the curve x2+y2=36and√|x|+√|y|=√6 is :
View Solution

