Shows a large closed cylindrical tank containing water, Initially the air trapped above the water surface has a height $h_{0}$ and pressure $2 p_{0}$ where $p_{0}$ is the atmospheric pressure. There is a hole in the wall of tank at a depth $h_{1}$ below the top from which water comes out. A long vertical tube is connected as shown. (a) Find the height $h_{2}$ of the water in the long tube above the top initially. (b) Find the speed with which water comes out of hole.(c)Find the height of the water in the long tube above the top when the water stops coming out of the hole.

Video Solution

Text Solution

Verified by Experts

(a) $2 P_{0} = (h_{2} + h_{0}) ρ g$
$[∴ Sin c e l i q u a t s a m e \leq v e l$
have same pressure
$\Rightarrow 2 P_{0} = (h_{2}) ρ g + (h_{0}) ρ g$
$\Rightarrow (h_{2}) ρ g = 2 (P_{0}) - (h_{0}) ρ g$
$\Rightarrow (h_{2}) = (2 \frac{P_{0}}{ρ} g) - ((h_{0}) ρ \frac{g}{ρ} g)$
$= (2 \frac{P - 0}{ρ} g) - (h_{0})$
$(b) K . E . o f t h e w a t e r = p r e s s u r e e \neq r g y o f$
the water at that layer
$\Rightarrow \frac{1}{2} m (V_{2}) = m \times (\frac{p}{ρ})$
$\Rightarrow (V_{2}) = (2 \frac{P}{ρ}) = \frac{2}{ρ} [P - 0 + ρ g (h_{1} - h_{0})]$
$\Rightarrow V = ⎛ ⎜ ⎜ ⎝ \frac{{[\frac{2}{ρ} {(P_{0}) + ρ g (h_{1} - h_{0})}]}^{1}}{2} ⎞ ⎟ ⎟ ⎠$
(c) From question,
$2 (P_{0}) + ρ g (h_{1} - h_{0}) = (P_{0}) + ρ g X$
$\Rightarrow X = (\frac{P_{0}}{ρ} g) + (h_{1} - h_{0}) = h_{2} + h_{1}$
$i . e . X i s h_{1} m e t e r b e l o w t h e ⊤$
$\Rightarrow X i s - h_{1} a b o v e t h e ⊤ .$

Updated on:21/07/2023

Class 11 PHYSICS KINETIC THEORY OF GASES

Topper's Solved these Questions

Knowledge Check

Figure shows a large closed cylindrical tank containing water. Initially, the air trapped above the water surface has a height $h_{0}$ and pressure $2 p_{0}$ where $r h_{0}$ is the atmospheric pressure. There is a hole in the wall of the tank at a depth $h_{1}$ below the top from which water comes out. A long vertical tube is connected as shown.

Find the height $h_{2}$ of the water in the long tube above top initially.
A $\frac{3 p_{0}}{ρ g} - \frac{h_{0}}{3}$
B $\frac{2 p_{0}}{ρ g} - \frac{h_{0}}{2}$
C $\frac{p_{0}}{ρ g} - h_{0}$
D $\frac{p_{0}}{2 ρ g} - 2 h_{0}$
Figure shows a large closed cylindrical tank containing water. Initially, the air trapped above the water surface has a height $h_{0}$ and pressure $2 p_{0}$ where $r h_{0}$ is the atmospheric pressure. There is a hole in the wall of the tank at a depth $h_{1}$ below the top from which water comes out. A long vertical tube is connected as shown.

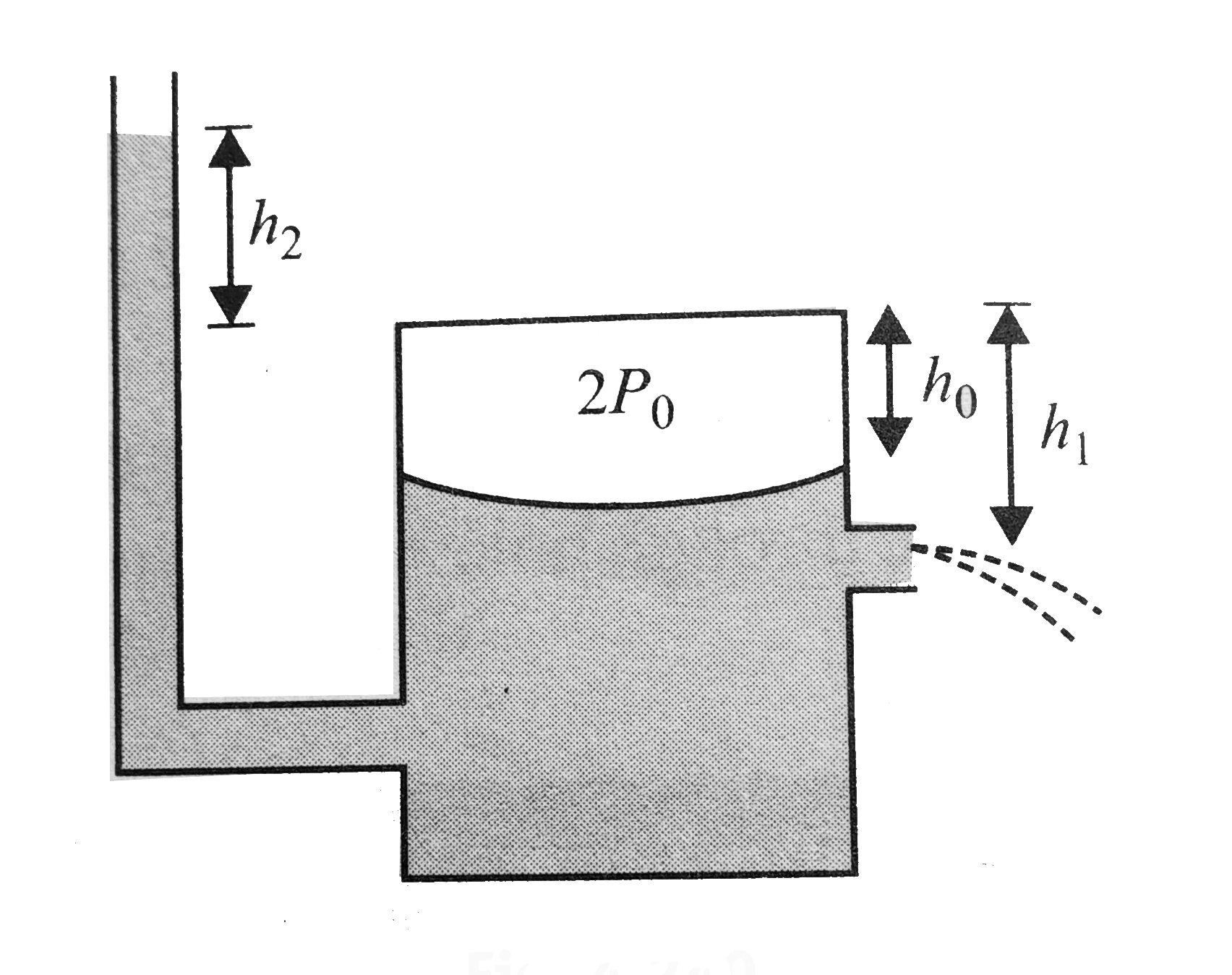
Find the speed with which water comes out of the hole
A $\frac{1}{ρ} {[p_{0} - ρ g (h_{1} - 2 h_{0})]}_{0}^{\frac{1}{2}}$
B ${[\frac{2}{ρ} [p_{0} + ρ g (h_{1} - h_{0})]]}^{\frac{1}{2}}$
C ${[\frac{3}{ρ} [p_{0} + ρ g (h_{1} + h_{0})]]}^{\frac{1}{2}}$
D ${[\frac{4}{ρ} [p_{0} - ρ g (h_{1} - h_{2})]]}^{\frac{1}{2}}$
Figure shows a large closed cylindrical tank containing water. Initially, the air trapped above the water surface has a height $h_{0}$ and pressure $2 p_{0}$ where $r h_{0}$ is the atmospheric pressure. There is a hole in the wall of the tank at a depth $h_{1}$ below the top from which water comes out. A long vertical tube is connected as shown.

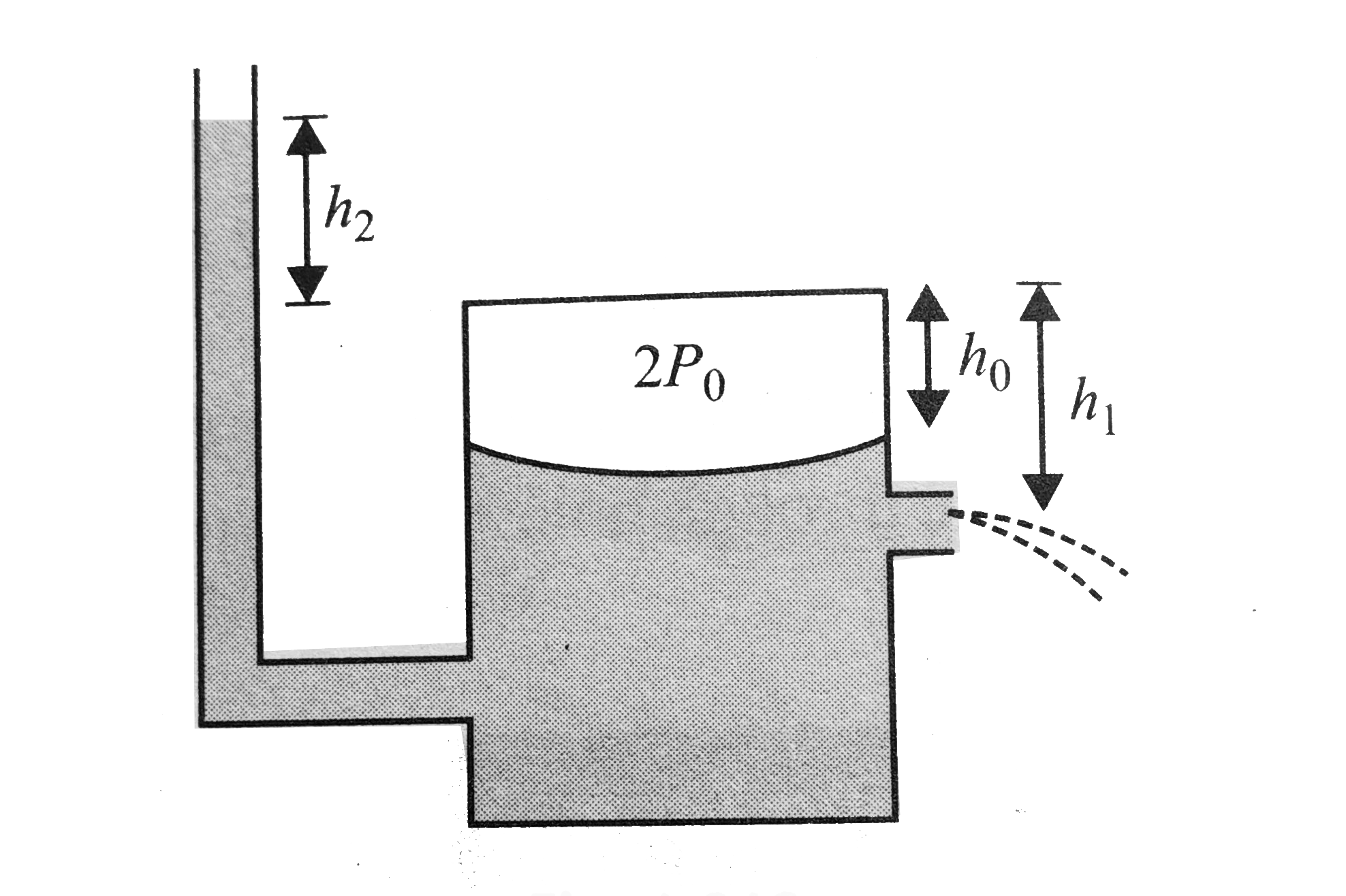
Find the height of the water in the long tube above the top when the water stops coming out of the hole.
A $- 2 h_{0}$
B $h_{0}$
C $h_{2}$
D $- h_{1}$

HC VERMA-KINETIC THEORY OF GASES-Exercises

Home
Ask Doubt
Profile

Topper's Solved these Questions

Similar Questions

Knowledge Check

HC VERMA-KINETIC THEORY OF GASES-Exercises