Find k,P(X<1),P(0<x<3).
Step by step video & image solution for Find k, P (X lt 1), P (0 lt x lt 3). by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If E and F are independent events such that 0<P(E)<1and0<P(F)<1, then
AE and F are mutually exclusiveBE and FC (complement of the event F) are independentCECandFC are independentDP(E/F)+P(EC/F)=1If E and F are independent events such that 0<P(E)<1and0<P(F)<1, then
AE and F are mutually exclusiveBE and FC (complement of the event F) are independentCECandFC are independentDP(E/F)+P(EC/F)=1
A random variable X has the following probability distribution:
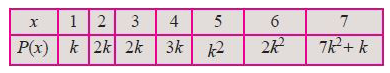
Determine (i)k,(ii)P(X<3),(iii)P(0<X<3),(iv)P(X>4).
View SolutionThe p.d.f of continuous random variable X is given by f(x)=x8,0<x<4=0 otherwise. Find (i) P(X<2)(ii)P(2<X≤3)(iii)P(X>3.)
View SolutionA random variable X has the following probability distribution :
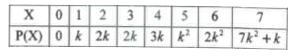
Determine
(i) k (ii) P(X<3)
(iii) P(X>6) (iv) P(0<X<3).
View SolutionFor the following probability density function (p.d.f) of X find : (i) P (X<1),(ii)p(|X|<1) if f(x)=x218'−3<x<3, f(x) = 0 , otherwise
View SolutionThe following is a probability distribution function of a random variable :
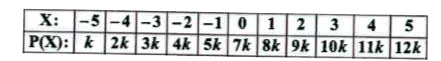
(i) Find k (ii) Find P(X>3) (iii) Find P(−3<X<4)
(iv) Find P(X<−3).
View SolutionIf f(x)= kx, 0 lt x lt 2 = 0 otherwise, is a probability density function of a random variable X , then find (i) value of k, (ii) P(1 lt x lt2).
View Solution
