The velocity of a particle is zero at t=0
The accelerationat t=0 must be zero
the acceleration at t=0 maybet zero
If the acceleratin is zero from t=0 to t=10 s, the speed is also zero in this interval.
If the speed is zer4o from t=0 to t=10 s the acceleration is zero in this interval.
The correct Answer is:B, C, D
To solve the question regarding the velocity of a particle being zero at t=0, we need to analyze the implications of this statement on the acceleration and speed of the particle over time. Here’s a step-by-step breakdown of the reasoning:
Step 1: Understand the Initial Condition
Given that the velocity of the particle at t=0 is zero, we can denote this as:
v(0)=0oru=0
where u is the initial velocity.
Step 2: Analyze the Acceleration
The question presents various statements about acceleration at t=0. We need to determine if the acceleration must be zero, may be zero, or if it affects the speed over time.
1. Acceleration at t=0 must be zero: This statement is incorrect. Just because the velocity is zero at t=0 does not imply that the acceleration must also be zero. A particle can have an initial velocity of zero and still experience acceleration (for example, if it starts from rest and begins to accelerate).
2. Acceleration at t=0 may be zero: This statement is correct. The acceleration can indeed be zero, but it is not a necessity. The particle could be at rest or could start accelerating immediately.
Step 3: Consider the Speed Over Time
Next, we need to analyze the implications of acceleration on speed over a time interval.
1. If acceleration is zero from t=0 to t=10 seconds: If the acceleration remains zero for this interval, then the speed will not change. Since the initial speed is zero and there is no acceleration to change it, the speed will remain zero throughout this interval. Thus, the speed at t=10 seconds is also zero.
2. If speed is zero from t=0 to t=10 seconds: This statement is also correct. If the speed is zero at t=0 and there is no acceleration to change it, then the speed will remain zero at t=10 seconds.
Conclusion
Based on the analysis:
- The initial velocity being zero does not imply that the acceleration must be zero.
- The acceleration may be zero.
- If acceleration is zero, the speed remains zero throughout the interval.
Summary of Correct Statements
- Acceleration at t=0 must be zero: False
- Acceleration at t=0 may be zero: True
- If acceleration is zero from t=0 to t=10 seconds, the speed is also zero: True
- If speed is zero from t=0 to t=10 seconds, the speed remains zero: True
Given that the velocity of the particle at t=0 is zero, we can denote this as:
v(0)=0oru=0
where u is the initial velocity.
The question presents various statements about acceleration at t=0. We need to determine if the acceleration must be zero, may be zero, or if it affects the speed over time.
Next, we need to analyze the implications of acceleration on speed over a time interval.
Based on the analysis:
- The initial velocity being zero does not imply that the acceleration must be zero.
- The acceleration may be zero.
- If acceleration is zero, the speed remains zero throughout the interval.
- Acceleration at t=0 must be zero: False
- Acceleration at t=0 may be zero: True
- If acceleration is zero from t=0 to t=10 seconds, the speed is also zero: True
- If speed is zero from t=0 to t=10 seconds, the speed remains zero: True
Topper's Solved these Questions
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises
51 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Objective 1
13 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMACHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Exercises
34 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
Similar Questions
Knowledge Check
The velocity of a particle is zero at time t = 2s, then
Adisplacement must be zero in the interval t = 0 to t = 2 s.Bacceleration may be zero at t = 2 s.Cvelocity must be zero for t>2s .Dacceleration must be zero at t = 2 s.Statement-I : When velocity of a particle is zero then acceleration of particle is zero.
Statement-II : Acceleration is equal to rate of change of velocity.AStatement-I is true, Statement-II is true, Statement-II is correct explanation for Statement-IBStatement-I is true, Statement-II is true, Statement-II is NOT a correct explanation for Statement-ICStatement-I is true, Statement-II is falseDStatement-I is false, Statement-II is true.The acceleratin of a particle increases linearly with time t as 6t. If the initial velocity of the particles is zero and the particle starts from the origin, then the distance traveled by the particle in time t will be
AtBt2Ct3Dt4
If the instantaneous velocity of a particle is zero, will its instantaneous acceleration be necessarily zero
View SolutionThe velocity time relation of a particle is given by v =(3t2−2t−1)m/s Calculate the position and acceleration of the particle when velocity of the particle is zero . Given the initial position of the particle is 5m .
View SolutionAcceleration-time graph of a particle moving in a straight line is as shown in figure. At time t=0, velocity of the particle is zero. Find
(a) average acceleration in a time interval from t=6s to t=12s,
(b) velocity of the particle at t=14s.
View SolutionThe velocity of a particle varies with time as →v=3ˆi+(4−5t)ˆjms−1. Find the average velocity of the particle for a time interval between t=0 and a time when the speed of the particle becomes minimum.
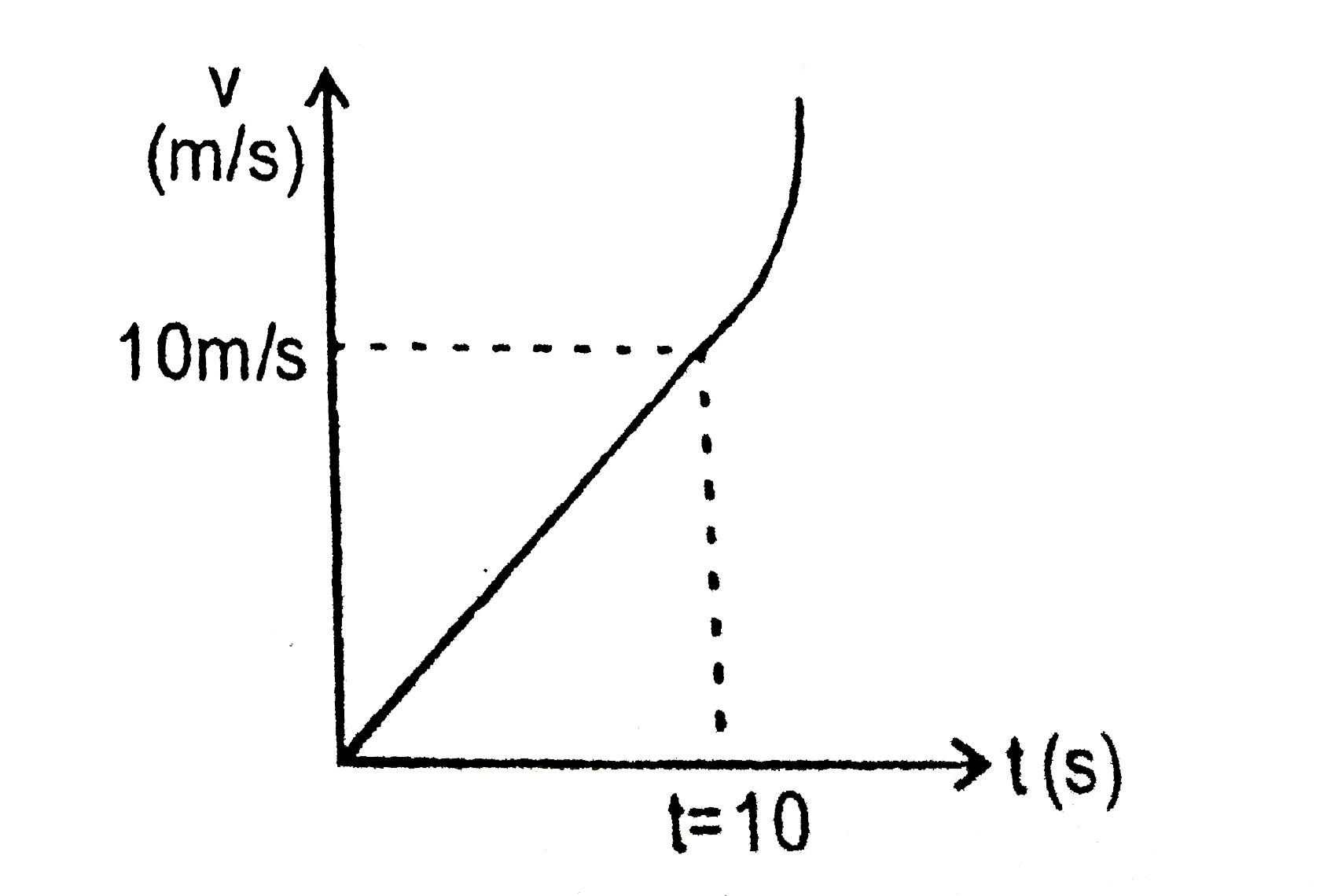
View SolutionA particle is moving in a straight line as :
a=1m/s20≤t≤10
a=2tt>10
where t is the time and a is the acceleration. If initial velocity of the particla is zero then :
View Solution
