A person standin on the top of a cliff 171 ft high has to throuw a packet to his friend standing on the ground 228 ft horizontally away. If he throws the packet directly aiming at the friend wilth a speed o 15.0 ft/s, how short will the packet fall?
The correct Answer is:B, C
To solve the problem, we need to analyze the motion of the packet thrown from the top of the cliff. The key points to consider are the height of the cliff, the horizontal distance to the friend, and the speed at which the packet is thrown.
1. Identify the Given Values:
- Height of the cliff (h) = 171 ft
- Horizontal distance to the friend (d) = 228 ft
- Speed of the packet (u) = 15 ft/s
2. Determine the Angle of Projection:
- The angle of projection (θ) can be found using the tangent function:
tan(θ)=heighthorizontal distance=171228
- Calculate θ:
θ=tan−1(171228)≈37∘
3. Break Down the Motion:
- The motion can be analyzed in two dimensions: horizontal (x-direction) and vertical (y-direction).
- The horizontal component of the initial velocity (u_x) is:
ux=u⋅cos(θ)=15⋅cos(37∘)
- The vertical component of the initial velocity (u_y) is:
uy=u⋅sin(θ)=15⋅sin(37∘)
4. Calculate Time of Flight:
- The time (t) it takes for the packet to fall to the ground can be found using the vertical motion equation:
y=uyt−12gt2
- Here, y = -171 ft (since it falls down), and g = 32 ft/s² (acceleration due to gravity).
- Rearranging gives:
−171=(15⋅sin(37∘))t−12(32)t2
5. Substituting Values:
- Calculate sin(37∘) and substitute:
−171=(15⋅0.6018)t−16t2
- This simplifies to:
−171=9.027t−16t2
- Rearranging gives:
16t2−9.027t−171=0
6. Solve the Quadratic Equation:
- Use the quadratic formula t=−b±√b2−4ac2a:
- Here, a=16, b=−9.027, c=−171.
- Calculate the discriminant:
D=b2−4ac=(−9.027)2−4⋅16⋅(−171)
- Find the roots using the quadratic formula.
7. Calculate Horizontal Distance:
- Once you have the time of flight (t), calculate the horizontal distance covered:
x=ux⋅t
8. Determine How Short the Packet Falls:
- The packet was aimed at a horizontal distance of 228 ft. The actual distance (x) calculated will show how short it fell:
Shortfall=228−x
Final Answer:
After performing the calculations, you will find the value of how short the packet falls.
- Height of the cliff (h) = 171 ft
- Horizontal distance to the friend (d) = 228 ft
- Speed of the packet (u) = 15 ft/s
- The angle of projection (θ) can be found using the tangent function:
tan(θ)=heighthorizontal distance=171228
- Calculate θ:
θ=tan−1(171228)≈37∘
- The motion can be analyzed in two dimensions: horizontal (x-direction) and vertical (y-direction).
- The horizontal component of the initial velocity (u_x) is:
ux=u⋅cos(θ)=15⋅cos(37∘)
- The vertical component of the initial velocity (u_y) is:
uy=u⋅sin(θ)=15⋅sin(37∘)
- The time (t) it takes for the packet to fall to the ground can be found using the vertical motion equation:
y=uyt−12gt2
- Here, y = -171 ft (since it falls down), and g = 32 ft/s² (acceleration due to gravity).
- Rearranging gives:
−171=(15⋅sin(37∘))t−12(32)t2
- Calculate sin(37∘) and substitute:
−171=(15⋅0.6018)t−16t2
- This simplifies to:
−171=9.027t−16t2
- Rearranging gives:
16t2−9.027t−171=0
- Use the quadratic formula t=−b±√b2−4ac2a:
- Here, a=16, b=−9.027, c=−171.
- Calculate the discriminant:
D=b2−4ac=(−9.027)2−4⋅16⋅(−171)
- Find the roots using the quadratic formula.
- Once you have the time of flight (t), calculate the horizontal distance covered:
x=ux⋅t
- The packet was aimed at a horizontal distance of 228 ft. The actual distance (x) calculated will show how short it fell:
Shortfall=228−x
After performing the calculations, you will find the value of how short the packet falls.
Topper's Solved these Questions
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Objective 2
10 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMACHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Exercises
34 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
Similar Questions
A person standing on the top of a tower 36m high has to throw a packet to his friend standing on the ground 48m horizontally away. If the throws a packet directly aiming the friend with a speed of 10m/s, how short will the packet fall ?
View SolutionA boy standing on the top of a tower 36 m high has a throw a packet to his friend standing on the ground 48 m horizontally away. If the throws a packet directly aiming the friend with a speed of 10ms−1, how short will be packet fall ?
View Solution
Knowledge Check
A man throws a packet from a tower directly aiming at his friend who is standing at a certain distance from the base which is same as the height of the tower. If the packet is thrown with a speed of 4ms−1 and it hits the ground midway between the tower base & his friend than the height of the tower is (g=10ms−2)
A5mB8mC3.2 mD13mThe giraffe is the tallest land mammal alive, its long legs and neck contributing to its impressive stature. Males can beup to 18ft.(5.5m tall), females a little less. In the wild, these beautiful creatures stretch their necks beyond those of antelope, kudu and even elephants to strip leaves from the untouched upper reaches of trees. The french zoologist Jean-Baptiste Lamarck is usually credited as the first person to suggest that long necks have evolved in giraffes because they allow them to get to the parts other herbivores cannot reach. As the giraffe lives “in places where the soil is nearly always arid and barren. It is obliged to browse on the leaves of trees and to make constant efforts to reach them’, he wrote in his 1809 book ‘Philosophie Zoologique’. “ From this habit long maintained in all its race, and that its neck is lengthened”. The English naturalist Charles Darwin also thought the giraffe’s extraordinary legs and neck must have something to do with foraging. “The giraffe, by its lofty stature, much elongated neck, fore-legs, head and tongue, has its whole frame beautifully adapted for browsing on the higher branches of trees”, he wrote in ‘On the origin of species’ in 1859. In short, giraffes’ long necks are the result of generation upon generation of repeated stretching and inheritance. During the dry season when feeding competition should be most intense giraffes generally feed from low shrubs, not tall trees. What’s more, giraffes feed most often and faster with their necks bent. Male giraffes often fight for access to females, a ritual referred to as “necking”. The rivals stand flank to flank, then start to whack each other with their heads. The top or back of the well-armoured skull is used as a club t o strike the neck, chest, ribs or legs of the opponent with a force capable of knocking a competitor off balance or unconscious. The largest males usually win these battles and do most of the breeding, says zoologist Anne Innis Dagg o f the university of waterloo in Ontario, Canada, who has been studying giraffes since the 1950s. “The o ther giraffes don’t get much breeding opportunity”. There is also evidence that females are more receptive to advances from larger males.
According to the passage, ‘necking’ is the :
How does a giraffe knock its opponent off balance or unconscious?ABy pulling the legs of the opponentBBy biting the opponent’s neck, chest and ribsCBy entangling its neck in the opponent’s neckDBy using its head as a club and hitting the opponentThe giraffe is the tallest land mammal alive, its long legs and neck contributing to its impressive stature. Males can beup to 18ft.(5.5m tall), females a little less. In the wild, these beautiful creatures stretch their necks beyond those of antelope, kudu and even elephants to strip leaves from the untouched upper reaches of trees. The french zoologist Jean-Baptiste Lamarck is usually credited as the first person to suggest that long necks have evolved in giraffes because they allow them to get to the parts other herbivores cannot reach. As the giraffe lives “in places where the soil is nearly always arid and barren. It is obliged to browse on the leaves of trees and to make constant efforts to reach them’, he wrote in his 1809 book ‘Philosophie Zoologique’. “ From this habit long maintained in all its race, and that its neck is lengthened”. The English naturalist Charles Darwin also thought the giraffe’s extraordinary legs and neck must have something to do with foraging. “The giraffe, by its lofty stature, much elongated neck, fore-legs, head and tongue, has its whole frame beautifully adapted for browsing on the higher branches of trees”, he wrote in ‘On the origin of species’ in 1859. In short, giraffes’ long necks are the result of generation upon generation of repeated stretching and inheritance. During the dry season when feeding competition should be most intense giraffes generally feed from low shrubs, not tall trees. What’s more, giraffes feed most often and faster with their necks bent. Male giraffes often fight for access to females, a ritual referred to as “necking”. The rivals stand flank to flank, then start to whack each other with their heads. The top or back of the well-armoured skull is used as a club t o strike the neck, chest, ribs or legs of the opponent with a force capable of knocking a competitor off balance or unconscious. The largest males usually win these battles and do most of the breeding, says zoologist Anne Innis Dagg o f the university of waterloo in Ontario, Canada, who has been studying giraffes since the 1950s. “The o ther giraffes don’t get much breeding opportunity”. There is also evidence that females are more receptive to advances from larger males.
According to the passage, ‘necking’ is the :
Giraffe’s feeding is faster when it feeds with its neck:AStraightBelongatedCbentDentangled
A boy standing on the top of a tower of height 54 ft. throws a packet with a speed of 20 ft/s directly aiming towards his friend standing on the ground at a distance of 72 ft from the foot of the tower. The packet falls short of the person on the ground by x×163 ft. The value of x is
View SolutionThere is a tall cylindrical building standing in a field. Radius of the cylinder is R=8m. A boy standing at A (at a distance of 10 m from the centre of the cylindrical base of the building) knows that his friend is standing at B behind the building. The line joining A and B passes through the centre of the base of the building. Distance between A and B is 50 m. A wants to throw a ball to B but he realizes that the building is too tall and he cannot throw the ball over it. He throws the ball at a speed of 20m/s such that his friend at B has to move minimum distance to catch it.
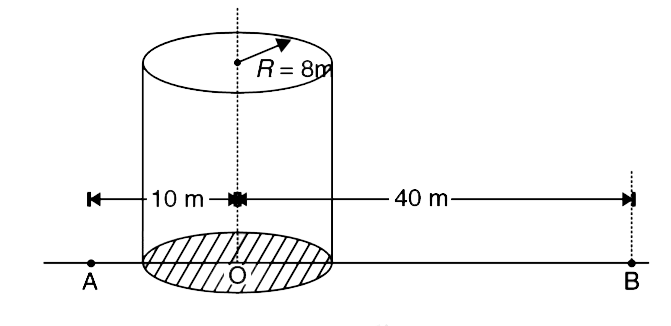
(a) What is the minimum distance that boy at B will have to move to catch the ball?
(b) At what angle to the horizontal does the boy at A throws the ball? Assume that the ball is released and caught at same height above the ground.
[Take g=10m/s2 and sin−1(0.75)≅48.6∘
View SolutionA popular game in Indian villages is goli which is played with small glass balls called golis. The goli of one player is situatted at a distance of 2.0 m from the goli of the second player. This second player has to project his goli by keeping the thumb of the left hand at the place of his goli, holding the goli between his two middlefilngers and making the throw. If he projected is 19.6 cm from the ground and the goli is to be projected horizontally, with what speed shold it be projected so that it directly hits the stationary goli without falling on the ground earlier?
View SolutionA wet ball is projected horizontally at a speed of u=10m/s from the top of a tower h=31.25m high. Water drops detach from the ball at regular intervals of Δt=1.0s after the throw.
(a) How many drops will detach from the ball before it hits the ground.
(b) How far away the drops strike the ground from the point where the ball hits the ground?
View SolutionThe giraffe is the tallest land mammal alive, its long legs and neck contributing to its impressive stature. Males can beup to 18ft.(5.5m tall), females a little less. In the wild, these beautiful creatures stretch their necks beyond those of antelope, kudu and even elephants to strip leaves from the untouched upper reaches of trees. The french zoologist Jean-Baptiste Lamarck is usually credited as the first person to suggest that long necks have evolved in giraffes because they allow them to get to the parts other herbivores cannot reach. As the giraffe lives “in places where the soil is nearly always arid and barren. It is obliged to browse on the leaves of trees and to make constant efforts to reach them’, he wrote in his 1809 book ‘Philosophie Zoologique’. “ From this habit long maintained in all its race, and that its neck is lengthened”. The English naturalist Charles Darwin also thought the giraffe’s extraordinary legs and neck must have something to do with foraging. “The giraffe, by its lofty stature, much elongated neck, fore-legs, head and tongue, has its whole frame beautifully adapted for browsing on the higher branches of trees”, he wrote in ‘On the origin of species’ in 1859. In short, giraffes’ long necks are the result of generation upon generation of repeated stretching and inheritance. During the dry season when feeding competition should be most intense giraffes generally feed from low shrubs, not tall trees. What’s more, giraffes feed most often and faster with their necks bent. Male giraffes often fight for access to females, a ritual referred to as “necking”. The rivals stand flank to flank, then start to whack each other with their heads. The top or back of the well-armoured skull is used as a club t o strike the neck, chest, ribs or legs of the opponent with a force capable of knocking a competitor off balance or unconscious. The largest males usually win these battles and do most of the breeding, says zoologist Anne Innis Dagg o f the university of waterloo in Ontario, Canada, who has been studying giraffes since the 1950s. “The o ther giraffes don’t get much breeding opportunity”. There is also evidence that females are more receptive to advances from larger males.
According to the passage, ‘necking’ is the :
View Solution
