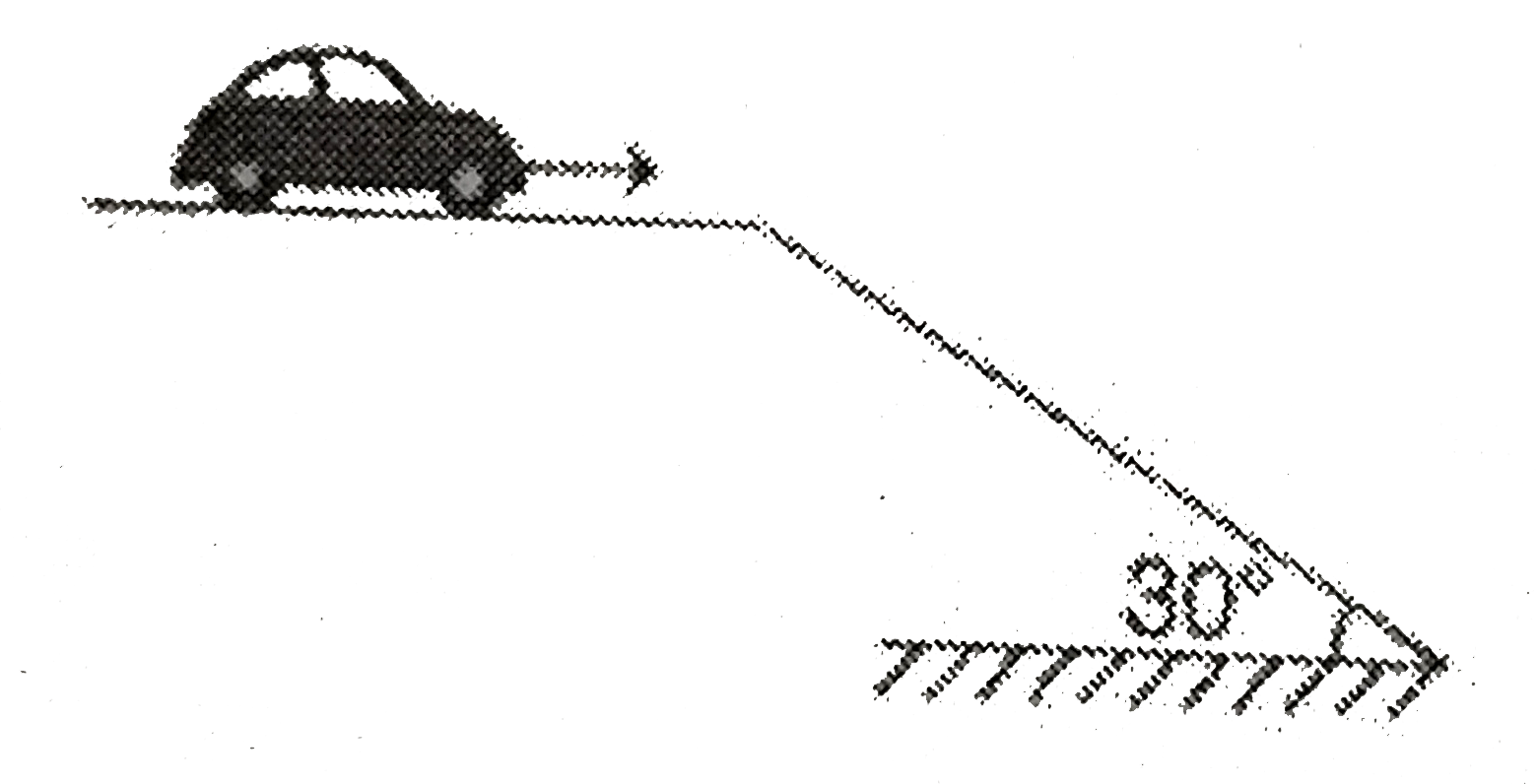
A car is going at a speed of 21.6 km/hr when it encounters a 12.8 m long slope of angle $30^{0}$ figure. $\frac{1}{2 \sqrt{3}}$ . Shwo tht no matter how hard the driver applies the brakes, tehcar will reach the bottim with a speed greater than 36 km/hr. Take $g = 10 \frac{m}{s^{2}}$

Video Solution

Text Solution

Verified by Experts

Hardest brake indicates that maximum force of friction is developed between car's tyre and road.
Maximum frictioN/Al force $= μ R$
From the free body diagram,
$R - m g cos θ = 0$
$\to R = m g cos θ$ ……..i
$and μ R + m a - m g sin θ = 0$ ………ii
$\to μ m g cos θ + m a - m g sin θ = 0$

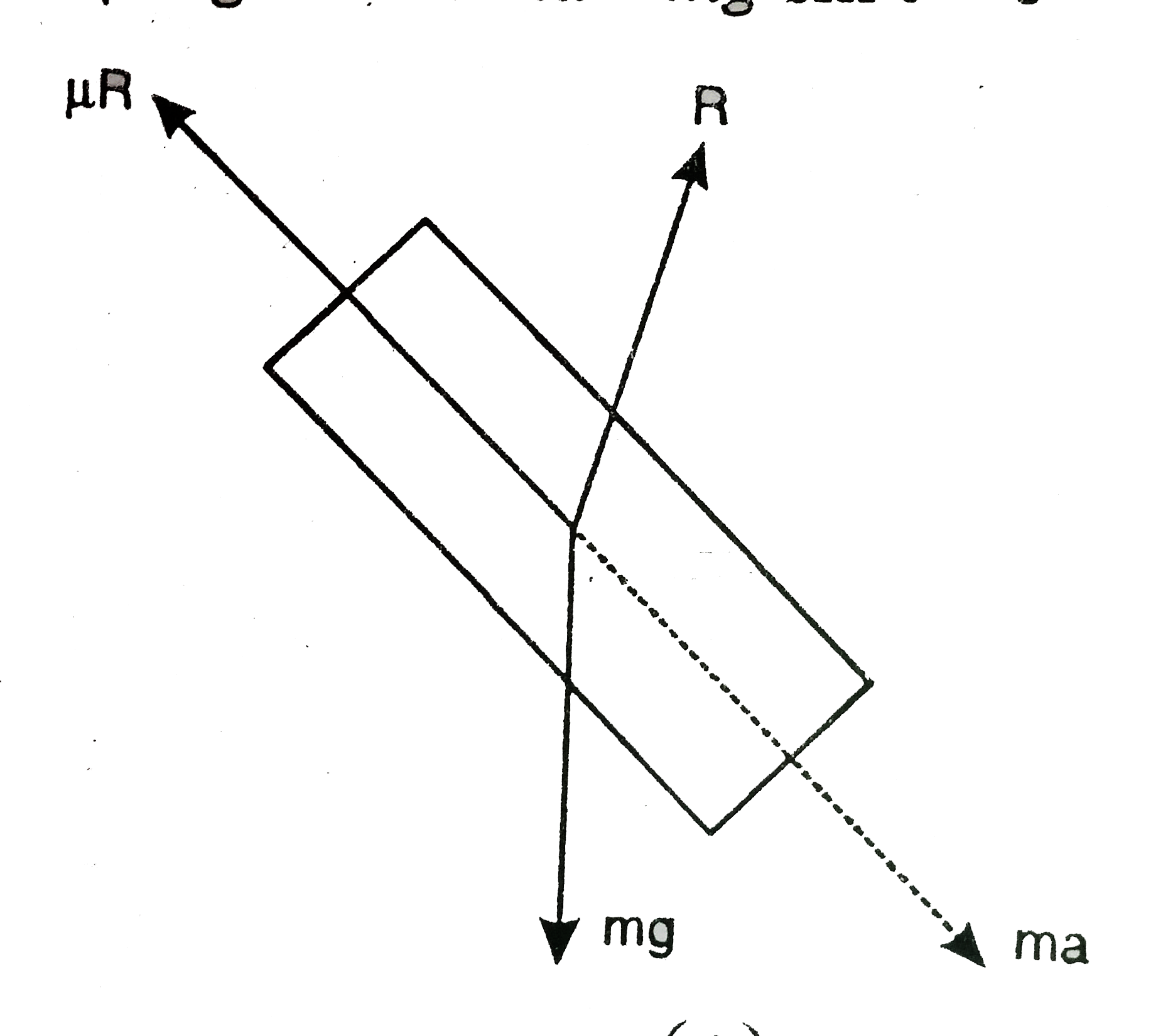
$\to m g cos θ + a - 10 \times (\frac{1}{2}) = 0$
$\to a = 5 - [1 - 2 \sqrt{3}] \times 10 (\frac{\sqrt{3}}{2})$
$= 5 - 2.5 = 2.5 \frac{m}{s^{2}}$
$S = 12.8 \frac{m}{s^{2}}$
$u = 6 \frac{m}{s}, v e l o c i t y a t t h e e n d o f \in c l \in e < b r >$ v=sqrt(u^2+2as) $< b r >$ =sqsrt(6^2+2(2.5)(12.8)) $< b r >$ =sqrt(36+64)
$= 10 \frac{m}{s} = 36 k \frac{m}{h} r < b r > H e n c e, t h e h a r d e r t h e d r i v e r a p p l i e s t h e b r a k e s, m t h e l o w e r w i l l b e t h e v e l o c i t y o f t h e c r w h e n i t r e a c h e s t h e g r o u n d i . e . a t$ 36 km/hr.

A car going at a speed of 6m/s when it encounters a 15m slope of angle $30^{\circ}$ . The friction coefficient between the road an tyre is 0.5. the driver applies the brakes. The minimum speed of car with with which it can reach the bottom is $(g = 10 m / s^{2})$

A4m/s
B3m/s
C7.49m/s
D8.45m/s
A gun is fired from behind a train the driver of train hears the sound $1 \frac{1}{2}$ min later than guard. Find the length of train if the speed of train & sound are 60 km/hr and 1100 m/min.
A100 m
B150 m
C125 m
D75 m
A train is travelling at a speed of $90 k m / h$ . Brakes are applied so as to produce a uniform acceleration of $- 0.5 m / s^{2}$ . Find how far the train will go before it is brought to rest.
A484 m
B250 m
C625 m
D400 m