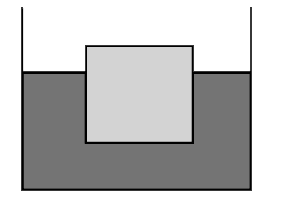
A cubical block of ice of mass m and edge L is placed in a large tray of mass M. If the ice melts, how far does the centre of mass of the system ''ice plus tray'' come down?
Consier ure. Suppose the centre of massof the tray is a distace x1 above the origin and that of the ice is a distance x2 above the origin. The height of the centre of mass of the ice tray system is
x=mx2+mx1m+M
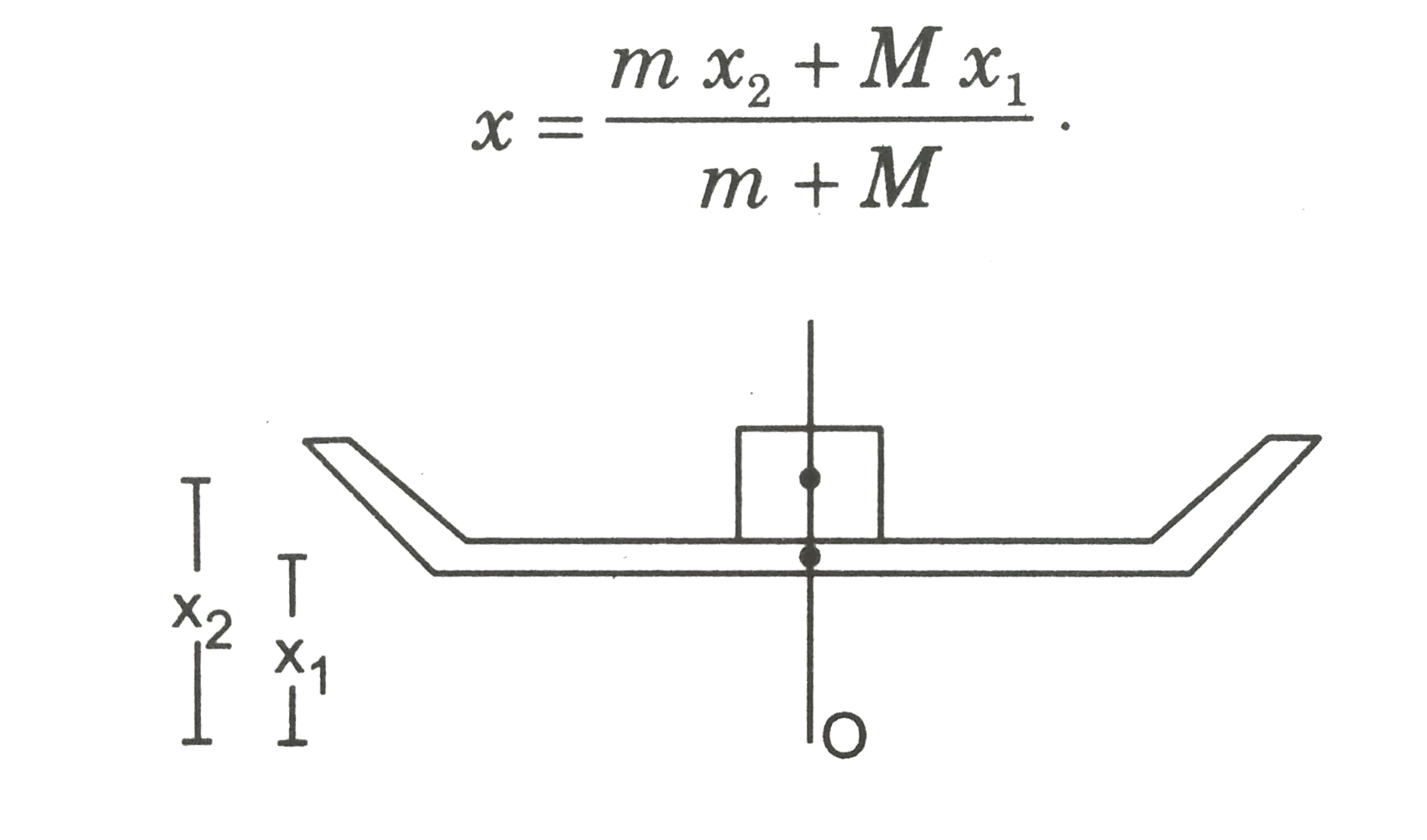
When the ice melts, the water of mass m spreads on the surface of the tray. As the tray is large, the height of water is then on the surfave of the tray and is at a distance x2−L2 above the origin. THe new centre of masss of theh ice -tray system will be at the height
x'=m(x2−L2)+Mx1m+M
THe shift in the centre of mass =x−x'=mL2(m+M)
Similar Questions
A cubical block of ice of mass m and edge L is placed in a large tray of mass M. If the ice melts, how far does the centre of mass of the system ''ice plus tray'' come down?
View SolutionConsider a gravity free hall in which a tray of mas M, carrying a cubical block of icce of mas m and edge L, is at rest in the middle.. If the ice melts, by what distnce does the centre of mass of the tray plus the ice system descend?
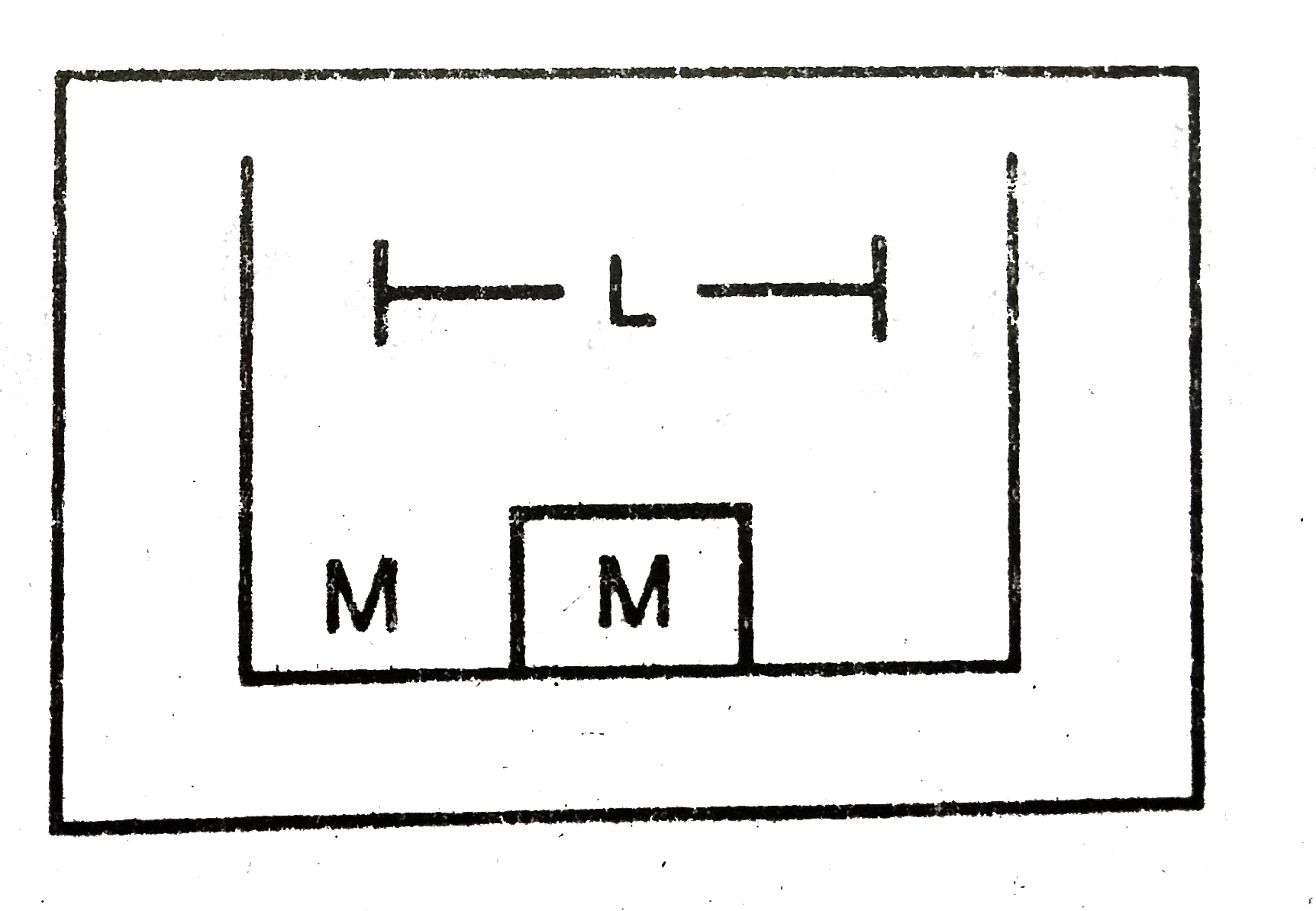
View Solution
Knowledge Check
Two point masses m and M are separated by a distance L . The distance of the centre of mass of the system from m is
AL(m/M)BL(M/m)CL(Mm+M)DL(mm+M)Two point masses m and M are separated bya distance L . The distance of the centre of mass of the system from m is
AL(m/M)BL(M/m)CL(Mm+M)DL(mm+M)
A cubical ice block of side length 'a' is floating in water in a beaker. Find the change in height of the centre of mass of (water+ice) system when the ice block melts completely. It is given that ratio of mass of water to mass of ice originally in the containe is 4:1.
View SolutionA block of mass m slides over a smooth wedge of mass M which is placed over a rough horizontal surface . The centre of mass of the system will move towards left

Here μ = coefficient of friction between the wedge and the ground .
View SolutionA block of mass m is placed at rest on a smooth wedge of mass M placed at rest on a smooth horizontal surface. As the system is released

View SolutionA cubical block of mass M and edge a slides down a rougg inclined plane of inclination θ with a uniform velocity. The torque of the normal force on the block about its centre has magnitude.
View SolutionAn ice cream cone of mass M has base radius R and height h. Assume its wall to be thin and uniform. When ice cream is filled inside it (so as to occupy the complete conical space) its mass becomes 5M. Find the distance of the centre of mass of the ice cream filled cone from its vertex.
View SolutionA bullet of mass 2 kg and speed 10 m/s hits a large block of ice at 0∘C and stops. If all the KE of bullet converts to heat, find the amount of ice that melted.
View Solution
