Question

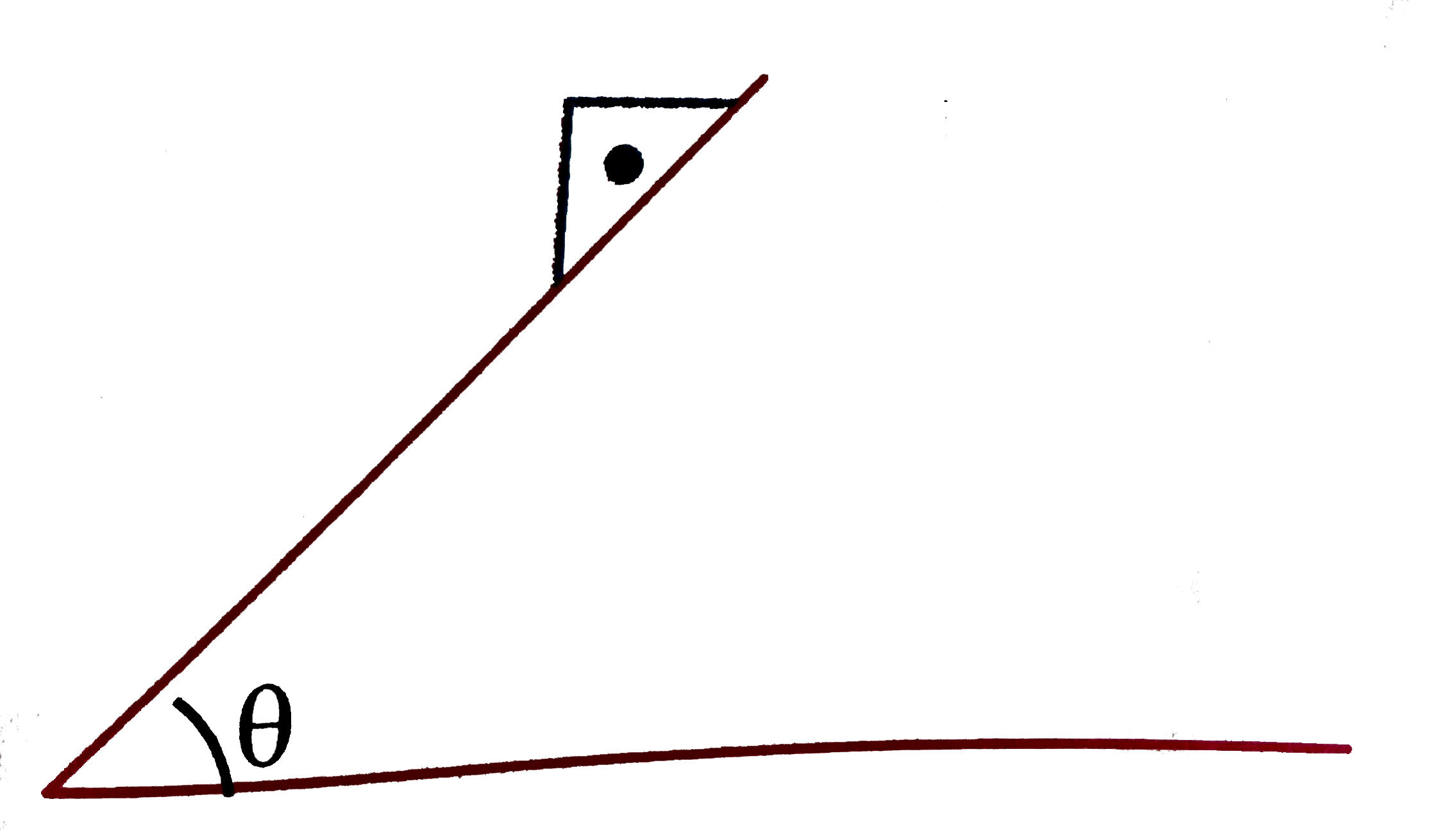
A cubical block of mass M and edge a slides down a rougg inclined plane of inclination $\theta$ with a uniform velocity. The torque of the normal force on the block about its centre has magnitude.

Options

A. zero
B. Mga
C. `Mgasintheta`
D. `1/2Mgasintheta`

Answer

D

Explanation

To find the torque of the normal force on a cubical block of mass $$M$$ and edge $$a$$ sliding down a rough inclined plane with an inclination $$\theta$$ at uniform velocity, we can follow these steps:

Step 1: Identify the Forces Acting on the Block
The forces acting on the block are:
- The weight of the block, \( \vec{W} = M \vec{g} \) acting vertically downwards.
- The normal force \( \vec{N} \) acting perpendicular to the inclined surface.
- The frictional force \( \vec{F} \) acting parallel to the inclined surface, opposing the motion of the block.

Step 2: Resolve the Weight into Components
The weight can be resolved into two components:
- Perpendicular to the incline: \( W_{\perp} = Mg \cos \theta \)
- Parallel to the incline: \( W_{\parallel} = Mg \sin \theta \)

Step 3: Analyze Forces in the Direction Perpendicular to the Incline
Since the block is moving with uniform velocity down the incline, the net force in the direction perpendicular to the incline must be zero. Therefore, the normal force \( N \) must balance the perpendicular component of the weight:
\( N = Mg \cos \theta \)

Step 4: Analyze Forces in the Direction Parallel to the Incline
For the block to slide down with uniform velocity, the frictional force must balance the parallel component of the weight:
\( F = Mg \sin \theta \)

Step 5: Calculate the Torque Due to Normal Force
The torque \( \tau \) due to the normal force about the center of the block is given by:
\( \tau_N = N \cdot d \)
where \( d \) is the perpendicular distance from the line of action of the normal force to the center of the block. Since the normal force acts at the edge of the block, the distance from the center to the edge is \( \frac{a}{2} \).

Thus, the torque due to the normal force is:
\( \tau_N = N \cdot \frac{a}{2} = (Mg \cos \theta) \cdot \frac{a}{2} = \frac{M g a \cos \theta}{2} \)

Step 6: Calculate the Torque Due to Friction
The torque due to the frictional force about the center of the block is:
\( \tau_F = F \cdot d \)
The distance \( d \) is also \( \frac{a}{2} \) since the friction acts at the bottom edge of the block. Therefore:
\( \tau_F = (Mg \sin \theta) \cdot \frac{a}{2} = \frac{M g a \sin \theta}{2} \)

Step 7: Equate the Torques
Since the block is not rotating, the net torque must be zero:
\( \tau_N = \tau_F \)
Thus:
\( \frac{M g a \cos \theta}{2} = \frac{M g a \sin \theta}{2} \)

Final Result
The torque of the normal force about the center of the block is:
\( \frac{M g a \cos \theta}{2} \)