A projectile is fired at a spedd of 100 m/s at an angel of $37^{0}$ above the horizontal. At the highest point, the projectile breaks into two parts of mass ratio 1:3 the smaller coming to rest. Findthe distance from the launching point to the where the heavier piece lands.

Video Solution

Text Solution

Verified by Experts

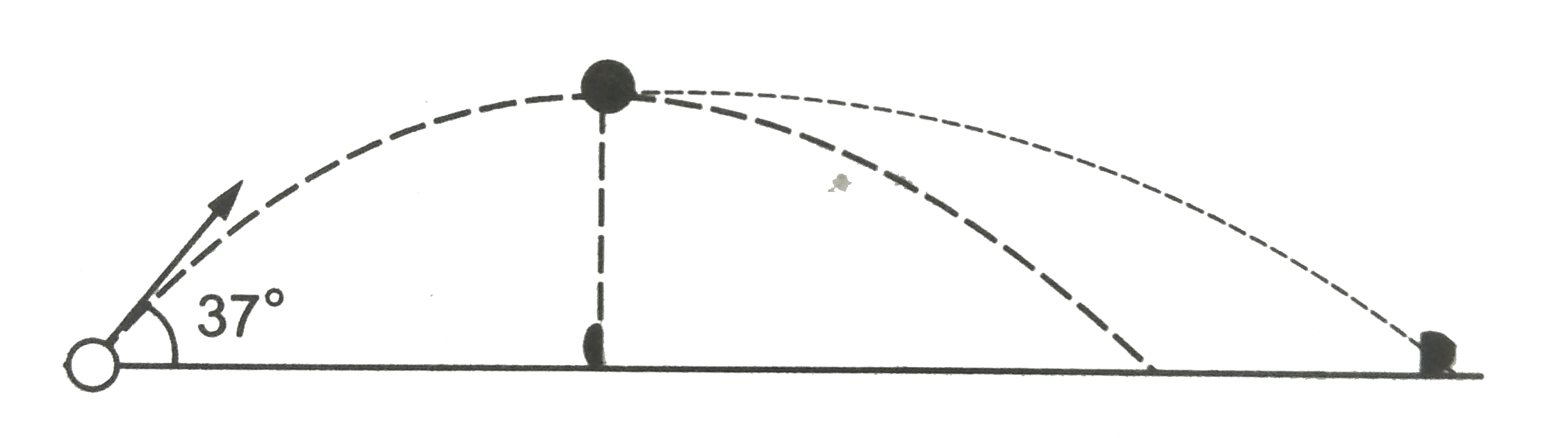
See ure. At the highest point, the projectrile has horizontal velocity. The lighter part comes to rest. Hence the heavier part willl move with inceased horizontal velocity. In vertical direction both parts have zero velocity and undergo same acceleration, hence they willl cover equal vertical displacements in a given time. Thus both will hit the ground together As interN/Al forces do not affect the motion of teh centre of mass, the centre of mass hits the ground at teh positin where the origiN/Al projectile would have landed. Te range of the origiN/Al projectile is
$x_{C M} = \frac{2 u^{2} \sin θ \cos θ}{g} = \frac{2 \times 10^{4} \times \frac{3}{5} \times \frac{4}{5}}{10} m$
=960m.
The centre of mass will hit the grouond at this position. As the smaller block comes to rest after breaking. It falls down vertically and hits the ground at half othe range i.e., at $x = 480 m$ . If the heavier block hits the ground at $x_{2}$ then
$x_{C M} = \frac{m_{1} x_{1} + m_{2} x_{2}}{m_{2} + m_{2}}$
$960 m = (\frac{M}{4} \times 480 m + \frac{3 M}{4} \times x_{2})$
or,
$x_{2} = 1120 m$

A projectile of mass 20 kg is fired with a velocity of $400 m / s$ at an angle of $45^{\circ}$ with the horizontal .At the highest point of the trajectocy the projectile explodes into two fragments of equal mass,one of which falls vertically downward with zero initial speed, The distance of the point where the other fragment falls from the point offiring is:
A24000 m
B16000 m
C32000 m
D8000 m
A bullet fired from gun with a velocity 30 m/s at an angle of $60^{\circ}$ with horizontal direction. At the highest point of its path, the bullet explodes into two parts with masses in the ratio 1 : 3. The lighter mass comes to rest immediately. Then the speed of the heavier mass is
A30 m/s
B20 m/s
C10 m/s
D5 m/s
A particle is projected such that its horizontal range would be R. At the highest point, the particle breaks into two identical parts P and Q. If P comes to rest, the horizontal distance of point from the point of projection (i.e. origin) where the particle Q lands on the ground is
A1.5 R
BR
C2.5 R
D2 R