Question

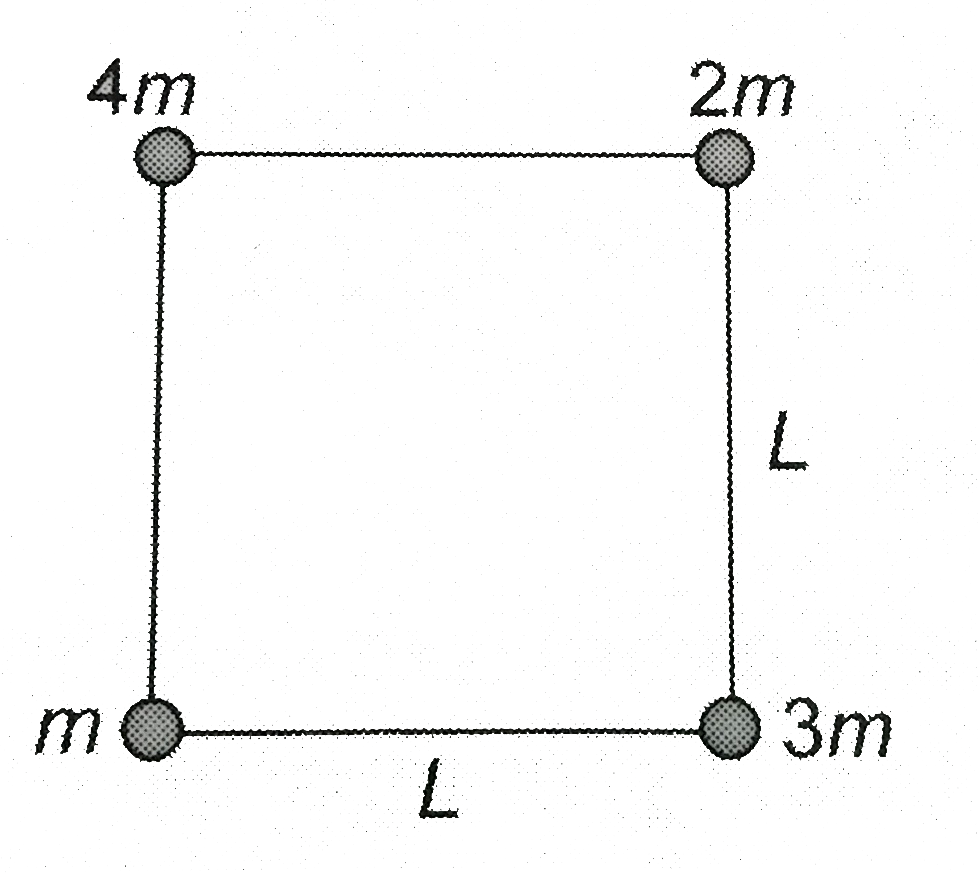
Four particles having masses, m, wm, 3m, and 4m are placed at the four corners of a square of edge a. Find the gravitational force acting on a particle of mass m placed at the centre.

Answer

A::B::D

Explanation

To find the gravitational force acting on a particle of mass $$m$$ placed at the center of a square with four particles at its corners, we can follow these steps:

1. Identify the Setup:
We have a square with side length \( a \). The masses at the corners are \( m \), \( 2m \), \( 3m \), and \( 4m \). The mass \( m \) is placed at the center of the square.

2. Calculate the Distance from the Center to the Corners:
The distance \( R \) from the center of the square to any corner is given by:
\( R = \frac{a}{\sqrt{2}} \)

3. Calculate the Gravitational Force from Each Mass:
The gravitational force \( F \) between two masses \( m_1 \) and \( m_2 \) separated by a distance \( r \) is given by:
\( F = \frac{G m_1 m_2}{r^2} \)
For each corner mass, we can calculate the force acting on the mass \( m \) at the center.

- Force due to mass \( m \) at corner:
\( F_1 = \frac{G m \cdot m}{R^2} = \frac{G m^2}{\left(\frac{a}{\sqrt{2}}\right)^2} = \frac{2G m^2}{a^2} \)

- Force due to mass \( 2m \) at corner:
\( F_2 = \frac{G (2m) \cdot m}{R^2} = \frac{G \cdot 2m^2}{\left(\frac{a}{\sqrt{2}}\right)^2} = \frac{4G m^2}{a^2} \)

- Force due to mass \( 3m \) at corner:
\( F_3 = \frac{G (3m) \cdot m}{R^2} = \frac{G \cdot 3m^2}{\left(\frac{a}{\sqrt{2}}\right)^2} = \frac{6G m^2}{a^2} \)

- Force due to mass \( 4m \) at corner:
\( F_4 = \frac{G (4m) \cdot m}{R^2} = \frac{G \cdot 4m^2}{\left(\frac{a}{\sqrt{2}}\right)^2} = \frac{8G m^2}{a^2} \)

4. Determine the Resultant Forces:
The forces \( F_1 \) and \( F_3 \) act in opposite directions along one diagonal, and \( F_2 \) and \( F_4 \) act along the other diagonal. Since they are symmetrical, we can calculate the net force in one direction and then combine them.

- Net force along one diagonal:
\( F_{\text{net, diagonal 1}} = F_4 - F_2 = \frac{8G m^2}{a^2} - \frac{4G m^2}{a^2} = \frac{4G m^2}{a^2} \)

- Net force along the other diagonal:
\( F_{\text{net, diagonal 2}} = F_3 - F_1 = \frac{6G m^2}{a^2} - \frac{2G m^2}{a^2} = \frac{4G m^2}{a^2} \)

5. Calculate the Resultant Force:
Since both net forces are equal and act at right angles to each other, we can use the Pythagorean theorem to find the resultant force \( F_{\text{net}} \):
\( F_{\text{net}} = \sqrt{(F_{\text{net, diagonal 1}})^2 + (F_{\text{net, diagonal 2}})^2} = \sqrt{\left(\frac{4G m^2}{a^2}\right)^2 + \left(\frac{4G m^2}{a^2}\right)^2} \)
\( = \sqrt{2 \left(\frac{4G m^2}{a^2}\right)^2} = \frac{4G m^2}{a^2} \sqrt{2} \)

6. Final Result:
Thus, the gravitational force acting on the particle of mass \( m \) at the center is:
\( F_{\text{net}} = \frac{4\sqrt{2} G m^2}{a^2} \)