The pressure in a liquid at two points in the same horizontal plane are equal. Consider an elevator accelerating upward and a car accelerating on a horizontal road. The above statement is correct in
the car only
the elevator only
both of them
neither of them
The correct Answer is:B
To solve the problem, we need to analyze the pressure in a liquid at two points in the same horizontal plane under two different scenarios: an elevator accelerating upwards and a car accelerating on a horizontal road.
1. Understanding Pressure in a Liquid:
The pressure at a point in a liquid is given by the formula:
P=P0+ρgh
where P0 is the atmospheric pressure, ρ is the density of the liquid, g is the acceleration due to gravity, and h is the depth of the liquid column above the point.
2. Case 1: Elevator Accelerating Upwards:
- When the elevator accelerates upwards, the liquid inside the elevator experiences an effective increase in pressure due to the upward acceleration.
- However, the surface of the liquid remains horizontal because the entire system (elevator and liquid) is accelerating uniformly.
- Therefore, at any two points in the same horizontal plane within the liquid, the depth h remains the same, and thus the pressure at these two points will be equal:
PA=P0+ρghAandPB=P0+ρghB
Since hA=hB, it follows that PA=PB.
3. Case 2: Car Accelerating on a Horizontal Road:
- In this scenario, when the car accelerates horizontally, the liquid inside the car will not remain level.
- Due to the inertia of the liquid, it will tilt towards the back of the car, creating a sloped surface.
- As a result, the depth of the liquid at one end of the container will be different from the depth at the other end. Let’s denote these depths as hA and hB.
- Therefore, the pressure at these two points will be different:
PA=P0+ρghAandPB=P0+ρghB
Since hA≠hB, it follows that PA≠PB.
4. Conclusion:
- From the analysis, we conclude that the pressure in a liquid at two points in the same horizontal plane is equal when the elevator is accelerating upwards, but not equal when the car is accelerating on a horizontal road.
- Therefore, the correct statement is that the pressure in the liquid at two points in the same horizontal plane is equal in the case of the elevator.
Final Answer:
The above statement is correct in the case of the elevator accelerating upwards.
---
The pressure at a point in a liquid is given by the formula:
P=P0+ρgh
where P0 is the atmospheric pressure, ρ is the density of the liquid, g is the acceleration due to gravity, and h is the depth of the liquid column above the point.
- When the elevator accelerates upwards, the liquid inside the elevator experiences an effective increase in pressure due to the upward acceleration.
- However, the surface of the liquid remains horizontal because the entire system (elevator and liquid) is accelerating uniformly.
- Therefore, at any two points in the same horizontal plane within the liquid, the depth h remains the same, and thus the pressure at these two points will be equal:
PA=P0+ρghAandPB=P0+ρghB
Since hA=hB, it follows that PA=PB.
- In this scenario, when the car accelerates horizontally, the liquid inside the car will not remain level.
- Due to the inertia of the liquid, it will tilt towards the back of the car, creating a sloped surface.
- As a result, the depth of the liquid at one end of the container will be different from the depth at the other end. Let’s denote these depths as hA and hB.
- Therefore, the pressure at these two points will be different:
PA=P0+ρghAandPB=P0+ρghB
Since hA≠hB, it follows that PA≠PB.
- From the analysis, we conclude that the pressure in a liquid at two points in the same horizontal plane is equal when the elevator is accelerating upwards, but not equal when the car is accelerating on a horizontal road.
- Therefore, the correct statement is that the pressure in the liquid at two points in the same horizontal plane is equal in the case of the elevator.
The above statement is correct in the case of the elevator accelerating upwards.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - HC VERMACHAPTER - FLUID MECHANICSEXERCISE - Objective 2
8 Videos
- FLUID MECHANICSBOOK - HC VERMACHAPTER - FLUID MECHANICSEXERCISE - Exercises
35 Videos
- FLUID MECHANICSBOOK - HC VERMACHAPTER - FLUID MECHANICSEXERCISE - Short Answer
16 Videos
- CIRCULAR MOTIONBOOK - HC VERMACHAPTER - CIRCULAR MOTIONEXERCISE - Exercises
30 Videos
- FRICTIONBOOK - HC VERMACHAPTER - FRICTIONEXERCISE - Exercises
31 Videos
Similar Questions
Knowledge Check
A car accelerates on a horizontal road due to the force exerted by
Athe engine of the carBthe driver of the carCthe earthDthe road
A wheel is rolling without slipping on a horizontal plane with velocity v and acceleration a of centre of mass as shown in figure. Acceleration atAA is vertically upwardsBB may be vertically downwardsCC cannot be horizontalDA point on the rim may be horizontal leftwards.A wheel is rolling on a horizontal plane. At a certain instant it has a velocity v and acceleration a of CM as shown in figure . Acceleration of
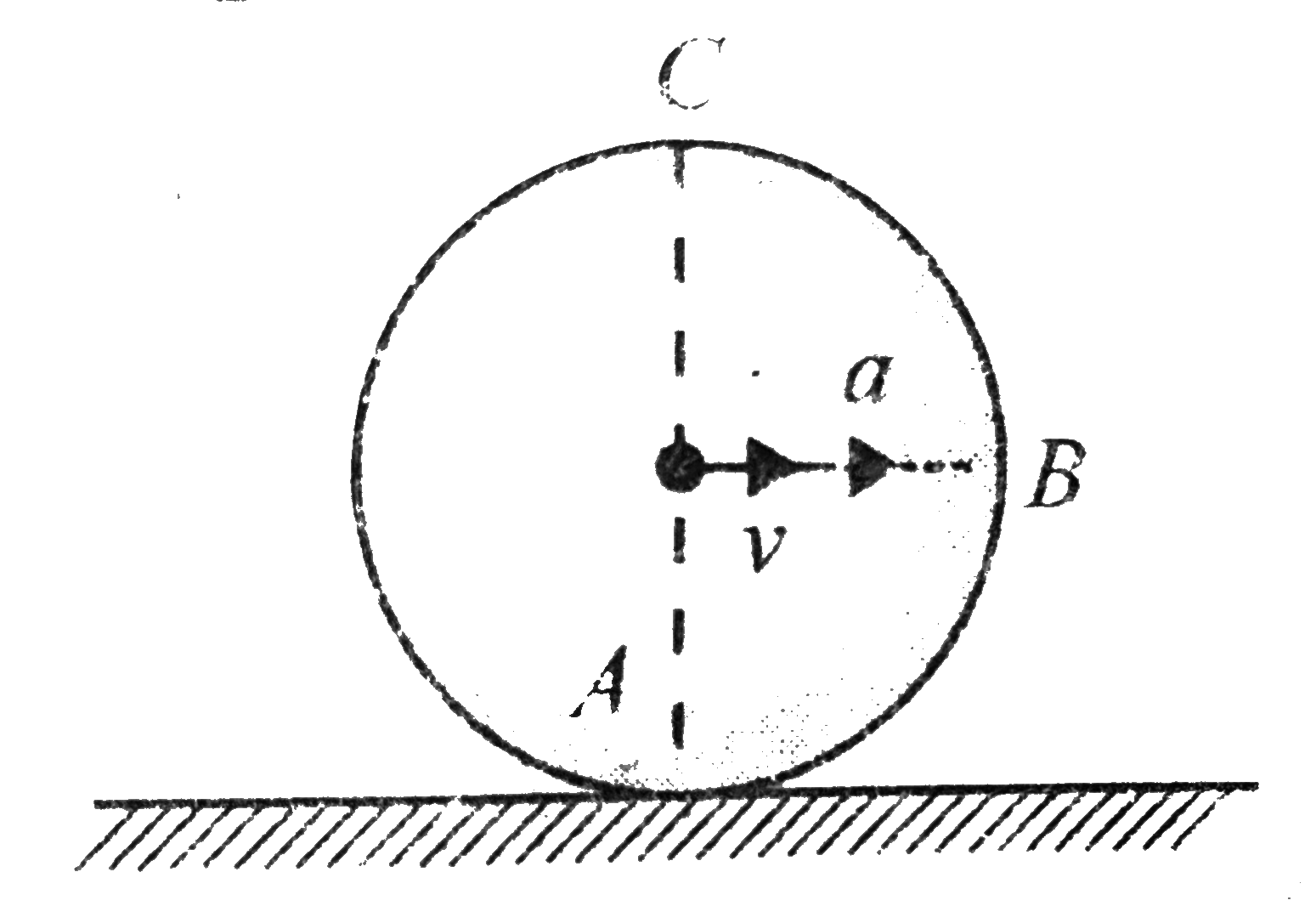 AA is vertically upwardsBB may be vertically down-wardsCC cannot be horizontalDsome point on the rim may he horizontal leftwards
AA is vertically upwardsBB may be vertically down-wardsCC cannot be horizontalDsome point on the rim may he horizontal leftwards
A barometer kept in an elevator accelerating upward reads 76 cm. The air pressure in the elevator is
View SolutionA spring mass system oscillates in a car. If the car accelerates on a horizontal road, the frequency of oscillation will
View SolutionA projectile is fired from horizontal at an angle θ . What are the horizontal and vertical acceleration at the highest point ?
View SolutionTwo bodies make an elastic head on colision on a smooth horizontal table kept in a car.Do you expect a change in the result if the car is accelerated on a horizontal road because of the noninertial character of the frame? Does the equation velocityof separation = velocity of approach remain valid in n accelerating car? Does the equation final momentum =initial momentum remain valid in the accelerating car?
View SolutionThe minimum work done required to accelerate a truck on a horizontal road from rest to speed v
View Solution
