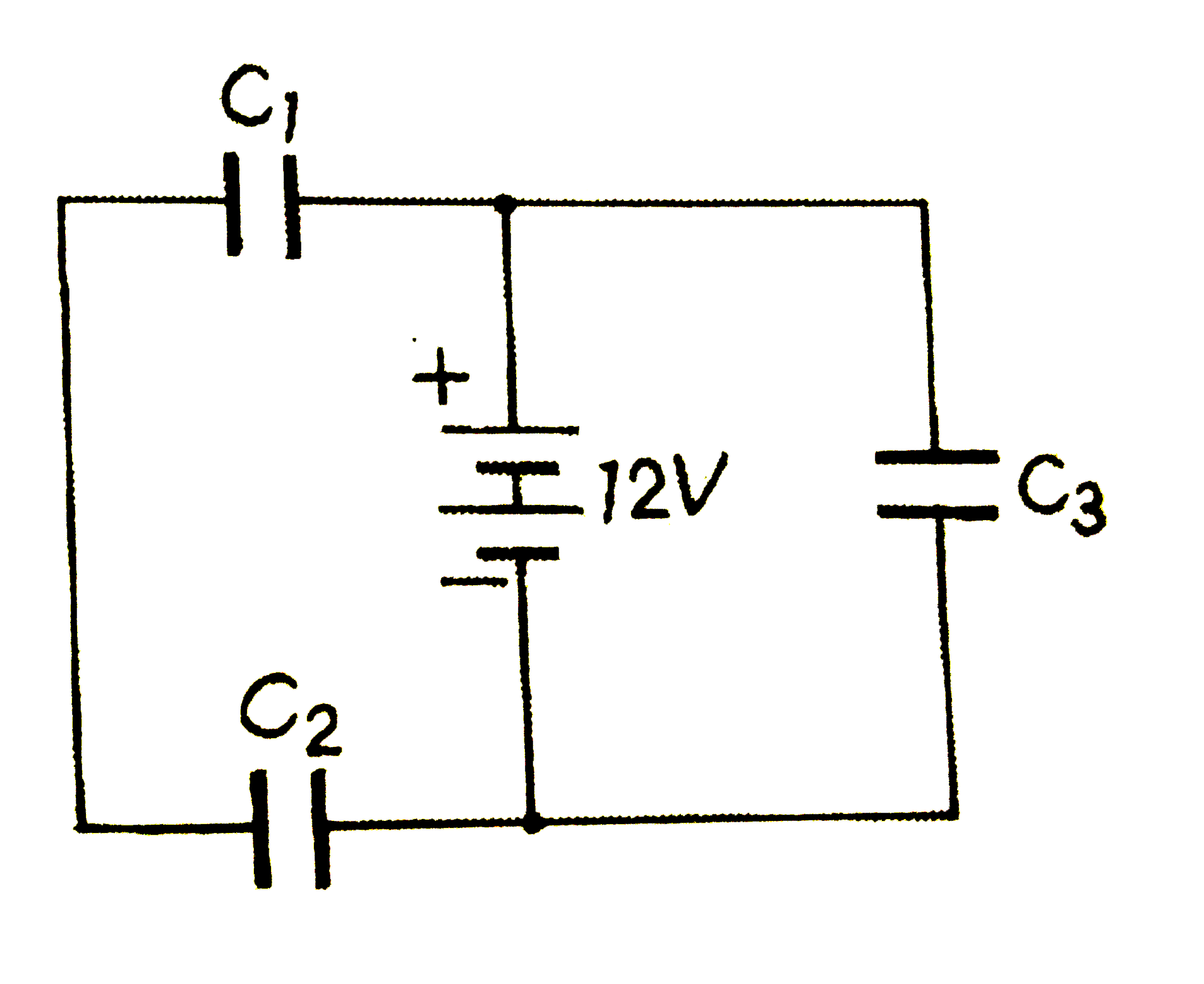
Three capacitors C1,C2andC3 are connected as show in the figure to a battery of V volt. If the capacitor C3 breaks down electrically, the change in total charge on the combination of capacitors is
(C1+C2)V[1−(C3C1+C2+C3)]
(C1+C2)V[1−(C1+C2C1+C2+C3)]
(C1+C2)V[1+(C3C1+C2+C3)]
(C1+C2)V[1+(C2C1+C2+C3)]
The correct Answer is:A
Since , C1andC2 are parallel, so their equivalent capacitance will be (C1+C2) Now (C1+C2)andC3 are in series, so the eat equvalent capacitance of circuit.
1C=1C3+1C1+C2=C1+C2+C3(C1+C2)C3
C1=(C1+C2)C3C1+C2+C3
since V is the voltage of the battery so change on this system .
q = CV
⇒q=(C1+C2C3VC1+C2+C3)
If the capacitor C3 breaks down, then total equivalent equivalent capacitance
C=C1+C2
Newm chage stored
q = C' V
q' = (C1+C2)V
Change in total charge
Δq=q'−q [∴q>q]
(C1+C2)V−(C1+C2)C3VC1+C2+C3
=C1+C2V[1−C3C1+C2+C3]
Δq=(C1+C2)V[1−C3C1+C2+C3]
Topper's Solved these Questions
Similar Questions
Which of the following quantity does not change due to damping of oscillations?
02:26View SolutionA body of mass 2kg is thrown into space with escape velocity 11.2km/s, the escape velocity for another body of mass 5 kg will be .
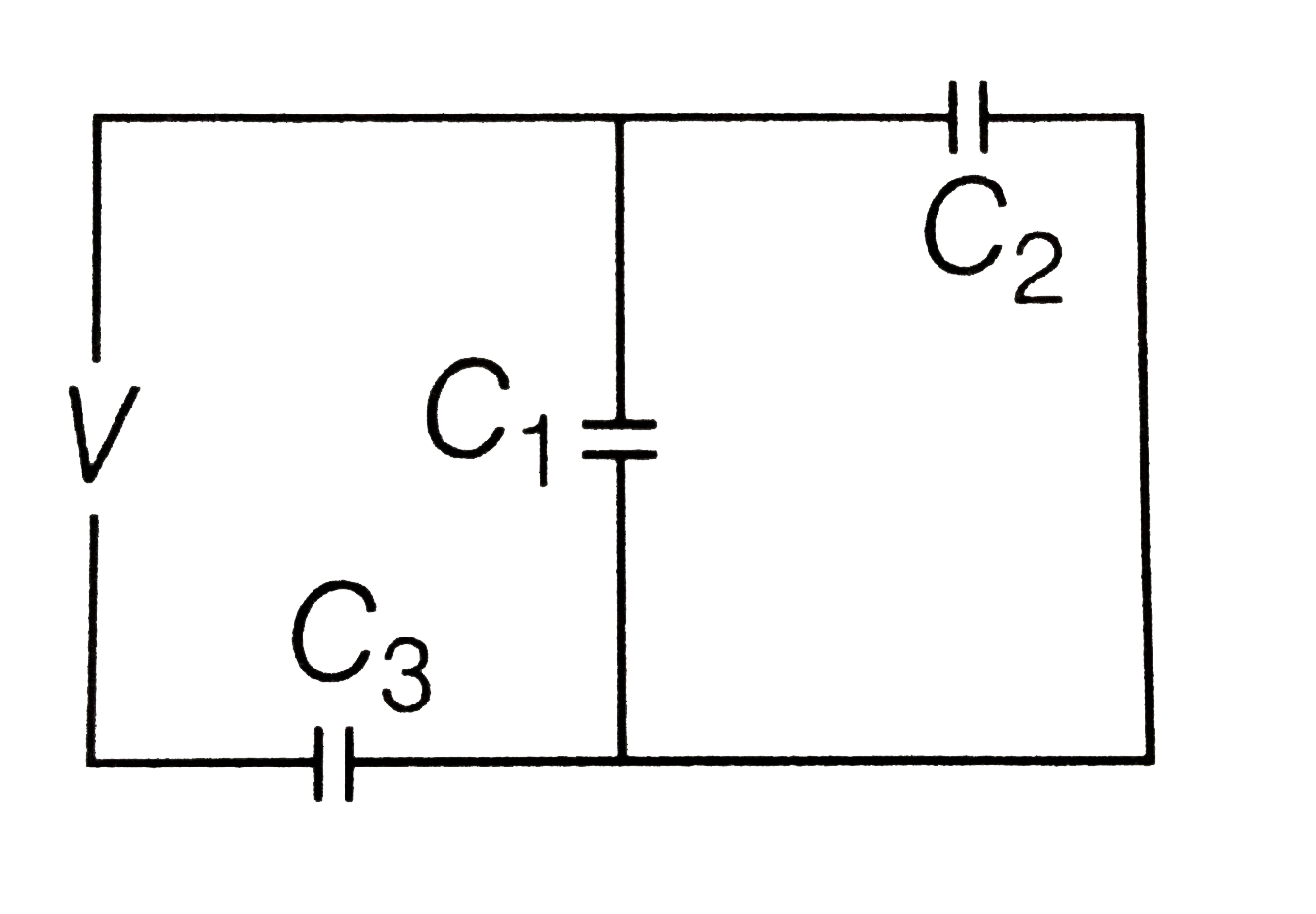
01:46View SolutionThree capacitors C1,C2andC3 are connected to a 6 V battery, as shown in Fig. Find the charges on the three capcitors.
 02:32View Solution
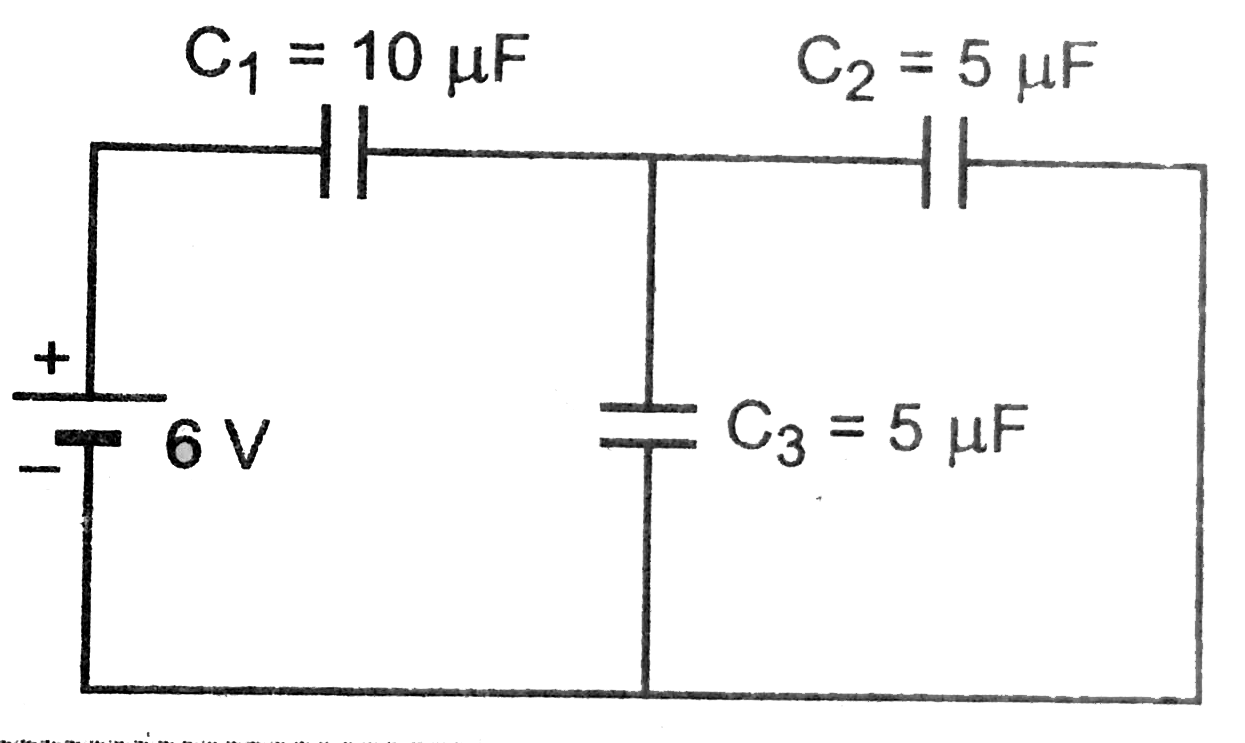
02:32View SolutionTwo capacitors C1 and C2 are connected to a battery of 6V as shown in Fig. Find the charge on each capacitor.
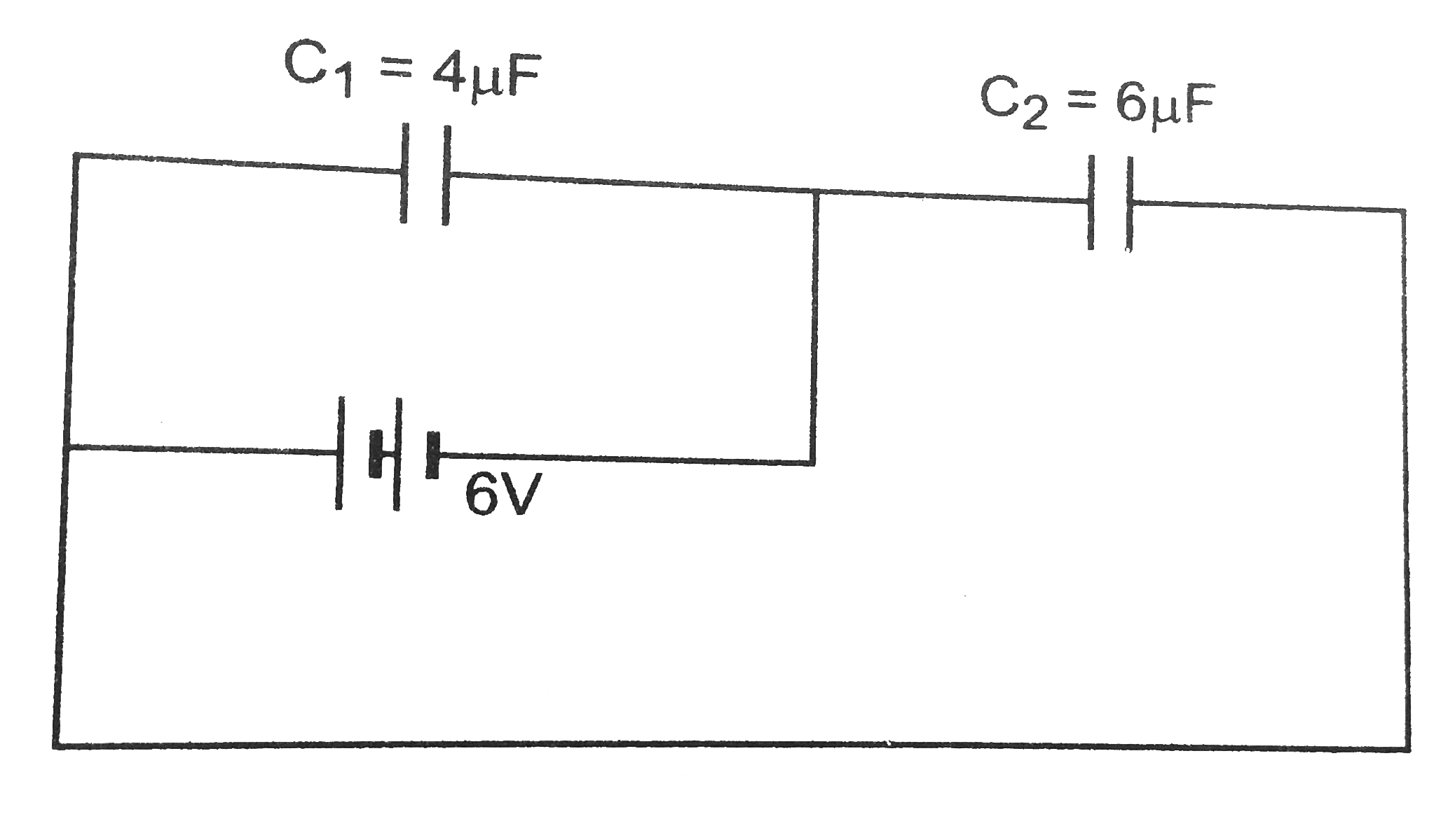 05:16View Solution
05:16View SolutionTwo capacitors C1=3μF and C2=6μF in series, are connected in parallel to a third capacitor C3=4μF. This arrangement is then connected to a battery of e.m.f., =30V, as shown. The energy lost by the battery in charging the capacitors
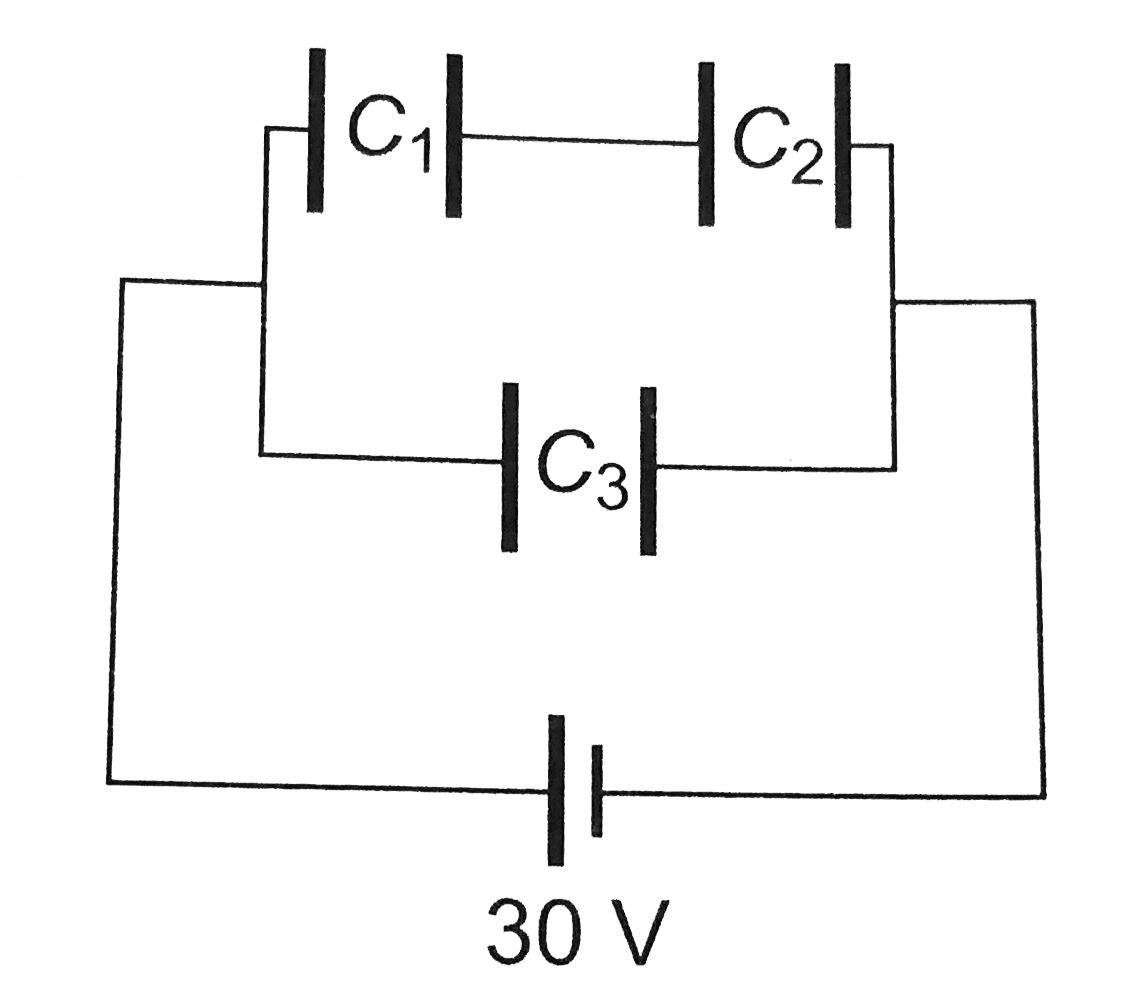 02:05View Solution
02:05View Solutionतीन समरूप संधारित्र C1,C2 तथा C3 जिनकी प्रत्येक की धारिता 6μF है, 12 V की बैटरी से जुड़े हैं जो कि चित्र में प्रदर्शित है । ज्ञात कीजिए : (i) प्रत्येक संधारित्र का आवेश, (ii) परिपथ की समतुल्य धारिता ।
 04:24View Solution
04:24View Solutionतीन आवेशित संधारित्र C1,C2, व C3 श्रेणीक्रम में जुड़े हैं, तुल्यधारिता होगी-
View SolutionA capacitor C1 is charged to a p.d.V. The charging battery is then removed and the capacitor is connected to an uncharged capacitor C2. The final p.d. across the combination is
01:12View SolutionThree capacitors of capacity C1,C2,C3 are connected in series. Their total capacity will be
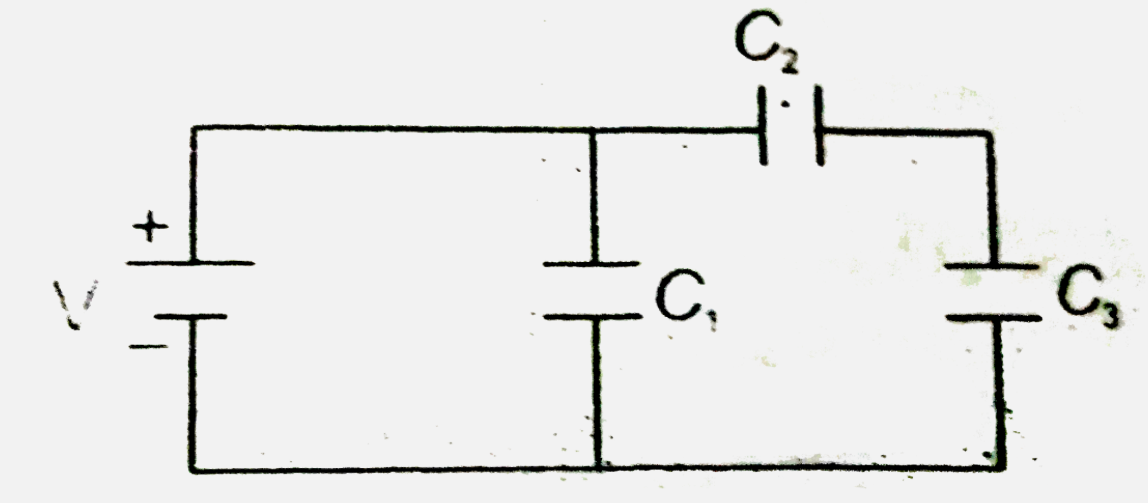
02:09View SolutionThree capacitors C1,C2 and C3 are connected to a battery as shown in the figure . The three capacitors have equal capacitances. Which capacitor stores the most energy ?
 01:57View Solution
01:57View SolutionFind the charge on capacitor C_(3) Given, then C_(1) = C_(2) and C_(3) = C_(4) = 3 C .
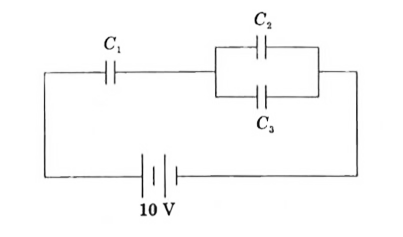
07:29View SolutionThe figure shows a network of three capacitors C1=2μF,C2=6μFandC3=3μF connected across a battery of 10 V. calculate the charge acquired by C1.
 02:12View Solution
02:12View Solution6muF Capacitor with three capacitance C_(1),C_(2) And C_(3) , Are connected to a 12 V battery [Figure 4.60]. (a) charge on each capacitor, (c) Find the energy stored in the capacitor circuit.
05:24View Solutionएक समान्तर प्लेट संधारित्र की प्रत्येक प्लेट का क्षेत्रफल 2 m^ 2 तथा दोनों प्लेटों के मध्य विद्युत क्षेत्र की तीव्रता 100N/C है। प्रत्येक प्लेट पर आवेश कितना है?
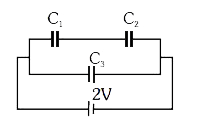
03:45View Solutionसंधारित्र C1=2μ तथा C2=6μF जो श्रेणी क्रम में हैं, को एक तीसरे संधारित्र C3=4μF के साथ समान्तर क्रम में जोड़ा जाता है। इस संयोजन को एक 2 बोल्ट वि. वा. बल वाली बैटरी से जोड़ा जाता है, जैसा कि चित्र में दर्शाया है। इन संधारित्र को आवेशित करने में बैटरी को कितनी ऊर्जा देनी
 03:25View Solution
03:25View Solution

