The area of the region bounded by the curve $y = 2 x - x^{2}$ and the line $y = x$ is

A

$\frac{1}{6}$

B

$\frac{1}{2}$

C

$\frac{1}{3}$

D

$\frac{7}{6}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

The intersection points of given curves are (0,0) and (1,1)
$∴$ Required area $= \int_{0}^{1} [(2 x - x^{2}) - x] d x$
$= \int_{0}^{1} (x - x^{2}) d x = {[\frac{x^{2}}{2} - \frac{x^{3}}{3}]}_{0}^{1}$

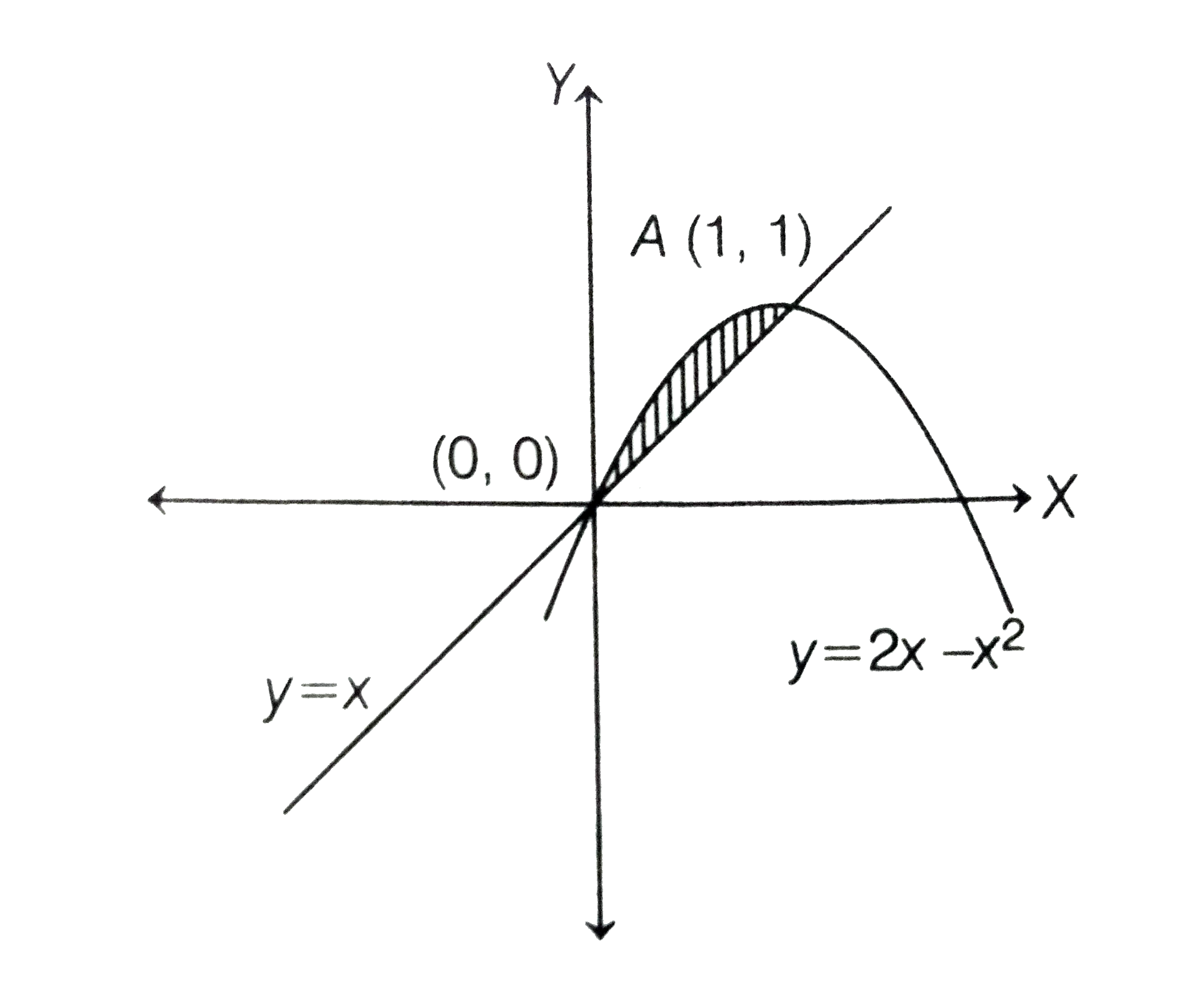
$= \frac{1}{6}$ sq. unit

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The area of the region bounded by the curve $y = x^{2}$ and the line $y = 16$ is :
A $\frac{32}{3}$
B $\frac{256}{3}$
C $\frac{64}{3}$
D $\frac{128}{3}$
Question 2 - Select One
The area bounded by the curve $y = 2 x - x^{2}$ and the line y = - x is
A $\frac{3}{2}$ sq units
B $\frac{9}{3}$ sq units
C $\frac{9}{2}$ sq units
DNone of these
Question 3 - Select One
The area of the region bounded by the curve $y = x + 1$ and the lines $x = 2, x = 3,$ is
A $\frac{7}{2} sq units$
B $\frac{9}{2} sq unit$
C $\frac{11}{2} sq unit$
D $\frac{13}{2} sq units$

MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS-SOLVED PAPER 2019-MCQS

Home
Ask Doubt
Profile