A random variable X has following probability distribution
X=x123456P(X=x)K3K5K7K8KK
Then P(2≤X<5)= . . . .
35
725
2325
2425
The correct Answer is:A
To solve the problem, we need to find the probability P(2≤X<5) for the given probability distribution of the random variable X.
1. Identify the Probability Distribution:
The random variable X has the following probability distribution:
X:1,2,3,4,5,6P(X=x):K,3K,5K,7K,8K,K
2. Set Up the Equation for Total Probability:
The sum of all probabilities must equal 1:
K+3K+5K+7K+8K+K=1
Simplifying this gives:
25K=1
3. Solve for K:
To find K, divide both sides by 25:
K=125
4. Calculate Individual Probabilities:
Now we can calculate the probabilities for each value of X:
P(X=1)=K=125P(X=2)=3K=325P(X=3)=5K=525=15P(X=4)=7K=725P(X=5)=8K=825P(X=6)=K=125
5. Determine the Required Probability:
We need to find P(2≤X<5), which includes X=2,3,4:
P(2≤X<5)=P(X=2)+P(X=3)+P(X=4)
Substituting the values:
P(2≤X<5)=325+525+725
6. Combine the Probabilities:
Adding these fractions:
P(2≤X<5)=3+5+725=1525=35
Final Answer:
Thus, the probability P(2≤X<5) is:
35
The random variable X has the following probability distribution:
X:1,2,3,4,5,6P(X=x):K,3K,5K,7K,8K,K
The sum of all probabilities must equal 1:
K+3K+5K+7K+8K+K=1
Simplifying this gives:
25K=1
To find K, divide both sides by 25:
K=125
Now we can calculate the probabilities for each value of X:
P(X=1)=K=125P(X=2)=3K=325P(X=3)=5K=525=15P(X=4)=7K=725P(X=5)=8K=825P(X=6)=K=125
We need to find P(2≤X<5), which includes X=2,3,4:
P(2≤X<5)=P(X=2)+P(X=3)+P(X=4)
Substituting the values:
P(2≤X<5)=325+525+725
Adding these fractions:
P(2≤X<5)=3+5+725=1525=35
Thus, the probability P(2≤X<5) is:
35
Topper's Solved these Questions
Similar Questions
A random variable X has the following probability distribution find K.

View SolutionA random variable X has the follwing probability distribution:
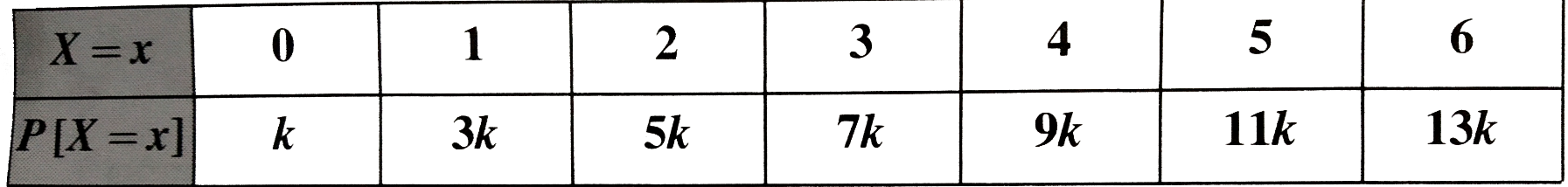
Find k.
View SolutionA random variable X has the following probability distribution: [X=x0123P(X=x)181214k]
View SolutionA random variable X has the following probability distribution:Determine (i) k (ii) P(X<3)(iii) P(X>6) (iv) P(0<X<3)
View SolutionA random variable X has the following probability distribution: X=xi: 1 2 3 4 P(X=xi): k 2k 3k 4k Write the value of P(X≥3) .
View SolutionA random variable X has the following probability distribution:
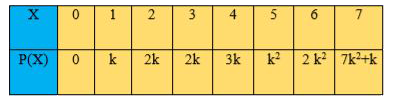
Determine : (i) k (ii) P(X<3) (iii) P(X>4)
View SolutionA random variable X has the following probability distribution: Values of X: −2 −1 0 1 2 3 P(X):0.1 k 0.2 2k 0.3 k
View SolutionA random variable X has the following probability distribution: I 0 1 2 3 4 5 6 7 P (X) 0 K 2K 2K 3K K2 2K2 7K2+K Determine: (i) K (ii) P(X<3) (iii) P(X > 6) (iv) P (0 < X < 3)
View SolutionA random variable X has the following probability distribution :
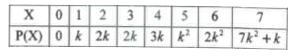
Determine
(i) k (ii) P(X<3)
(iii) P(X>6) (iv) P(0<X<3).
View SolutionA random variable X has the following probability distribution:
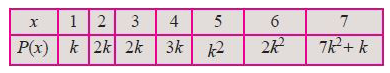
Determine (i)k,(ii)P(X<3),(iii)P(0<X<3),(iv)P(X>4).
View Solution
