If ¯a and ¯b any two non-collinear vectors lying in the same plane, then prove that any vector ¯r coplanar with them can be uniquely expressed as ¯r=t1¯a+t2¯b, where t1andt2 are scalars.
Step by step video & image solution for If bar(a) and bar(b) any two non-collinear vectors lying in the same plane, then prove that any vector bar(r) coplanar with them can be uniquely expressed as bar(r)=t_(1)bar(a)+t_(2)bar(b), where t_(1)andt_(2) are scalars. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MODEL QUESTION PAPER FOR PRACTICE BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MODEL QUESTION PAPER FOR PRACTICE EXERCISE - SECTION-D (Atempt any five of the following)
8 Videos
- MODEL QUESTION PAPER FOR PRACTICE BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MODEL QUESTION PAPER FOR PRACTICE EXERCISE - SECTION-B (Attempt any Eight of the following )
12 Videos
- MATRICESBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MATRICESEXERCISE - MULTIPLE CHOICE QUESTIONS
12 Videos
- PAIR OF STRAIGHT LINES BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PAIR OF STRAIGHT LINES EXERCISE - MULTIPLE CHOICE QUESTIONS
10 Videos
Similar Questions
Knowledge Check
A vector coplanar with the non-collinear vectors ¯aand¯b is
A¯aׯbB¯a+¯bC¯a.¯bD¯a×3¯b
¯a & ¯b are two non- collinear vectors then the value of ⎧⎨⎩¯a|¯a|2−¯b∣∣¯b∣∣2⎫⎬⎭2 is equal to
View Solution¯a and ¯b are non- collinear vectors. If ¯c=(x−2)¯a+¯b and ¯d=(2x+1)¯a−¯b are collinear , then find the value of x
View SolutionIf ¯¯¯Aand ¯¯¯Bare two vectors then A new vector parallel to ¯¯¯Ahaving the magnitude of ¯¯¯Bis
View Solution¯a and ¯b are non-collinear vectors. If ¯c=(x−2)¯a+¯b and ¯d=(2x+1)¯a−¯b are collinear, then find the value of x.
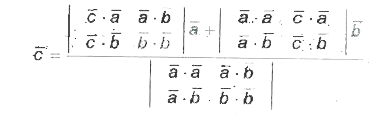
View Solution¯a,¯b,¯c are three coplanar vectors, If ¯a is not parallel to ¯b, Show that
View SolutionIf ¯aand¯b are unit vectors then the vectors (¯a+¯b)×(¯aׯb) is parallel to the vector
View SolutionIf ¯a,¯b,¯c are non-coplanar unit vectors each including the angle of measure 30∘ with the other, then find the volume of tetrahedron whose co-terminal edges are ¯a,¯b,¯c.
View Solution
