Question

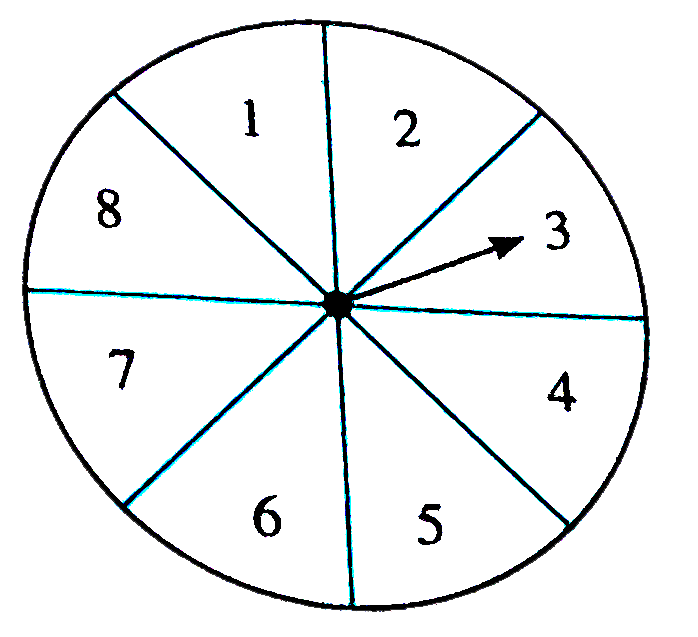
In a game of chance, the spinning arrow rests at one of the numbers $1,2,3,4,5,6,7,8.$ All these are equally likely outcomes. Find the probability of the following events.
(i) the arrow rests at an odd number
(ii) it rests at a prime number
(iii) it rests at a multiple of 2.

Explanation

Here, `S={1,2,3,4,5,6,7,8}" "therefore(S)=8`
(i) Let A be the event that the arrow rests at an odd number.
Then `A={1,3,5,7}" "therefore n(A)=4`
`(A)=(n(A))/(n(S))" "thereforeP(A)=4/8=1/2`
(ii) Let B the event that the arrow rests at a prime number.
Then `B={2,3,5,7}" " thereforen(B)=4`
`P(B)=(n(B))/(n(S))" "thereforeP(B)=4/8=1/2`
(iii) Let C be the event that the arrow rests at a multiple of 2.
Then `C={2,4,6,8}" "thereforen(C)=4`
`P(C)=(n(C))/(n(S))" "P(C)=4/8=1/2.`
In each of the cases the probability is `1/2.`