The following table shows the increase in height of 20 students in a year. Complete the activity to find the median increase in height :
![The following table shows the increase in height of 20 students in a year. Complete the activity to find the median increase in height : Here, (N)/(2)=10,cf=2,f=10,h=15. Median =L+[((N)/(2)-cf)/(f)]xxh](https://d10lpgp6xz60nq.cloudfront.net/physics_images/NVT_21_MAT_P1_X_C06_E04_003_Q01.png)
Here, N2=10,cf=2,f=10,h=15.
Median =L+⎡⎣N2−cff⎤⎦×h ...... (Formula)
=1.5+□−210×1.5
=1.5+□10×1.5=1.5+□=□
The median increase in height is 2.7 cm.
The correct Answer is:10 ; 8 ; 1.2 ; 2.7.
Step by step video & image solution for The following table shows the increase in height of 20 students in a year. Complete the activity to find the median increase in height : Here, (N)/(2)=10,cf=2,f=10,h=15. Median =L+[((N)/(2)-cf)/(f)]xxh" "...... (Formula) =1.5+(square-2)/(10)xx1.5 =1.5+(square)/(10)xx1.5=1.5+square=square The median increase in height is 2.7 cm. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- STATISTICSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - STATISTICSEXERCISE - Assignment 6.4
9 Videos
- STATISTICSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - STATISTICSEXERCISE - EXAMPLES
3 Videos
- STATISTICSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - STATISTICSEXERCISE - Assignment 6.2
5 Videos
- SIMILARITYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - SUBJECTIVE TYPE
20 Videos
- THEOREMS BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - THEOREMS EXERCISE - CIRCLE
15 Videos
Similar Questions
The following table expresses the age of eight students . Find the median age.
S. No.12345678Age (Years)18161411131092
View SolutionComplete the following activity by filing the boxes :
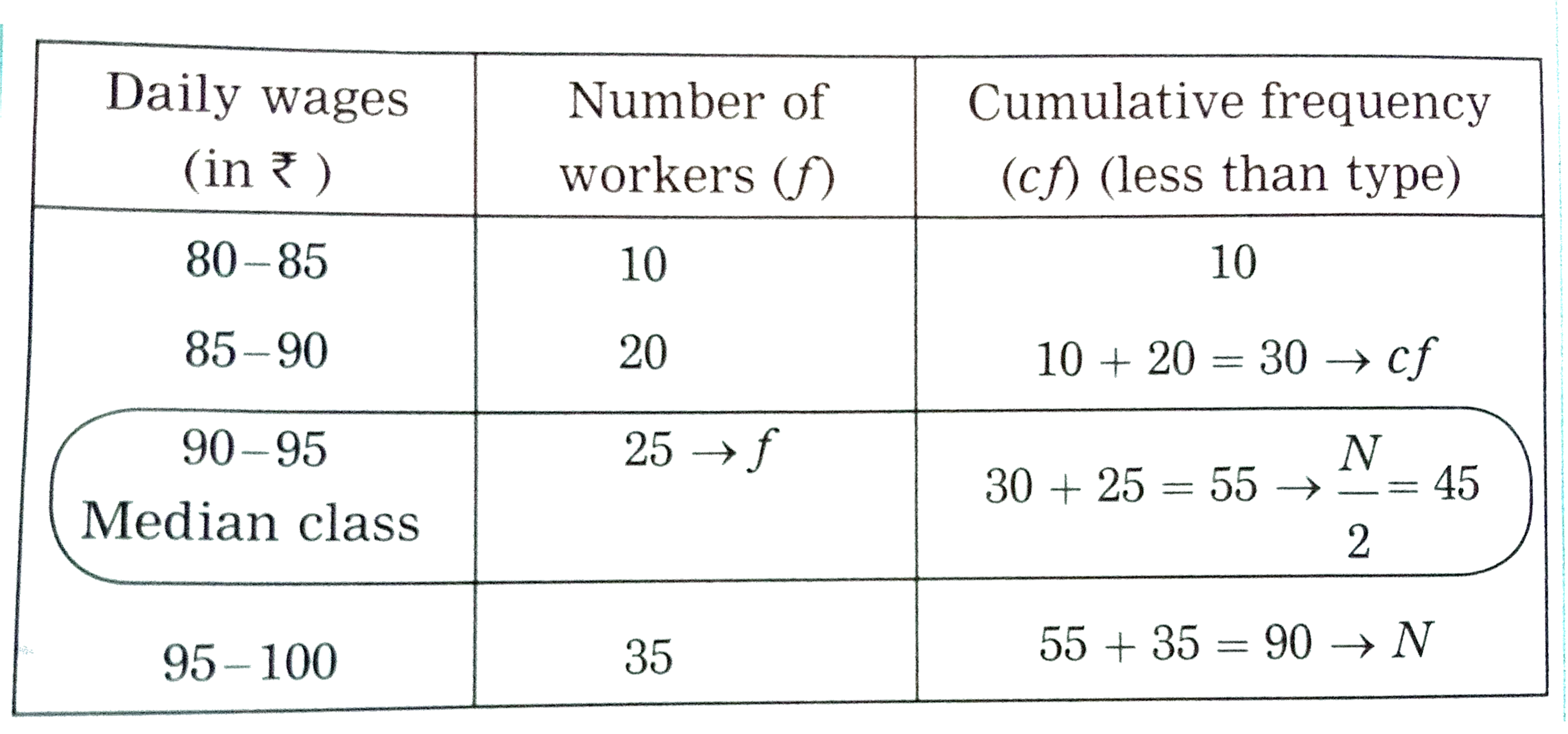
Here, L=90,N2=45,cf=30,f=25,
h = class interval of the median class =□
Median =L+⎡⎣N2−cff⎤⎦×h …(Formula)
=□+□−□25×5 .....(Substituting the values)
=□+□5=□+□=93
View Solution
Knowledge Check
The sides of a square area is increasing at the rate of 0.5 cm/sec. If the side of a square is 10 cm long, then the rate of increase of its perimeter is
A2 cm/secB4 cm/secC3 cm/secD5 cm/sec
The following frequency distribution table shows the number of mango trees in a grove, their yield of mangoes and also the cumulative frequency. Complete the following activity to find the median of the data.
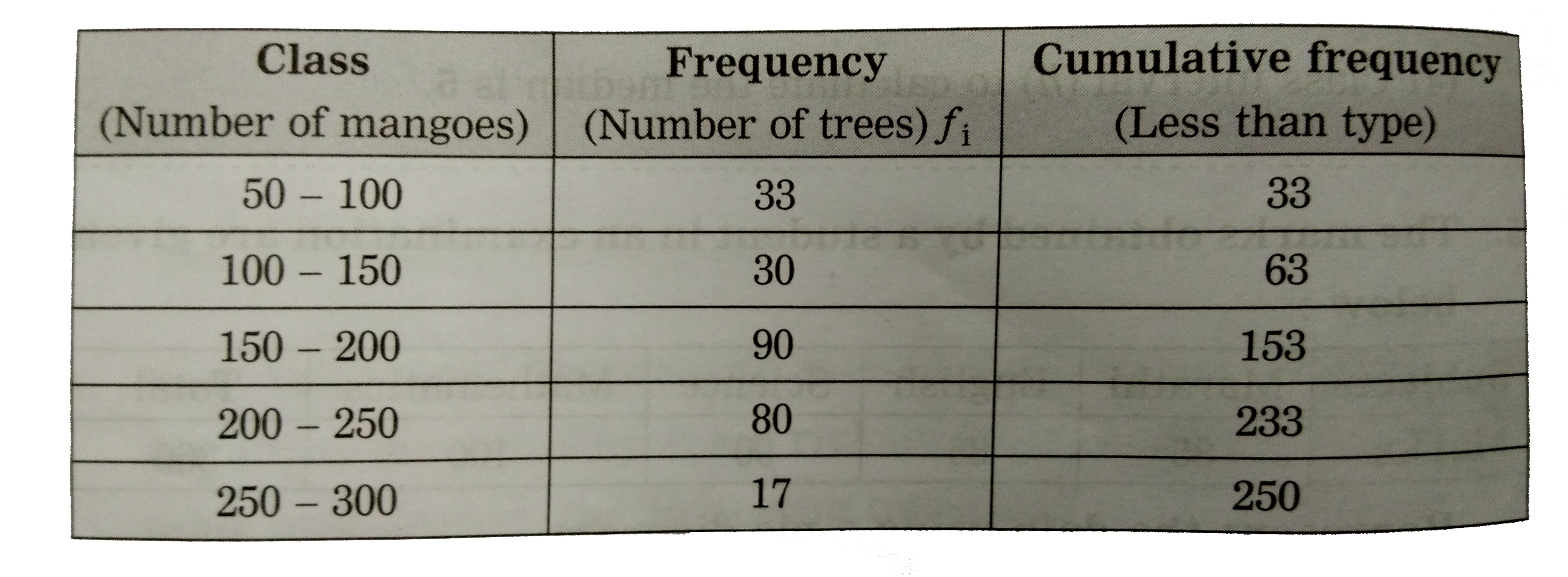
Here, total of frequencies N=250.
N2=2502=125
The cumulative frequency which is just greater than 125 is □
∴ the corresponding class □ is the median class.
L=150,f=90,cf=□,h=50
Median =L+[□f]×h
=150+[□90]×50
=150+□90×50≈184.4
The median of the data is 184.4 mangoes.
View SolutionThe first term and the common difference of an A.P. are 10 and 5 respectively. Complete the following activity to find the sum of the first 30 terms of the A.P.
Sn=n2[□+(n−1)d]
∴S30=302[20+(30−1)×□]
=15[20+□]
=15×165
=□
View Solution(a)□−58=14
(b)□−15=12
(c) 12−□=16
View SolutionThe area of a square is increasing the rate of 0.5 cm2/sec. Find the rate of increase of its perimeter when the side of square is 10 cm long.
View SolutionFor a projectile the ratio of the square of the time of flight to its maximum height is (A) 5:4 (B) 5:2 (C) 4:5 (D) 10:1
View SolutionFollowing ovservations are arrahged in ascending order : 2,5,7,9,10,12,15. Find the median of the data.
View SolutionFind the point of intersection of the medians of a triangle whose vertices are :
(−1,0) , (5,−2) and (8,2).
View Solution
