For a conical pendulum of string length L, its angular speed is proportional to
√L
L
1√L
1L
The correct Answer is:C
To solve the problem of determining how the angular speed of a conical pendulum is related to its string length L, we can follow these steps:
Step 1: Understand the Setup
A conical pendulum consists of a mass (bob) attached to a string of length L that swings in a horizontal circle while the string traces out a cone. The angle between the string and the vertical is denoted as θ.
Step 2: Identify Forces Acting on the Bob
The forces acting on the bob are:
- The gravitational force mg acting downwards.
- The tension T in the string acting along the string.
Step 3: Resolve Forces into Components
We can resolve the tension T into two components:
1. The vertical component: Tcosθ which balances the weight of the bob.
2. The horizontal component: Tsinθ which provides the centripetal force necessary for circular motion.
Step 4: Write the Equations
From the vertical balance of forces, we have:
Tcosθ=mg(1)
From the horizontal motion, the centripetal force is given by:
Tsinθ=mω2r(2)
where r is the horizontal radius of the circular path, which can be expressed as r=Lsinθ.
Step 5: Substitute for r
Substituting r into equation (2):
Tsinθ=mω2(Lsinθ)
This simplifies to:
T=mω2Lsinθsinθ=mω2L(3)
Step 6: Substitute T from Equation (1) into Equation (3)
From equation (1), we can express T:
T=mgcosθ
Setting the two expressions for T equal gives:
mgcosθ=mω2L
Cancelling m from both sides (assuming m≠0):
gcosθ=ω2L
Step 7: Solve for ω
Rearranging gives:
ω2=gLcosθ
Thus, we can express ω as:
ω=√gLcosθ
Step 8: Determine Proportionality
From the equation ω=√gLcosθ, we can see that:
- ω is proportional to 1√L when θ is constant.
Conclusion
Thus, the angular speed ω of a conical pendulum is inversely proportional to the square root of the length L of the string.
Final Answer
The angular speed ω is proportional to 1√L.
A conical pendulum consists of a mass (bob) attached to a string of length L that swings in a horizontal circle while the string traces out a cone. The angle between the string and the vertical is denoted as θ.
The forces acting on the bob are:
- The gravitational force mg acting downwards.
- The tension T in the string acting along the string.
We can resolve the tension T into two components:
1. The vertical component: Tcosθ which balances the weight of the bob.
2. The horizontal component: Tsinθ which provides the centripetal force necessary for circular motion.
From the vertical balance of forces, we have:
Tcosθ=mg(1)
From the horizontal motion, the centripetal force is given by:
Tsinθ=mω2r(2)
where r is the horizontal radius of the circular path, which can be expressed as r=Lsinθ.
Substituting r into equation (2):
Tsinθ=mω2(Lsinθ)
This simplifies to:
T=mω2Lsinθsinθ=mω2L(3)
From equation (1), we can express T:
T=mgcosθ
Setting the two expressions for T equal gives:
mgcosθ=mω2L
Cancelling m from both sides (assuming m≠0):
gcosθ=ω2L
Rearranging gives:
ω2=gLcosθ
Thus, we can express ω as:
ω=√gLcosθ
From the equation ω=√gLcosθ, we can see that:
- ω is proportional to 1√L when θ is constant.
Thus, the angular speed ω of a conical pendulum is inversely proportional to the square root of the length L of the string.
The angular speed ω is proportional to 1√L.
Topper's Solved these Questions
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MULTIPLE CHOICE QUESTIONSEXERCISE - Gravitation8videos
MULTIPLE CHOICE QUESTIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MULTIPLE CHOICE QUESTIONSEXERCISE - Rotational Motion7videos
MULTIPLE CHOICE QUESTIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MODEL QUESTION PAPER FOR PRACTICEEXERCISE - SECTION-D5videos
MODEL QUESTION PAPER FOR PRACTICE
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Semiconductors Devices (Long Answer ( LA) ( 4 marks Each) )3videos
QUESTION BANK 2021
Similar Questions
Conical pendulum|| Examples
View SolutionA coical pendulum consists of a string of length L whose upper end is fixed and another end is tied to a bob. The bob is moving in horizontal circle with constant angular speed ω such that the string makes a constant angle θ with the verticle. calculate time period T0 of revolution of bob in terms of L,g and θ.
View Solution
Knowledge Check
- Question 1 - Select One
A ball of mass M is rotating in a conical pendulum by a string of length L. If radius of circular path is L√2, find the velocity of mass
A√gLB√gL2C√2gLD√gL√2 - Question 2 - Select One
A conical pendulum of length L makes an angle θ with the vertical. The time period will be
 A2π√lcosθgB2π√LgcosθC2π√LtanθγD2π√Lgtanθ
A2π√lcosθgB2π√LgcosθC2π√LtanθγD2π√Lgtanθ - Question 3 - Select One
The period of a conical pendulum in terms of its length (l) , semivertical angle (θ) and acceleration due to gravity (g) is
A12π√lcosθgB12π√lsinθgC2π√lcosθgD4π√ltanθg
Find the period of revolution of a conical simple pendulum whose thread of length l makes an angle α with the vertical (Fig).
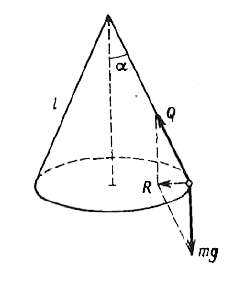 View Solution
View SolutionThe bob of a simple pendulum of length l is given a sharp hit to impart it a horizontal speed of √3gl . When it was at its lowermost position. Find
angle α shown of the string from upside of vertical and speed of the particle when the string becomes slack.
 View Solution
View SolutionA car is moving with constant acceleration a. There is a.conical pendulum of length l in the car which just. touches the roof while performing circular motion (of conical pendulum). The time period of periodic motion is π√xlg, Find x.
'(##CEN_KSR_PHY_JEE_C07_030_Q19##)'View SolutionThe torque required to produce a constant angular acceleration in a body connected to a weightless string of length r, will be proportional to?
View SolutionA heavy particle is tied to the end A of a string of the length1.6 m. Its other end O is fixed. It revolves as a conical pendulum with the string making 60∘ with the horizontal. Then,
View Solution

