Prove theoretically E=−dΦdt.
Consider a rectangular wire loop PQRS of width l, with its plane perpendicular to a uniform magnetic field of induction →B. The loop is being pulled out of the magnetic field at a constant speed v, as shown.
At any instant, let x be the length of the part of the loop in the magnetic field. Then, the magnetic flux through the loop is
Φ=Blx ...(1)
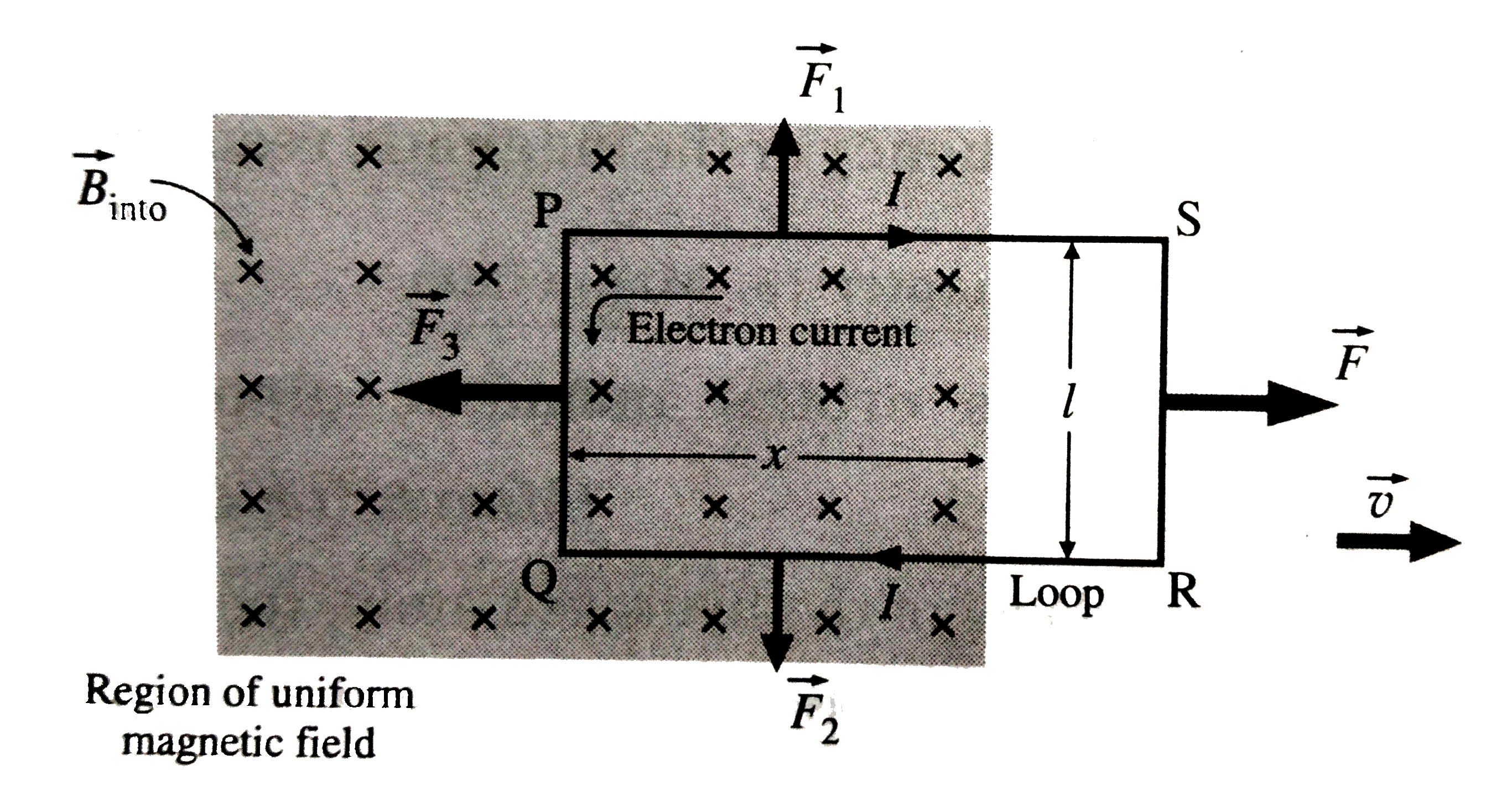
As the loop moves to the right through a distance dx = vdt in time dt, the area of the loop inside the field changes by dA=ldx=lvdt.
And, the change in the magnetic flux dΦ through the loop is
dΦ=Bda=Blvdt ...(2)
Then, the time rate of change of magnetic flux is
dΦdt=Blvdtdt=Blv ...(3)
The changing magnetic flux induces a current I in the clockwise direction, as shown. a current-carrying conductor in a magnetic field experiences a force →F=I→L×→B (in the usual notation), whose direction can be found using Fleming's left hand rule. Accordingly, forces →F1and→F2 on wires PS and QR, respectively, are equal in magnitude (=IxB), opposite in direction and have the same line of action. Hence, they balance each other. There is no force on wire RS as it lies outside the field.
The force →F3 on wire PQ has magnitude F3=IlB and is directed towards the left.
To move the loop with constant velocity →v, an external force →F=−→F3 must be applied.
The work done by the external agent is
dW=Fdx=−IlBdx=−IBdA=−IdΦ [from Eq. (2)] ...(4)
Therefore, the power, i.e., the time rate of doing work, is
P=dWdt=I(−dΦdt) ...(5)
The electric power when an emf E drives a current through a circuit is given by
P=EI ...(6)
In Eq. (4), P is the electric power when a current I is driven through a circuit as a consequence of a change in the magnetic flux through it.
From Eqs. (5) and (6), dW=Pdt=EIdt ...(7)
Therefore, comparing Eq. (4) with Eq. (7), E=−dΦdt ...(8)
Thus, the emf induced in an electric circuit is equal to the negative of the time rate of change of the magnetic flux through the circuit, which is Faraday-Lenz's law.
Topper's Solved these Questions
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - DERIVATIONS-IIEXERCISE - Atoms, Molecules and Nuclei7videos
DERIVATIONS-II
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - DERIVATIONS-IIEXERCISE - Semiconductors1videos
DERIVATIONS-II
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - DERIVATIONS-IIEXERCISE - Magnetism1videos
DERIVATIONS-II
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - DERIVATIONS-IEXERCISE - Assignments9videos
DERIVATIONS-I
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - DISTINGUISH BETWEENEXERCISE - Assingment3videos
DISTINGUISH BETWEEN
Similar Questions
Assertion : Motional induced emf e=Bvl can be derived from the relation e=−dϕdt
Reason : Lenz's law is a consequence of law of conservation of energy.View SolutionIn Figure, DE∣∣AC and DF∣∣AE . Prove that EFBF=ECBE .
View SolutionProve that tanϕ1−cotϕ+cotϕ1−tanϕ=1+tanϕ+cotϕ
View SolutionProve the following A∩(B−A)=ϕ
View SolutionProve that A∩(A∪B)'=ϕ.
View SolutionName the physical quantity with unit same as that of ∣∣∣∈0dϕdt∣∣∣ where ϕe→ electric flux.
View SolutionShow that the dimensions of the displacement current (ε0dΦEdt) are that of an electric current.
View SolutionWrite Maxwell's generalization of Ampere's Circuital Law. Show that in the process of charging a capacitor the , current produced within the plates of the capcitor is I=ε0=dΦEdt where ΦE is the electric flux produced during charging of the capacitor plates.
View SolutionProve that for a null set ϕ,n{P(P(ϕ))}=2.
View SolutionShow that the dimensions of the displacement current (ω0dΦEdt) are that of an electric current.
View Solution

