If for some x∈R the frequency distribution of the marks obtained by 20 statement in a test is :
Marks2357Frequency(x+12)(2x−5)(x2−3x)x then the mean of the marks is :
3.2
3
2.5
2.8
The correct Answer is:A
To find the mean of the marks obtained by 20 students based on the given frequency distribution, we will follow these steps:
Step 1: Set up the frequency distribution
The frequency distribution is given as:
- Marks: 2, 3, 5, 7
- Frequency: (x+12),(2x−5),(x2−3x),x
Step 2: Write the equation for total frequency
The total frequency must equal the total number of students, which is 20. Therefore, we set up the equation:
(x+12)+(2x−5)+(x2−3x)+x=20
Step 3: Simplify the equation
Now, we simplify the left side:
x+1+2x−5+x2−3x+x=20
Combining like terms:
x2+(x+2x−3x+x)+(1−5)=20
This simplifies to:
x2+0x−4=20
Thus, we have:
x2−4=20
Rearranging gives:
x2=24
Step 4: Solve for x
Now we solve for x:
x2−24=0
Factoring gives:
(x−4)(x+4)=0
So, x=4 or x=−4. Since frequency cannot be negative, we take x=4.
Step 5: Substitute x back into the frequency distribution
Now substitute x=4 back into the frequency expressions:
- Frequency for 2: 4+12=5
- Frequency for 3: 2(4)−5=3
- Frequency for 5: 42−3(4)=4
- Frequency for 7: 4=4
So the frequencies are:
- Marks: 2, 3, 5, 7
- Frequencies: 5, 3, 4, 4
Step 6: Calculate the mean
The mean is given by the formula:
Mean=∑(xi⋅fi)∑fi
Calculating ∑fi:
5+3+4+4=16
Calculating ∑(xi⋅fi):
(2⋅5)+(3⋅3)+(5⋅4)+(7⋅4)=10+9+20+28=67
Now, we can find the mean:
Mean=6716=4.1875
Final Answer
The mean of the marks is 4.1875.
---
The frequency distribution is given as:
- Marks: 2, 3, 5, 7
- Frequency: (x+12),(2x−5),(x2−3x),x
The total frequency must equal the total number of students, which is 20. Therefore, we set up the equation:
(x+12)+(2x−5)+(x2−3x)+x=20
Now, we simplify the left side:
x+1+2x−5+x2−3x+x=20
Combining like terms:
x2+(x+2x−3x+x)+(1−5)=20
This simplifies to:
x2+0x−4=20
Thus, we have:
x2−4=20
Rearranging gives:
x2=24
Now we solve for x:
x2−24=0
Factoring gives:
(x−4)(x+4)=0
So, x=4 or x=−4. Since frequency cannot be negative, we take x=4.
Now substitute x=4 back into the frequency expressions:
- Frequency for 2: 4+12=5
- Frequency for 3: 2(4)−5=3
- Frequency for 5: 42−3(4)=4
- Frequency for 7: 4=4
- Marks: 2, 3, 5, 7
- Frequencies: 5, 3, 4, 4
The mean is given by the formula:
Mean=∑(xi⋅fi)∑fi
Calculating ∑fi:
5+3+4+4=16
Calculating ∑(xi⋅fi):
(2⋅5)+(3⋅3)+(5⋅4)+(7⋅4)=10+9+20+28=67
Mean=6716=4.1875
The mean of the marks is 4.1875.
Topper's Solved these Questions
Similar Questions
If for somex∈R, the frequency distribution of the marks obtained by 20 students in a test is:
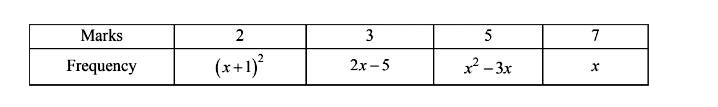
Then the mean of the marks is:View SolutionThere are 60 students in a class. The following is the frequency distribution of marks obtained by the students in a test: Marks:, 0, 1, 2, 3, 4, 5 Frequency , x−2 , x , x2 , (x+1)2 , 2x , x+1. Determine mean and standard deviation of the marks.
View Solution
Knowledge Check
- Question 1 - Select One
If for somes x∈R the frequency distribution of marks obtained by 20 students in a test is:

Then the mean of the marks is:A2.5B3.0C2.8D3.2 - Question 2 - Select One
The frequency distribution of marks obtained by 28 students in a test carrying 40 marks is given below:
Marks:0−1010−2020−3030−40Number of students:6xy6
If the mean of the above data is 20, then the difference between x and y isA3B2C1D0 - Question 3 - Select One
The frequency distribution of discrete data given below, the frequency x against value 0 is missing. Variable x:012345 Frequency f:x204040204 If the mean is 2.5, then the missing frequency x will be
A0B1C3D4
(x2+2x−3)(x2+7x+5)
View SolutionFollowing is the distribution of marks in Statistics obtained by 32 students :
Mid - value51525354555Frequency4610732
Caculate the median marks.View SolutionThe frequency distribution of the marks obtained by 28 students in a test carrying 40 marks is given below :

If the mean of the above data is 20 , then find the difference between x and y .View SolutionThere are 60 students in a class. The following is the frequency distribution of the marks obtained by the students in a test:

Where "p" is positive integer. Determine the mean and standard deviation of the marks.View SolutionIf the mean of the distribution is 2.6, then the value of y is
Variate x12345Frequency f of x45y12View Solution

