Two non ideal batteries are connected in parallel with positive terminals. Consider the following statements:
(A)The equivalent emf is smaller than either of the two emfs.
(B) The equivalent internal resistance is smaller than either of the two internal resistances.
(a)Both A and B are correct
(b)A is correct but B is worng
(c )B is correct but A is worng
(d)Both A and B are worng
The correct Answer is:C
To solve the problem regarding the two non-ideal batteries connected in parallel, we will analyze the given statements step by step.
Step 1: Understand the Configuration
We have two batteries connected in parallel, which means their positive terminals are connected together, and their negative terminals are also connected together. Let’s denote the following:
- ϵ1 = emf of battery 1
- ϵ2 = emf of battery 2
- r1 = internal resistance of battery 1
- r2 = internal resistance of battery 2
Step 2: Equivalent EMF Calculation
For batteries in parallel, the equivalent emf (ϵeq) can be calculated using the formula:
ϵeq=ϵ1/r1+ϵ2/r21/r1+1/r2
This formula takes into account the internal resistances of the batteries.
Step 3: Equivalent Internal Resistance Calculation
The equivalent internal resistance (req) of two resistors in parallel is given by:
req=r1⋅r2r1+r2
Step 4: Analyze Statement (A)
The first statement claims that the equivalent emf is smaller than either of the two emfs.
- Since the formula for ϵeq involves a weighted average of the emfs divided by their respective internal resistances, it is possible for ϵeq to be less than both ϵ1 and ϵ2, especially if one battery has a significantly higher internal resistance compared to the other. However, in general, the equivalent emf can also be greater than one of the individual emfs depending on the values of ϵ1, ϵ2, r1, and r2.
Step 5: Analyze Statement (B)
The second statement claims that the equivalent internal resistance is smaller than either of the two internal resistances.
- From the formula for req, we see that when resistors are in parallel, the equivalent resistance is always less than the smallest individual resistance. Therefore, this statement is true.
Conclusion
- Statement (A) is not necessarily true as the equivalent emf can be greater than one of the individual emfs.
- Statement (B) is true as the equivalent internal resistance is indeed smaller than either of the two internal resistances.
Final Answer
- Statement A: False
- Statement B: True
We have two batteries connected in parallel, which means their positive terminals are connected together, and their negative terminals are also connected together. Let’s denote the following:
- ϵ1 = emf of battery 1
- ϵ2 = emf of battery 2
- r1 = internal resistance of battery 1
- r2 = internal resistance of battery 2
For batteries in parallel, the equivalent emf (ϵeq) can be calculated using the formula:
ϵeq=ϵ1/r1+ϵ2/r21/r1+1/r2
This formula takes into account the internal resistances of the batteries.
The equivalent internal resistance (req) of two resistors in parallel is given by:
req=r1⋅r2r1+r2
The first statement claims that the equivalent emf is smaller than either of the two emfs.
- Since the formula for ϵeq involves a weighted average of the emfs divided by their respective internal resistances, it is possible for ϵeq to be less than both ϵ1 and ϵ2, especially if one battery has a significantly higher internal resistance compared to the other. However, in general, the equivalent emf can also be greater than one of the individual emfs depending on the values of ϵ1, ϵ2, r1, and r2.
The second statement claims that the equivalent internal resistance is smaller than either of the two internal resistances.
- From the formula for req, we see that when resistors are in parallel, the equivalent resistance is always less than the smallest individual resistance. Therefore, this statement is true.
- Statement (A) is not necessarily true as the equivalent emf can be greater than one of the individual emfs.
- Statement (B) is true as the equivalent internal resistance is indeed smaller than either of the two internal resistances.
- Statement A: False
- Statement B: True
Topper's Solved these Questions
- ELECTRIC CURRENT IN CONDUCTORSBOOK - HC VERMACHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - Objective 2
9 Videos
- ELECTRIC CURRENT IN CONDUCTORSBOOK - HC VERMACHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - Exercises
84 Videos
- ELECTRIC CURRENT IN CONDUCTORSBOOK - HC VERMACHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - Short Answer
17 Videos
- DISPERSION AND SPECTRABOOK - HC VERMACHAPTER - DISPERSION AND SPECTRAEXERCISE - Exercises
11 Videos
- ELECTRIC CURRENT THROUGH GASESBOOK - HC VERMACHAPTER - ELECTRIC CURRENT THROUGH GASESEXERCISE - Exercises
23 Videos
Similar Questions
Two non ideal batteries are connected in parallel with positive terminals. Consider the following statements:
(A)The equivalent emf is smaller than either of the two emfs.
(B) The equivalent internal resistance is smaller than either of the two internal resistances.
View Solutionthe nonideal batteries are connected in series. Consider the following statements :
(A)The equivalent emf is larger than either of the two emfs.
(B) the equivalent internal resistances is smaller than either of the two internal resistances.
View Solution
Knowledge Check
Two non-metal batteries are connected, in parallel. Consider the following statements.
(i) The equivalent emf is smaller than either of the two emfs.
(ii) The equivalent internal resistance is smaller than either of the two internal resistances.ABoth (i) and (ii) are correctB(i) is correct but (ii) is wrongC(ii) is correct but (i) is wrongDBoth (i) and (ii) are wrongTwo non-metal batteries are connected, in parallel. Consider the following statements.
(i) The equivalent emf is smaller than either of the two emfs.
(ii) The equivalent internal resistance is smaller than either of the two internal resistances.ABoth (i) and (ii) are correctB(i) is correct but (ii) is wrongC(ii) is correct but (i) is wrongDBoth (i) and (ii) are wrongTwo non-ideal identical batteries are connected in parallel. Consider the following statements
(i) The equivalent e.m.f. is smaller than either of the two e.m.f.s
(ii) The equivalent internal resistance is smaller than either of the two internal resistancesABoth (i) and (ii) are correctB(i) is correct but (ii) is wrongC(ii) is correct but (i) is wrongDBoth (i) and (ii) are wrong
If three cell are connected in parallel then equivalent emf……. and equivalent internal resistance is …..
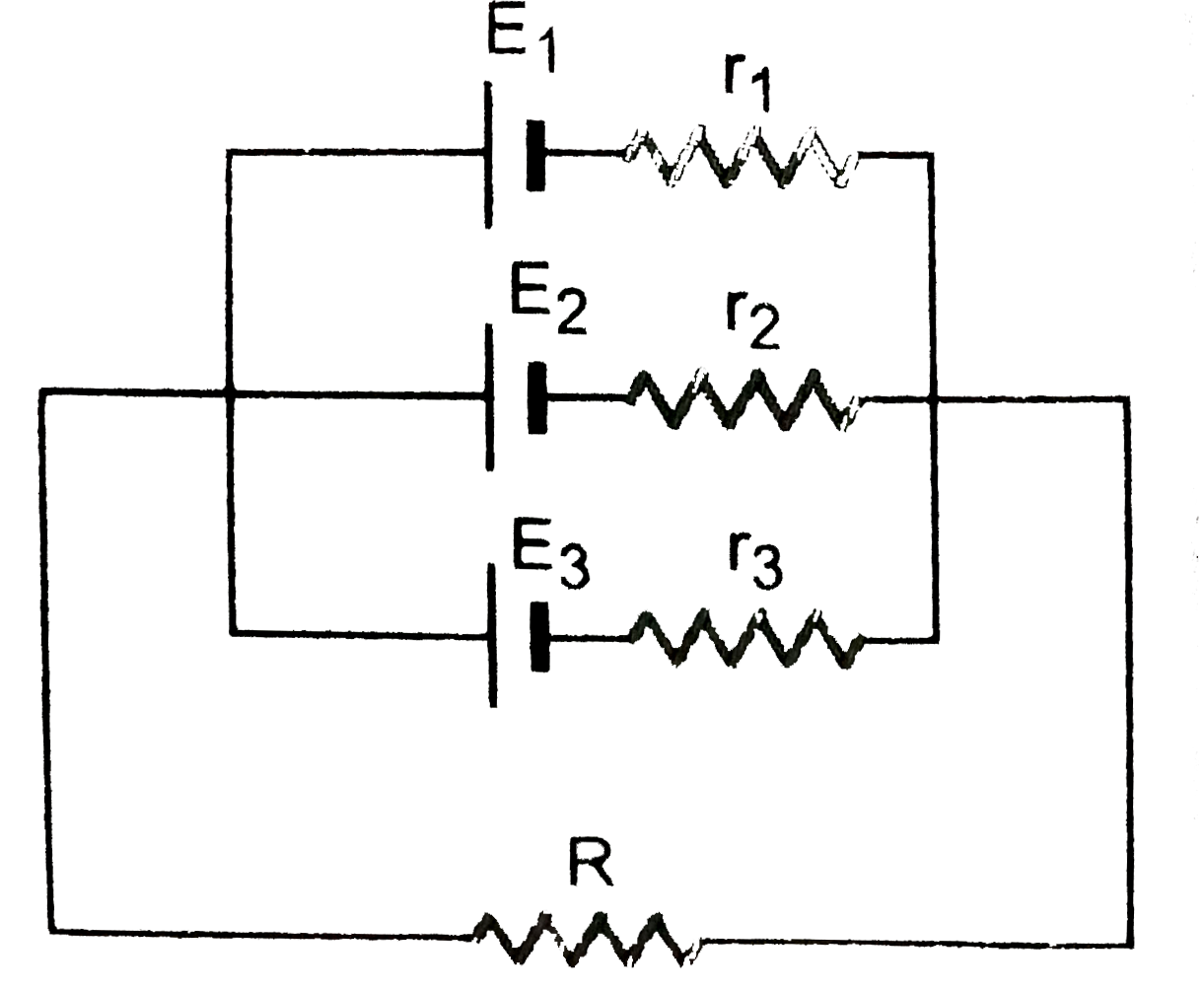
View SolutionIf two equal resistances are connected in parallel the equivalent resistance is halved.
View SolutionFind the equivalent emf and internal resistance of the arrangement shown in figure.
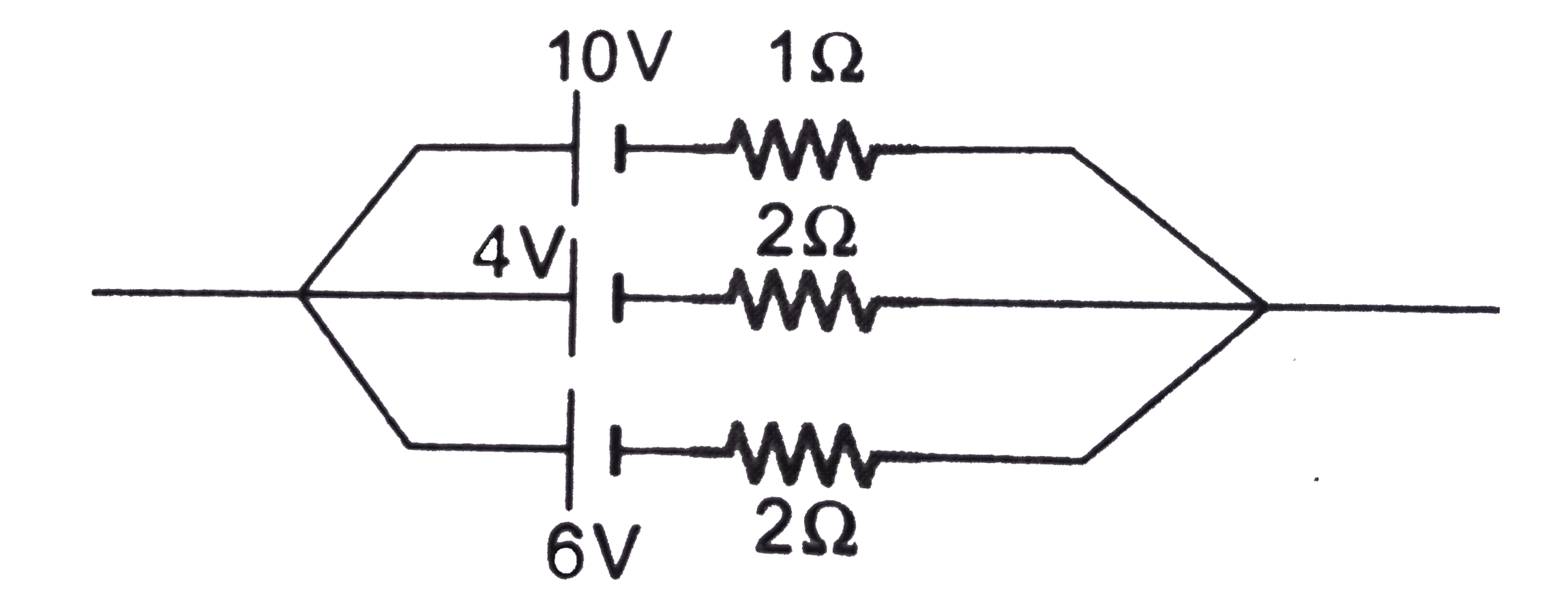
View SolutionAssertion : Two non-ideal batteries are connected in parallel with same polarities on same: side. The equivalent emf is smaller than either of the two emfs.
Reason : Two non-ideal batteries are connected in parallel, the equivalent inter resistance is smaller than either of the two internal resistances.
View SolutionIf n cells each of emf E and internal resistance are connected in parallel, then the total emf and internal resistances will be
View Solution