(a) the magnetic field in a region varies as shown in . Calculate the average induced emf in a conducting loop of area 2.0X10^(-3) m^2 placed perpendicular to the field in each of the10ms intervals shown. (b) In which intervals is the emf not constant? Neglect the behaviour near the ends of 10 ms intervals.
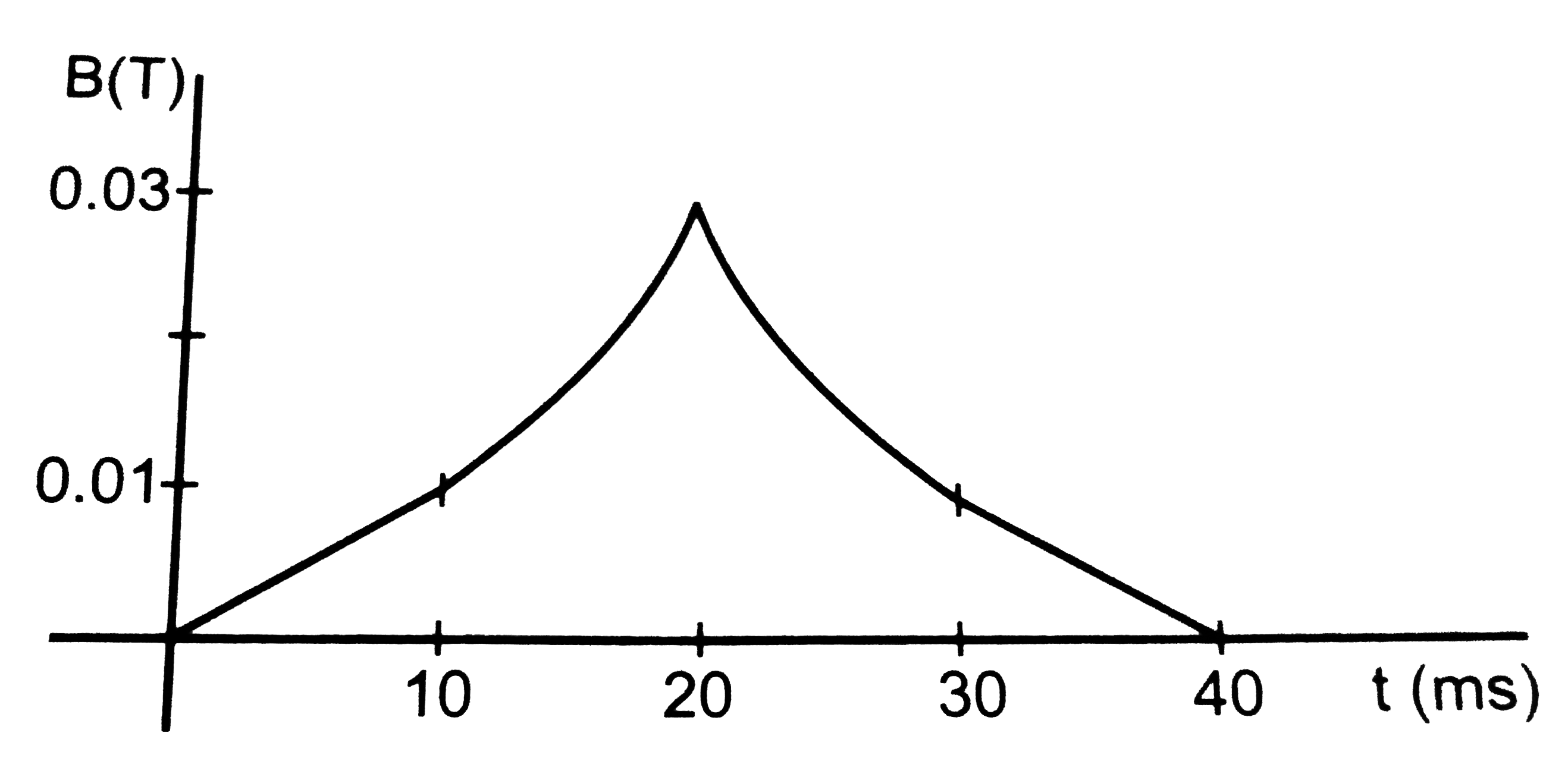
(a)ϕ2=B.A=00.01×2×10−3
=2×10−5[sinceϕ1=0]
dϕ=2×10−5
e=−dϕdt=−2×10−510×10−3
=2×10−3=−2mV
ϕ3=B.A=0.04×2×10−3
=6×10−5
dϕ=4×10−5
e=−dϕdt=−4mV
ϕ4=B.A=0.01×2×10−3
=2×10−5
dϕ=−4×10−5
e=−dϕdt=4mV
ϕ5=B.A=0
dϕ=−2×10−5
e=−dϕdt=−(−2)×10−510×10−3=2mV.
(b)Emfis¬constantiscaseof→10−20msand20ms−30msas−4mVand4m.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective 2
10 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMACHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Exercises
75 Videos
- ELECTROMAGNETIC WAVESBOOK - HC VERMACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercises
9 Videos
Similar Questions
(a) The magnetic field in a region varies as shown in figure. Caculate the average induced emf in a conduction loop of area 10−3m2 placed perpendicular to the field in each of the 10ms intervals shown. (b) In which interval(s) I sthe emf not constant ? Neglect the behaviour near the ends of 10 ms intervals.

View SolutionA wire 88 cm long bent into a circular loop is placed perpendicular to the magnetic field of flux density is increased to 3.0 wbm−2. Calculate the value of the emf induced .
View Solution
Knowledge Check
A conducting square loop is placed in a magnetic field B with its plane perpendicular to the field. Now the sides of the loop start shrinking at a constant rate α. the induced emf in the loop at an instant when its side is a is
A2aαBBa2αBC2a2αBDaαBa conducting loop of radius R is precent in a uniform magnetic-field B perpendicular the plane of the ring. If radius R varies as a function of time 't', as R0+t. The e.m.f induced in the loop is
 A2π(R0+t)B clockwiseBπ(R0+t)B clockwiseC2π(R0+t)B anticlockwiseDzero
A2π(R0+t)B clockwiseBπ(R0+t)B clockwiseC2π(R0+t)B anticlockwiseDzeroA conducting loop of radius R is present in a uniform magnetic field B perpendicular to the plane of the ring. If radius R varies as a function of time t, as R=R0+t. The emf induced in the loop is
 A2π(R0+t)B clockwiseBπ(R0+t)B clockwiseC2π(R0+t)B anticlockwiseDzero
A2π(R0+t)B clockwiseBπ(R0+t)B clockwiseC2π(R0+t)B anticlockwiseDzero
A coil having an area 2m2 is placed in a magnetic field which changes from 1wb/m2 n interval of 2s. The e.m.f. induced in the coil is:
View SolutionA conducting wire of length 10 cm is moving perpendicular in a region of magnetic field 1 tesla with a speed of 10ms−1. Calculate the induced emf in the conductor.
View SolutionA rectangular copper coil is placed in a uniform magnetic field of induction 40 mT with its plane perpendicular to the field. The area of the coil is shrinking at a constant rate of 0.5m2s−1. The emf induced in the coil is
View SolutionA single conducting loop with an area of 2.0 m2 rotates in a uniform magnetic field so that the induced emf has a sinusoidal time dependence as shown.
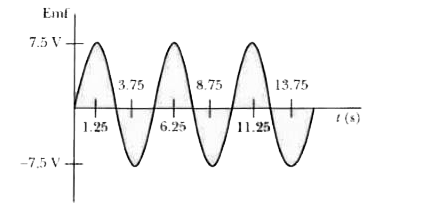
What is the period of the induced current?
View SolutionThe figure shows a uniform, 3.0 T magnetic field that is normal to the plane of a conducting, circular loop with a resistance of 1.5 Ω and a radius of 0.024 m. The magnetic field is directed out of the paper as shown . Note: The area of the non-circular portion of the wire is considered negligible compared to that of the circular loop.
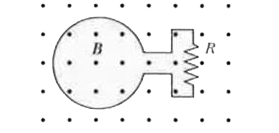
If the magnetic field is held constant at 3.0 T and the loop is pulled out of the region that contains the field in 0.2 s, what is the magnitude of the average induced emf in the loop?
View Solution
