A circular coil of one turn of radiius 5.0 cm is rotated about a diameter with a constatn angular speed of 80 revolutions per minute. A uniform magnetic field B =0.010 T exists in a directon perpendicular to the axis of rotation. Find (a) the maximum emf induced, (b) the average emf induced in the coil over a long period and (c ) the average of the squares of emf induced over a long period.
Since emf=(d phi)/(dt)=(dB.A cos theta)/(dt)<br>=-BA omega. Sin theta<br>(dq//dt= the rate of change its direction every time, So for the average emf=0.<br>=BA omega<br>=0.010xx25xx10^(-4)xx80xx(2 pixxpi)/(6))<br>=0.66xx10^(-3)=6.66xx10^(-4) volt.<br>(b) Since the induced emf changes its direction every time.so for the average emf.=0.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises
89 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMACHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Exercises
75 Videos
- ELECTROMAGNETIC WAVESBOOK - HC VERMACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercises
9 Videos
Similar Questions
A circular coil of one turn of radius 5.0cm is rotated about a diameter with a constant angular speed of 80 revolutions per minute. A uniform magnetic field B=0.0IT exists in a direction perpendicular to the axis of rotation, the maximum emf induced, the average emf induced in the coil over a long period and the average of the squares of emf induced over a long period is
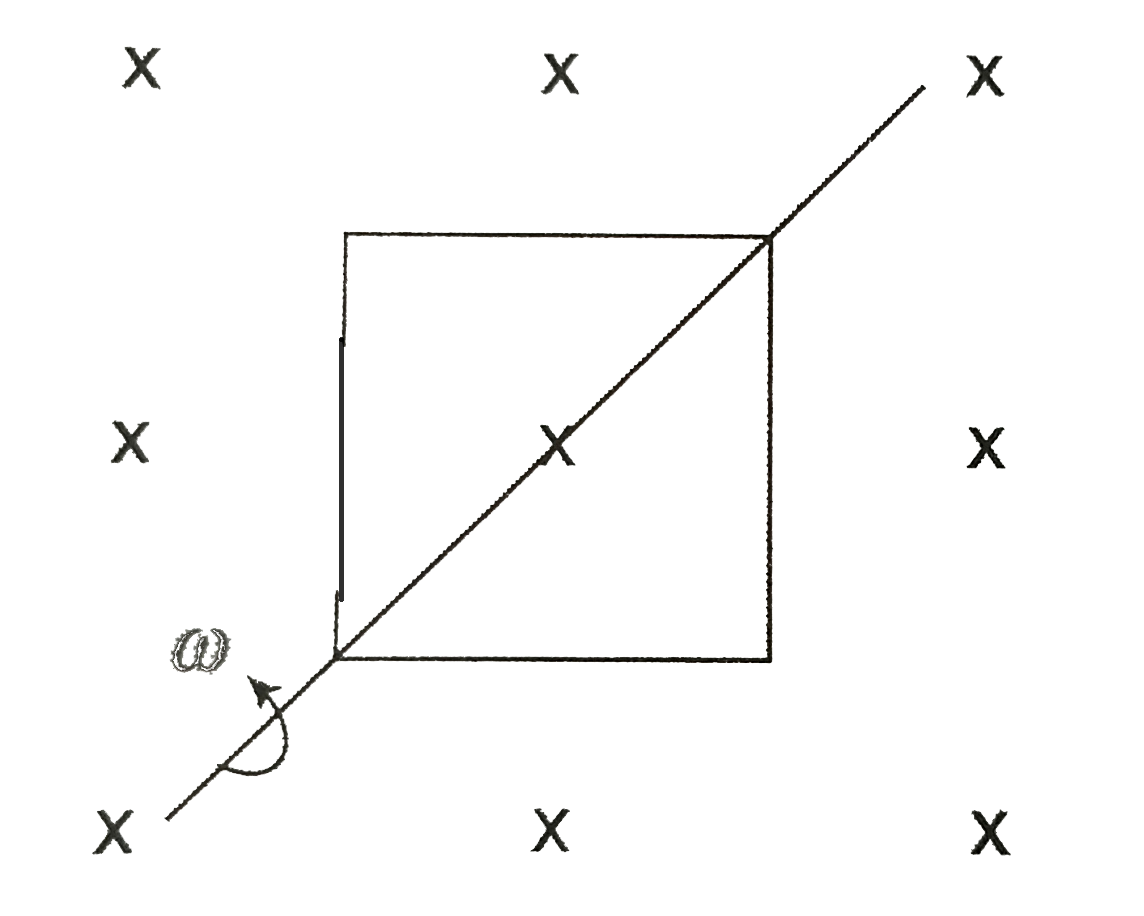
View SolutionA square loop of edge a having N turns is rotated with a uniform angular velocity ω about one of its diagonals which is kept fixed in a horizontal position. A uniform magnetic field B exists in the vertical direction. Find
(a) the emf induced ion the coil as a function of time t
(b) the maximum emf induced
(c) the average emf induced in the loop over a long period (d) the average of the squares of emf induced over a long period
(e) if resistance of loop is R , amount of change flow in time t=0 to t=2T
(f) heat produced in time t=0 to =4T
View Solution
Knowledge Check
A circular coil of one turn of radius 5.0cm is rotated about a diameter with a constant angular speed of 80 revolutions per minute. A uniform magnetic field B=0.0IT exists in a direction perpendicular to the axis of rotation, the maximum emf induced, the average emf induced in the coil over a long period and the average of the squares of emf induced over a long period is
A6.4×10−4V,zero,2.2×10−7V2B6.6×10−4V,zero,2.0×10−7V2C6.8×10−4V,zero,2.5×10−7V2D6.4×10−4V,zero,2.0×10−6V2A coil of 50 turns, each of area 0.12 m2 is rotated at a constant speed of 600 revolutioins per minute in a uniform magnetic field of induction 0.02 T about an axis in the plane of the coil and perpendicular to the direction of the field. The maximum emf induced in a coil is
A7.536 VB0.75 VC0.075 VD0.0075 VAn emf induced in a coil rotating in a uniform magnetic field is given by
Ae=e0sinωtBe0=esinωtCe=sinωtDe=e0sinω
A circular coil of radius 8 cm and 20 turns rotates about its vertical diameter with an angular speed of 50s−1 in a uniform horizontal magnetic field of magnitude 3×10−2T. Find the maximum and average value of the emf induced in the coil.
View SolutionA rectangular coil of area 'A', having number of turns N, is rotated at 'f' revolutions per second in a uniform magnetic field B, the field being perpendicular to the coil. Prove that the maximum emf induced in the coil is 2πfNBA.
View SolutionA square loop of edge b having M turns is rotated with a uniform angular velocity ω about one of its diagonals which is kept fixed in a horizontal position. A uniform magnetic field B0 exists in the vertical direction.
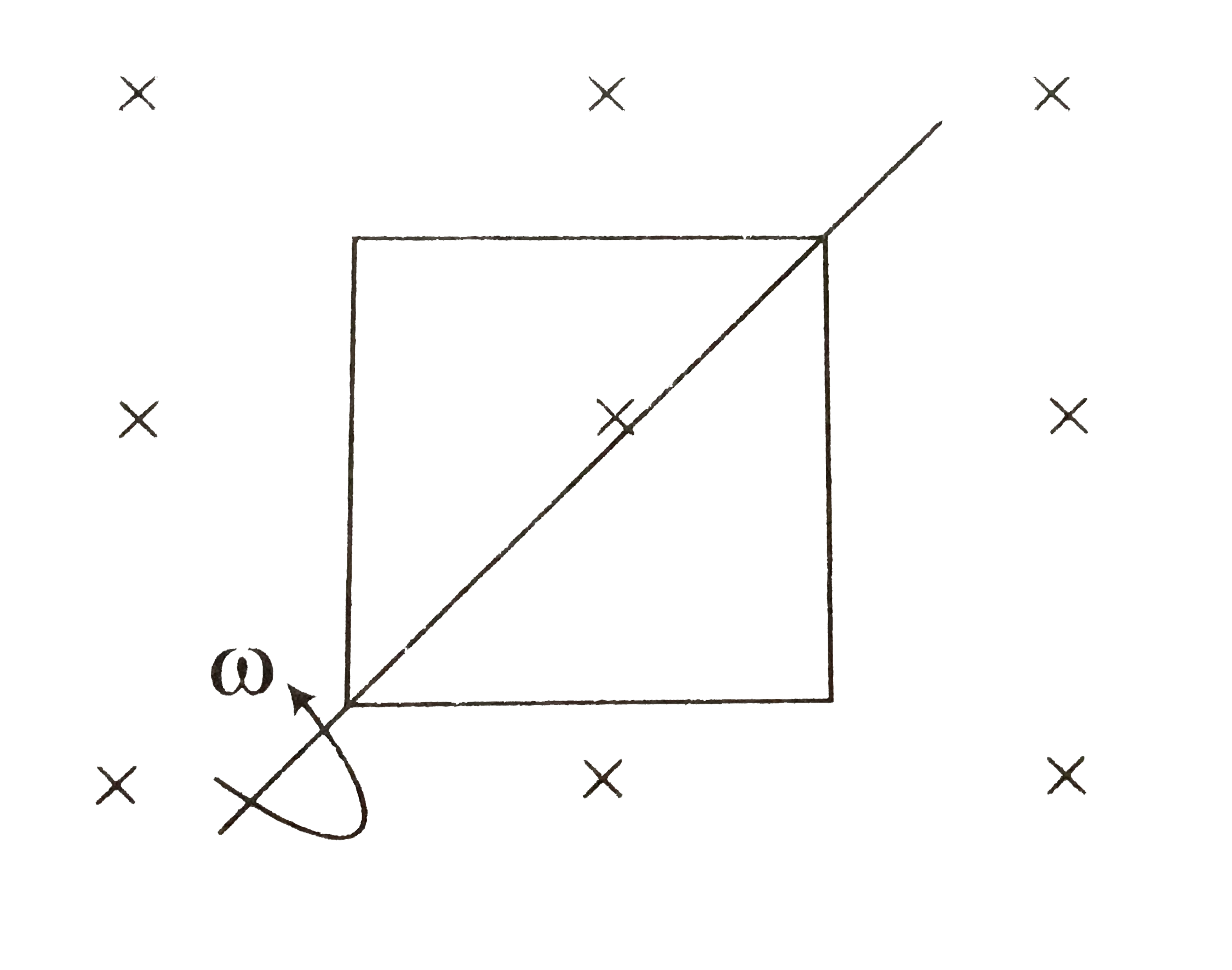
Find (i) the emf induced in the coil as a function of time t.
(ii) the maximum emf induced.
(iii) the average emf induced in the loop over a long period.
(iv) if resistance of loop is R, amount of charge flown in time t = 0 to t = 2T.
(v) heat produced in time t = 0 to t = 2T.
View SolutionA square loop of edge b having M turns is rotated with a uniform angular velocity ω about one of its diagonals which is kept fixed in a horizontal position. A uniform magnetic field B0 exists in the vertical direction.

Find (i) the emf induced in the coil as a function of time t.
(ii) the maximum emf induced.
(iii) the average emf induced in the loop over a long period.
(iv) if resistance of loop is R, amount of charge flown in time t = 0 to t = 2T.
(v) heat produced in time t = 0 to t = 2T.
View SolutionA 100 turn coil of area 0.1m2 rotates at half a revolution per second. It is placed in a magnetic field of 0.01 T perpendicular to the axis of rotation of the coil. Calculate the maximum voltage generated in the coil.
View Solution
