A rectangular coil of area 'A', having number of turns N, is rotated at 'f' revolutions per second in a uniform magnetic field B, the field being perpendicular to the coil. Prove that the maximum emf induced in the coil is $2 π f N B A .$

Text Solution

Verified by Experts

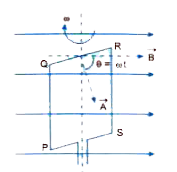
Consider a rectangular coil PQRS of N turns, each of area A, held in a uniform magnetic field $\to B$ . Let the coil be rotated at a steady angular velocity w about its own axis.
Let at any instant t, normal to the area (i.e., the area vector $\to A$ ) subtends an angle $θ = ω t$ from direction of magnetic field $\to B$ . Then, at that moment, the magnetic flux linked with the coil is
$ϕ_{B} = N \to B . \to A = N B A cos θ = N B A cos ω t$
$∴$ Induced emf $ɛ = - (d ϕ_{B}) d t = - \frac{d}{d t} (N B A cos ω t)$
$= - N B A \frac{d}{d t} (cos ω t) = N B A ω t$
where $ɛ_{0} = N B A ω$ = maximum value of induced emf.
If the coil be rotating at .f. revolutions per second, then $ω = 2 π f$ and the maximum induced emf
$ɛ_{0} = N B A ω = 2 π f N B A$

Updated on:21/07/2023

A boy peddles a stationary bicycle the pedals of the bicycle are attached to a 200 turn coil of area $0.10 m^{2}$ . The coil rotates at half a revolution per second and it is placed in a uniform magnetic field of 0.02 T perpendicular to the axis of rotation of the coil. The maximum voltage generated in the coil is
A $1.26 V$
B $2.16 V$
C $3.24 V$
D $4.12 V$
A boy peddles a stationary bicycle the pedals of the bicycle are attached to a 200 turn coil of area $0.10 m^{2}$ . The coil rotates at half a revolution per second and it is placed in a uniform magnetic field of 0.02 T perpendicular to the axis of rotation of the coil. The maximum voltage generated in the coil is
A $1.26 V$
B $2.16 V$
C $3.24 V$
D $4.12 V$
A rectangular coil of area $\to A$ and number of turns n is placed in a uniform magnetic field vecB. If the coil carries a current I, then the torque acting on the coil is given by
A $n i (\to A . \to B)$
B $\frac{1}{n} (i \to A \times \to B)$
C $\frac{1}{n} (i \to A . \to B)$
D $n i (\to A \times \to B)$