Figure is the plot of the stopping potential versus the frequency of the light used in an experiment on photoelectric effect. Find (a) the ratio h/e and (b) the work function.

Text Solution

Verified by Experts

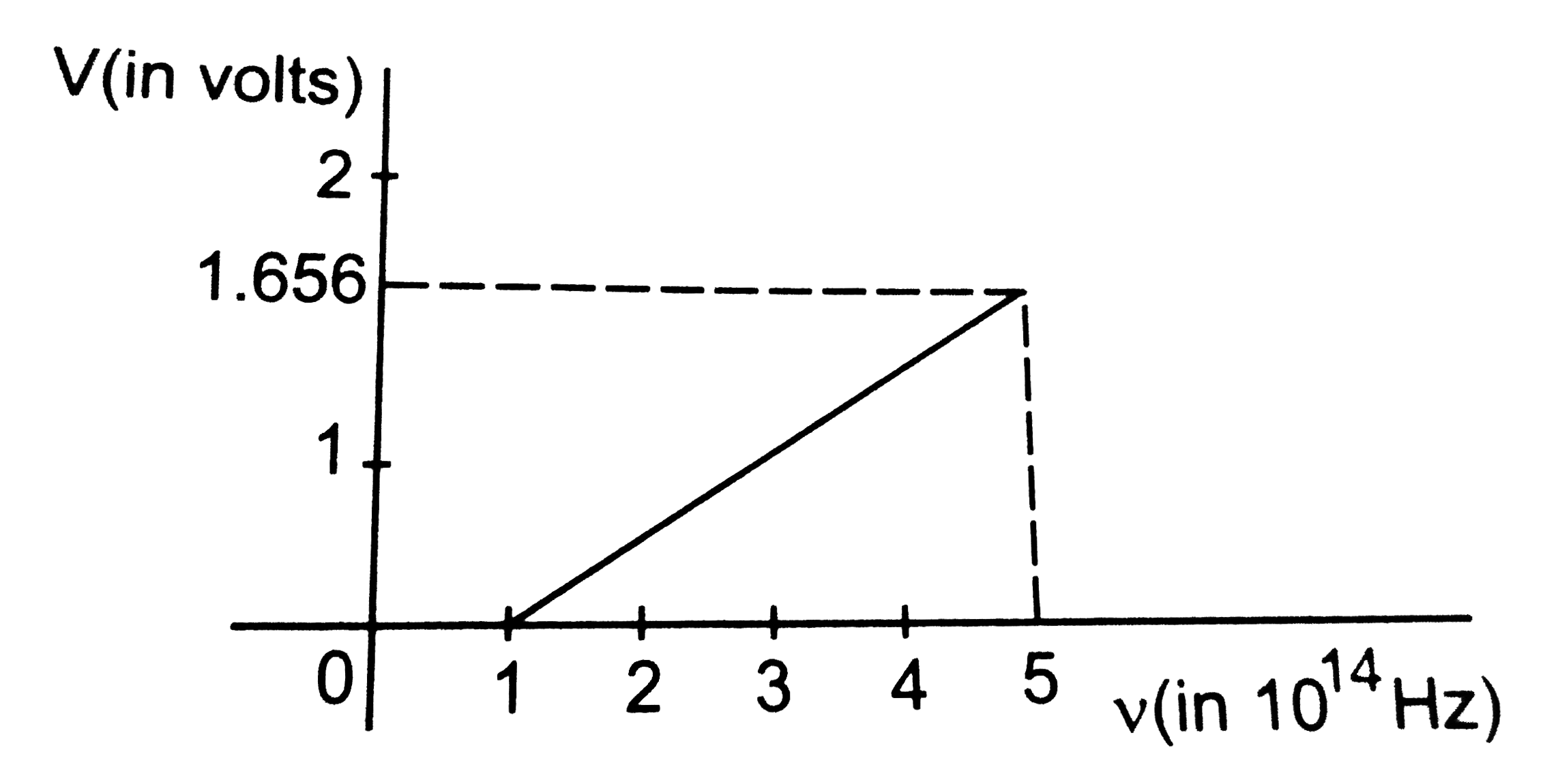
We have to take two cases
Case - I $v_{0} = 1.656$ $v = 5 \times 10^{14} H z$
Case -II $v_{0} = 0$
$v = 1 \times 10^{14} H z$
(b) We know $e v_{0} = h v - W_{0}$
$1.656 e = h \times 5 \times 10^{14} - W_{0}$
$0 = 5 h \times 10^{14} - 5 W_{0}$
$1.6556 e = 4 W_{0}$
$\Rightarrow W_{0} = \frac{1.656}{4} e V$
$= 0.414 e V$
(a) Putting the value of W_0 in equation (2)
$\Rightarrow 5 W_{0} = 5 h \times 10^{14}$
$\Rightarrow 5 \times 0.414 = 5 \times h \times 10^{14}$
$\Rightarrow h = 4.414 \times 10^{- 15} e V - s$

|

Updated on:21/07/2023

Class 12 PHYSICS DUAL NATURE OF RADIATION AND MATTER

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One

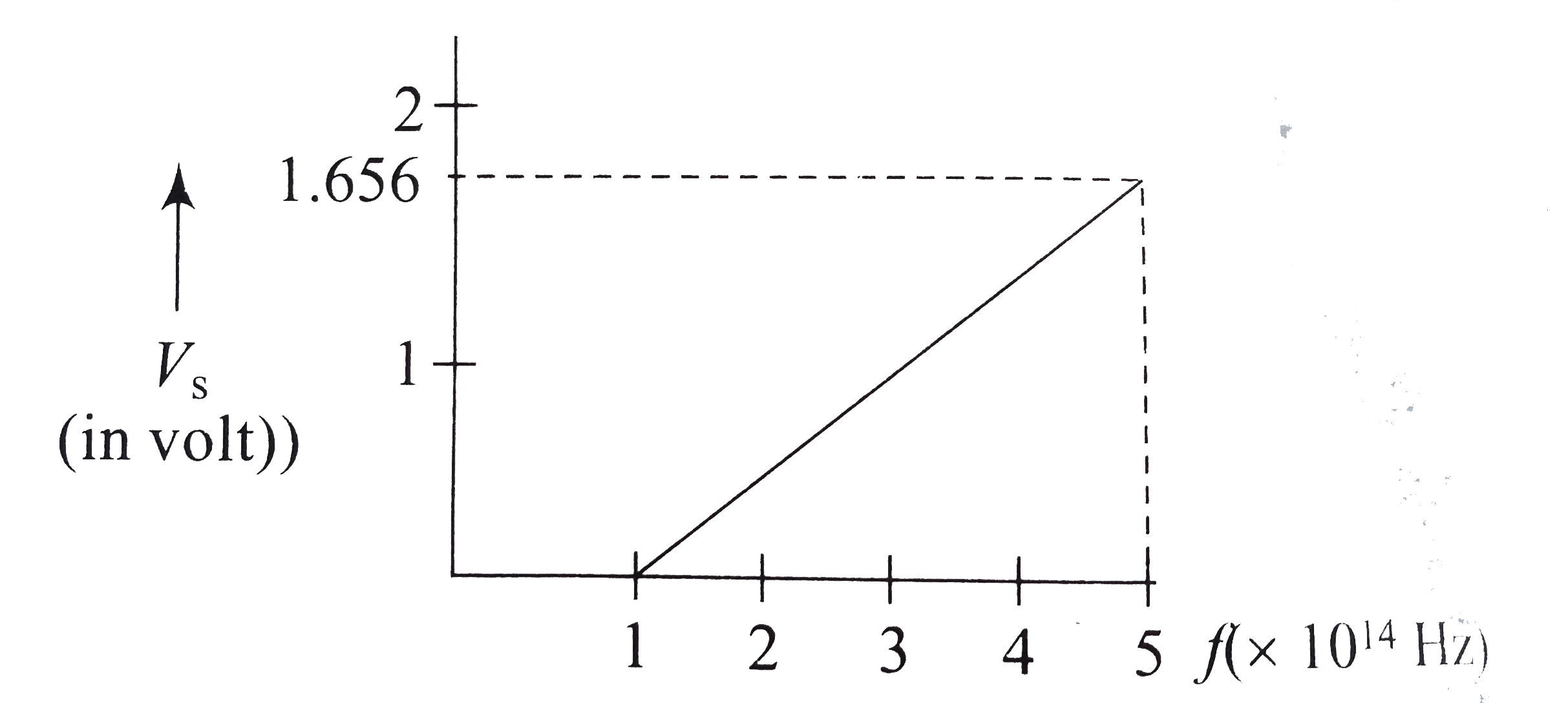
Figure shows the plot of the stopping potential versus the frequency of the light used in an experiment on photoelectric effect. The ratio $\frac{h}{e}$ is
A $10^{- 15} V s^{- 15} V s$
B $2 \times 10^{- 15} V s$
C $3 \times 10^{- 15} V s$
D $4.14 \times 10^{- 15} V s$
Question 2 - Select One
A non-monochromatic light is used in an experiment on photoelectric effect. The stopping potential
Ais related to the mean wavelength
Bis related to the longest wavelength
Cis related to the shortest wavelength
Dis not related to the wavelength
Question 3 - Select One
Graph between stopping potential and frequency of light as shown find the work function
A4.01 ev
B2.07 ev
C5.01 ev
D2.09 ev

HC VERMA-PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITY-Exercise

Home
Ask Doubt
Profile