A horizontal cesium plate is moved vertically downward at a constant speed v in a room full of radiation of wavelength 250nm and above. What should be the minimum value of v so that the vertically upward component of velocity is nonpositive for each photoelectron?
When λ=250nm
Energy of Photon
=hcλ=1240250=4.96eV
∴K.E.=hcλ−W=4.96−1.9eV
=3.06eV
Velocity to be non positive for each photo electron . The minimum value of velocity of plate should be equal to velocity of photo electron
∴Velocityofpho→e≤ctron
=(√2KEM)=(√2×3.06×1.6×10−199.1×10−31)
=1.04×106msec.
Topper's Solved these Questions
- PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYBook:HC VERMAChapter:PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYExercise:Exercise
Explore 2 Videos
- PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYBook:HC VERMAChapter:PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYExercise:Objective 2
Explore 7 Videos
- PERMANENT MAGNETSBook:HC VERMAChapter:PERMANENT MAGNETSExercise:Exercises
Explore 25 Videos
- PHOTOMETRYBook:HC VERMAChapter:PHOTOMETRYExercise:Exercises
Explore 16 Videos
Similar Questions
A horizontal photoelectric plate whose work function is 1.9 eV is moved vertically downward at a constant speed v in a room full of radiation of wavelength 250 nm and above. What should be the minimum value of v so that the vertically upward component of velocity of ejected photoelectrons is non-positive?
View SolutionA particle of mass m is kept on the top of a smooth sphere of radius LR. It is given a sharp impulse whichimparts it a horizontal speed v. a. find thenormal force between the sphere and the particle just after the impulse. B. What should be the minimum value of v for which the particle does not slip on the sphere? c. Assuming the velocity v to be half the minimum calculated in part, d. find teh angle made by the radius through the particle with the vertical when it leaves the sphere.

View Solution
Knowledge Check
A bucket full of water is tied at the end of rope of length 1.6 m. It is rotated in vertical circle with constant speed around the other end . What should be the minimum speed of bucket so that water does not spill out at the highest position of the water circle?
A16m/sB4m/sC8m/sD2m/s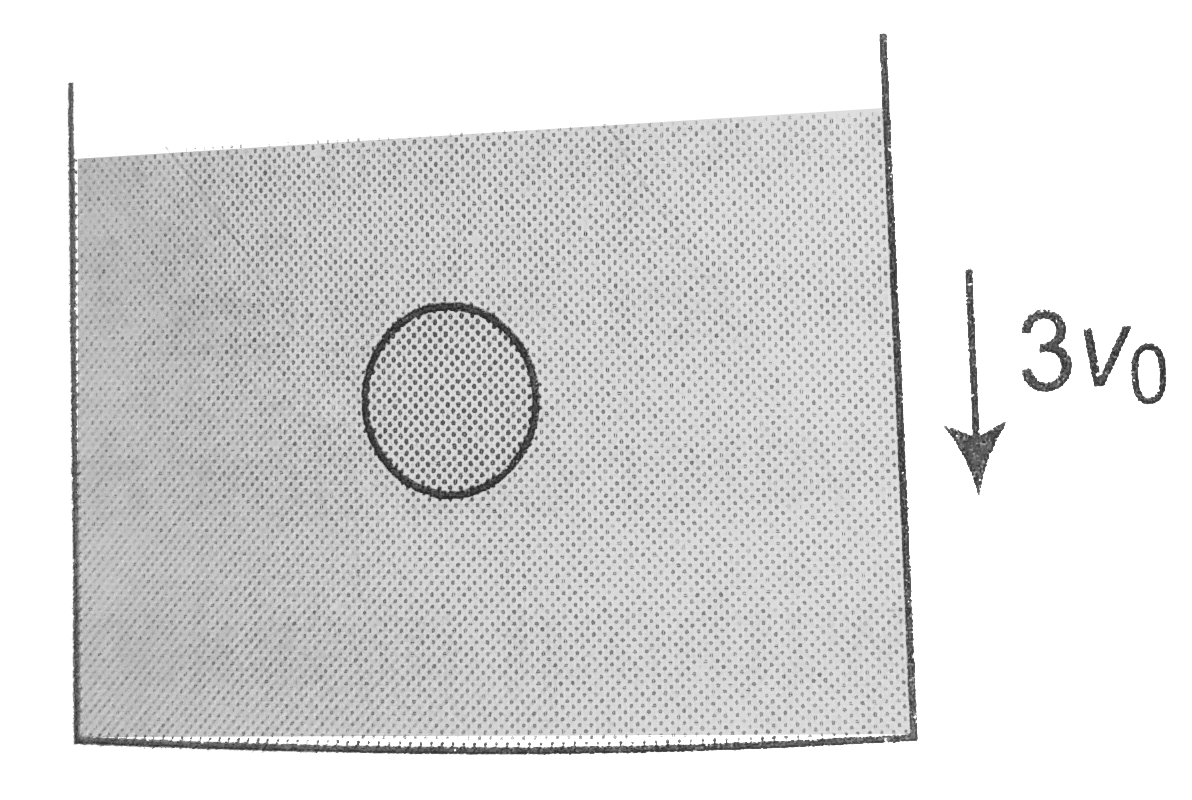
A container filled with viscous liquid is moving vertically downwards with constant speed 3v0. At the instant shown, a sphere of radius r is moving vertically downwards (in liquid) has speed v0. The coefficient of viscosity is η. There is no relative motion between the liquid and the container. Then at the shoen instant, The magnitude of viscous force acting on sphere isA6πηrv0B12πηrv0C18πηrv0D24πηrv0A shell is fired vertically upwards with a velocity v1 from a trolley moving horizontally with velocity v2. A person on the the ground observes the motion of the shell as a parabole, whose horizontal range is
A2v21v2gB2v21gC2v22gD2v1v2g
When a satellite is moving around the earth with velocity v, then to make the satellite escape, the minimum percentage increase in its velocity should be
View SolutionA particle moves vertically with an upward initial speed v0=5m/s. If its acceleration varies with time as shwon in a−t graph in the fig. 4.30, find the velocity of the particle at t=7s.

View SolutionAt the initial moment three points A, B and C are on a horizontal straight line at equal distances from one another. Point A begins to move vertically upward with a constant velocity v and point C vertically downward without any initial velocity but with a constant acceleration a. How should point B move vertically for all the three points to be constantly on one straight line. The points begin to move simultaneously.
View SolutionA particle moves vertically with an upwards initial speed v0=10.5ms−1. If its acceleration varies with time as shown in a-t graph in figure, find the velocity of the particles at t = 4s.
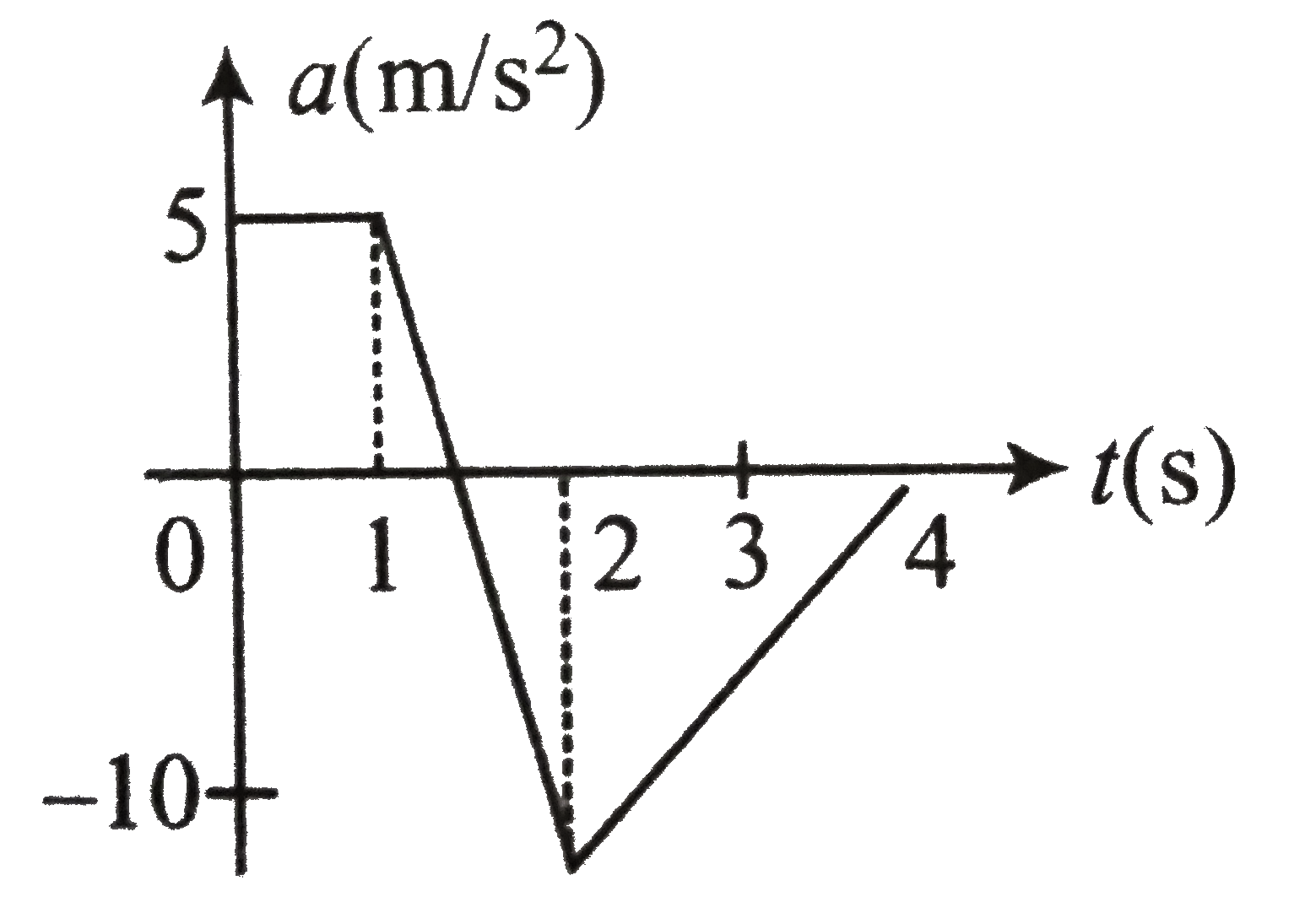
View SolutionA uniform rectangular block of dimensions shown in fig is sliding on the horizontal surface with a velocity v when it strikes a small kerb in the surface. Determine the minimum value of v for which the block will pivot about the kerb and just reach the vertical position with no velocity. Assume negligible rebound at the step.

View Solution
