By what fraction does the mass of a spring is 200 g at its natural lenth and the spring constant is 500 N m ^(-1)
Given
Energy stored
Increasein mass
Fractional Change of mass
.
Topper's Solved these Questions
- THE SPECIAL THEORY OF RELATIVITYBook:HC VERMAChapter:THE SPECIAL THEORY OF RELATIVITYExercise:Exercise
Explore 1 Video
- THE SPECIAL THEORY OF RELATIVITYBook:HC VERMAChapter:THE SPECIAL THEORY OF RELATIVITYExercise:Objective 2
Explore 6 Videos
- THE NUCLEOUSBook:HC VERMAChapter:THE NUCLEOUSExercise:Exercises
Explore 53 Videos
- THERMAL AND CHEMICAL EFFECT OF ELECTRIC CURRENTBook:HC VERMAChapter:THERMAL AND CHEMICAL EFFECT OF ELECTRIC CURRENTExercise:Exercises
Explore 24 Videos
Similar Questions
A block of mass 3kg is attached with an ideal spring of spring constant 900 N/m. The other end of the spring connected with a rigid wall. A fixed convex mirror of radius of curvature 120 cm is placed at a distance 110 cm from the block and the spring is in its natural length. the spring is extended by 100 cm and released. the speed of the image of block is (15n)ms, when extension in the spring remains 50 cm. Find the value of 'n'.

View SolutionA mass m=8kg is attached to a spring passing over a pulled whose other end is fixed to ground and held in position so that the spring remains unstretched. The spring constant is 200N/m. The mass m is then released and begins to undergo small oscillations. Find maximum velocity of mass
View Solution
Knowledge Check
A mass M=5 kg is attached to a string as shown in the figure and held in position so that the spring remains unstretched. The spring constant is 200Nm−1 . The mass M is then released and begins to undergo small oscillations. The amplitude of oscillation is
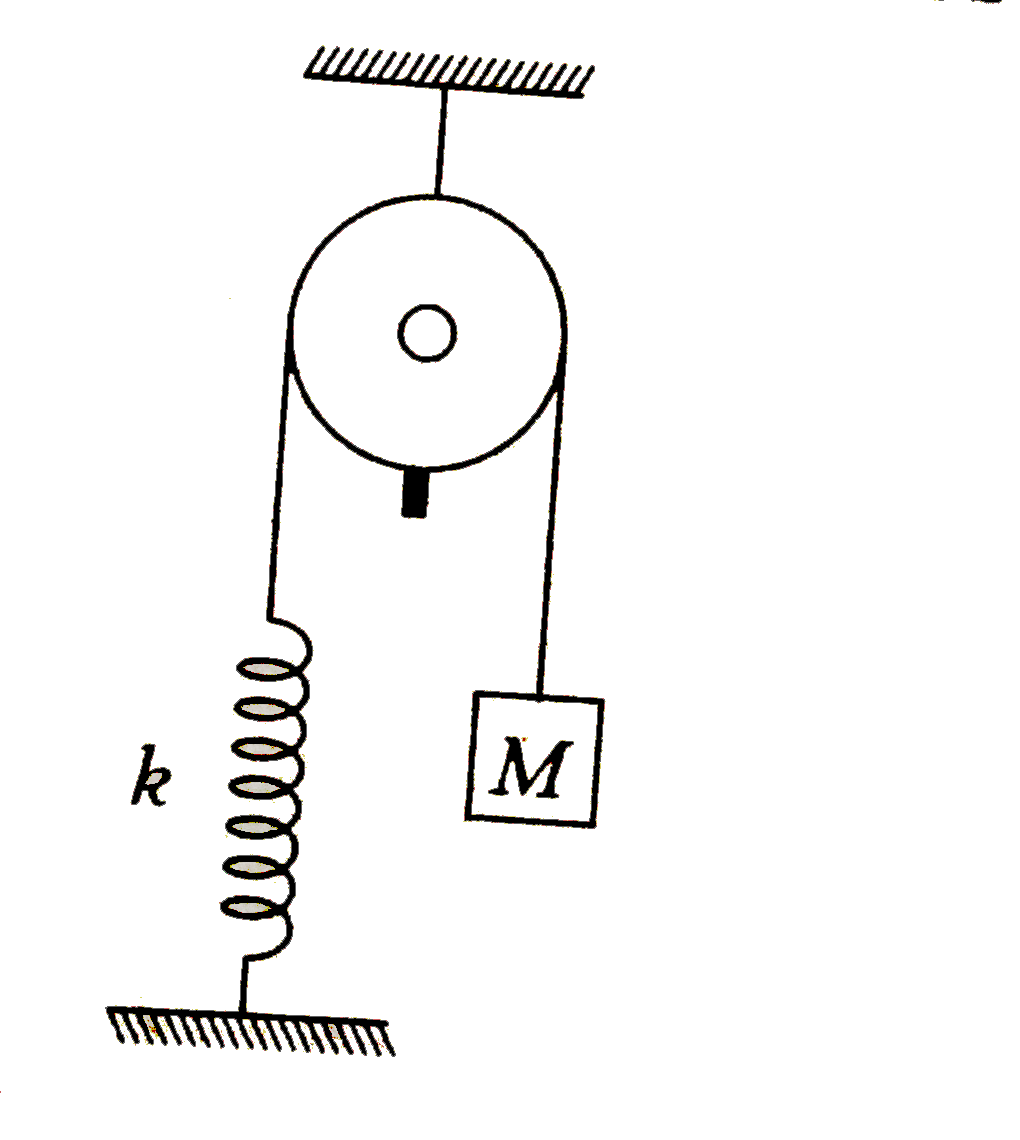 A0.5 mB0.25 mC0.2 mD0.1 m
A0.5 mB0.25 mC0.2 mD0.1 mFor the small damping oscillator, the mass of the block is 500 g and value of spring constant is k = 50 N/m and damping constant is 10 g s^(-1) The time period of oscillation is (approx.)
A2πsB2π13sCπ5sDπ√5sA mass m=8kg is attached to a spring as shown in figure and held in position so that the spring remains unstretched. The spring constant is 200N/m. The mass m is then released and begins to undergo small oscillations. The maximum velocity of the mass will be (g=10m/s2)
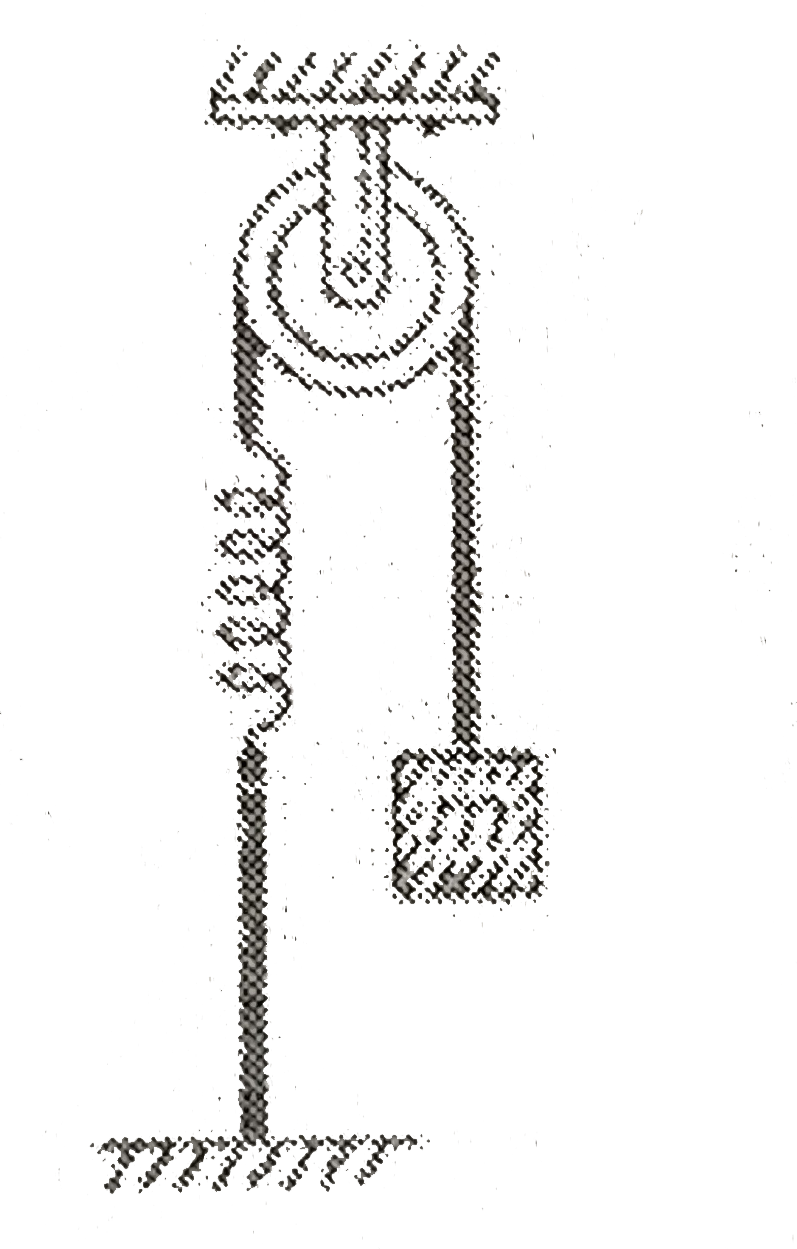 A1 m/sB2 m/sC4 m/sD5 m/s
A1 m/sB2 m/sC4 m/sD5 m/s
The spring constant of a spring depends on its
View SolutionWhat will be the spring constant k of a. spring if length of the spring is made n times?
View SolutionA spring is compressed by 10 cm by a ball of mass 20 g . What will be the potential energy of a spring if the force constant of the spring is 20 N cm−1 .
View SolutionThe system is released from rest with spring intially in its natural length. If mass of the block m = 10 kg. and spring constant k=100N/m, then maximum extension in spring is :
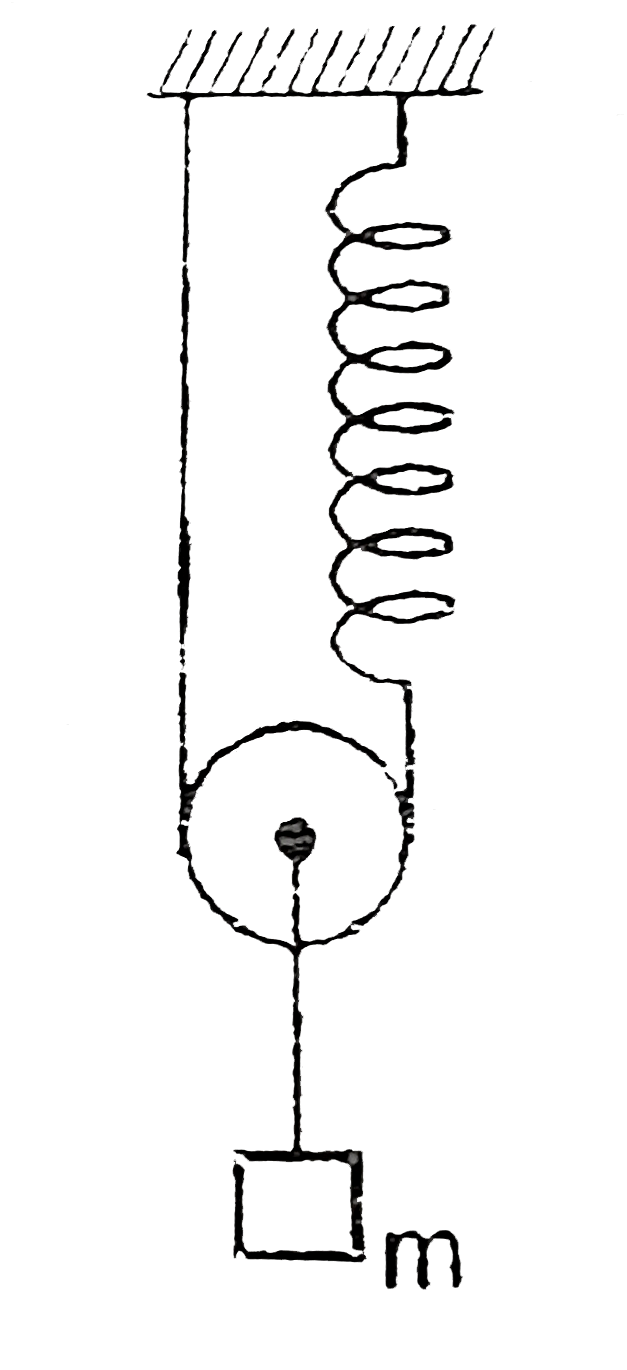
View SolutionTwo identical blocks A and B , each of mass m resting on smooth floor are connected by a light spring of natural length L and spring constant k, with the spring at its natural length. A third identical block C (mass m) moving with a speed v along the line joining A and B collides with A. The maximum compression in the spring is
View Solution