NEWTON'S LAW OF COOLING
Step by step video & image solution for NEWTON'S LAW OF COOLING by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
A hot liquid is kept in a bog room. Accoding to Newton's law of cooling rate of cooling liquid (represented as y) is plotted against its temperature T. Which of the following curves may represent the plot?
A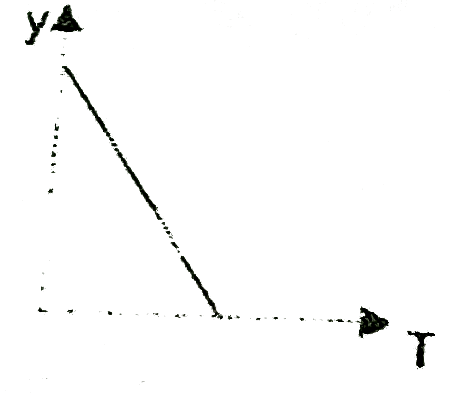 B
B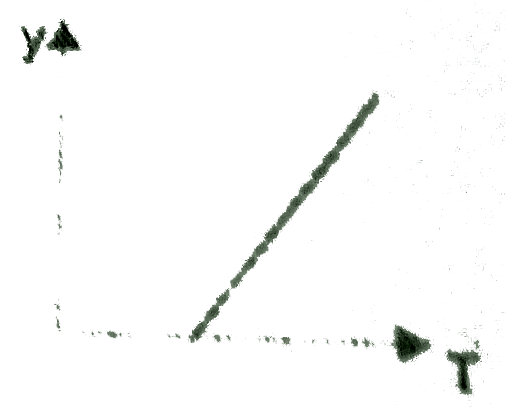 C
C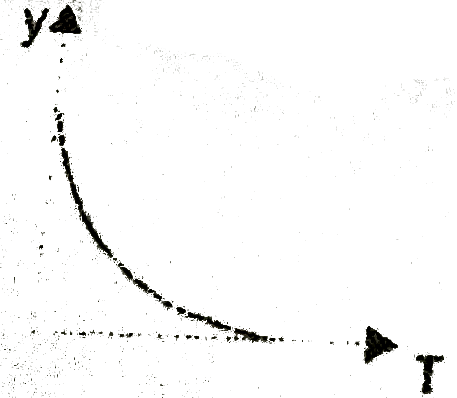 D
D
A body with an initial temperature θ1 is allowed to cool in a surrounding which is at a constant temperature of θ0(θ<θ1) Assume that Newton's law of cooling is obeyed Let k= constant The temperature of the body after time t is best experssed by .
A(θi−θ0)e−ktB(θi−θ0)In(kt)Cθ0+(θi−θ0)e−ktDθie−kt−θ0
Ac cording to Newton's law of cooling, the rate of cooling of a body is proportional to (Δθ)n, where Δθ is the difference of the temperature of the body and thae surrounding. What is the value of n out of 4,3,2,and 1?
View SolutionA body cools in 7 minutes from 60∘C to 40∘C. What will be its temperature after the next 7 minutes? The temperature of the surrounding is 10∘C. Assume that Newton's law of cooling holds good throughout the process.
View SolutionA calorimeter containing a hot lilquid is placed inside an enclosure whose walls are at 10∘C and cools from 80∘C to 60∘C in 10 minutes. How long will it take to cool from 60∘C to 40∘C, if Newton's law of cooling holds good ?
View SolutionA hot fbody placed in air is cooled down according to Newton's law of cooling, the rate of decrease of temperature being k times the temperature difference from the surrounding. Starting from t=0 , find the time in which the body will loss half the maximum heat it can lose.
View SolutionA hot body placed in air is cooled down according to newton's law of cooling the rate of decrease of temperature being k times the temperature difference from the surrounnding Starting from t=0 The time in which the body will lose half of the maximum heat is xLn22k Find the value of x .
View SolutionA container with 1 kg of water in it kept in sunlight , which causes the water to get warmer than the surroundings . The average energy per time per unit area received due to the sunlight is 700Wm−2 and it is absorbed by the water over an effective area of 0.05m2 . Assuming that the heat loss from the water to the surroundings is governed by Newton's law of cooling , the difference (in ∘C) in the temperature of water and the surroundings after a long time will be ________ . (Ignore effect of the container, and take constant for Newton's law of cooling = 0.001s−1 , Heat capacity of water = 4200 Jkg−1K−1)
View Solution