Two intersecting circles have a common tangent or not?
Step by step video & image solution for Two intersecting circles have a common tangent or not? by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Column I|Column II Two intersecting circle|p. have a common tangent Two mutually external circles|q. have a common normal Two circles, one strictly inside the other|r. do not have a common tangent Two branches of a hyperbola|s. do not have a common normal
View SolutionIn the given figure, MN is the common chord of two intersecting circles and AB is their common tangent.
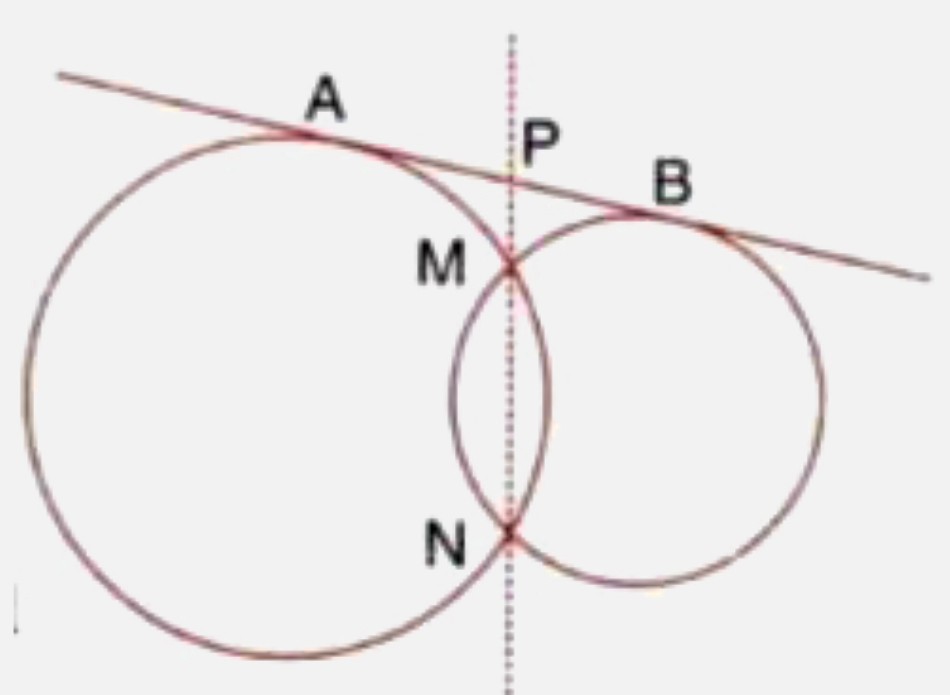
Prove that the line NM produced bisects AB at P.
View Solution
Knowledge Check
STATEMENT-1 : Let x2+y2=a2and x2+y2−6x−8y−11=0 be two circles. If a = 5 then two common tangents are possible.
and
STATEMENT-2 : If two circles are intersecting then they have two common tangents.AStatement-1 is true, statement-2 is true, Statement -2 is a correct explanation for Statement -1BStatement -1 is true, Statement-2 is true , Statement-2 is NOT a correct explanation for statement-1CStatement-1 is true, Statement-2 is FalseDStatement-1 is False, Statement-2 is true
Two circles of radii a and b (a < b) touch externally. Length of the direct common tangent from the point of intersection of the direct common tangents to the smaller circle is
View SolutionIntersecting lines
View SolutionIf from any point on the common chord of two intersecting circles, tangents be drawn to the circles, prove that they are equal.
View SolutionSTATEMENT-1 : Let x2+y2=a2and x2+y2−6x−8y−11=0 be two circles. If a = 5 then two common tangents are possible.
and
STATEMENT-2 : If two circles are intersecting then they have two common tangents.
View SolutionIf from any point on the common chord of two intersecting circles, tangents be drawn to the circles, prove that they are equal.
View SolutionThe point of intersection of direct common tangents and transverse common tangents to two circles divide the line segmenta joining the two centres externally and internally respectively in the ratio of their radii.
View SolutionIn the figure, two circles touch each other at the point C.Prove that the common tangents to the circles at C, bisect the common tangents at P and Q.
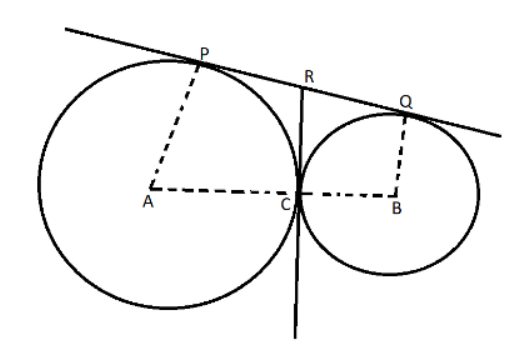
View Solution
