∫2−2min(x−[x],−x−[x])dx equals, where [x] represents greates integer less than or equal to x.
A
2
B
1
C
4
D
0
Video Solution
Text Solution
The correct Answer is:B
The graph of f(x)=min(x−[x],−x−[−x])dx is as shown in fig.9
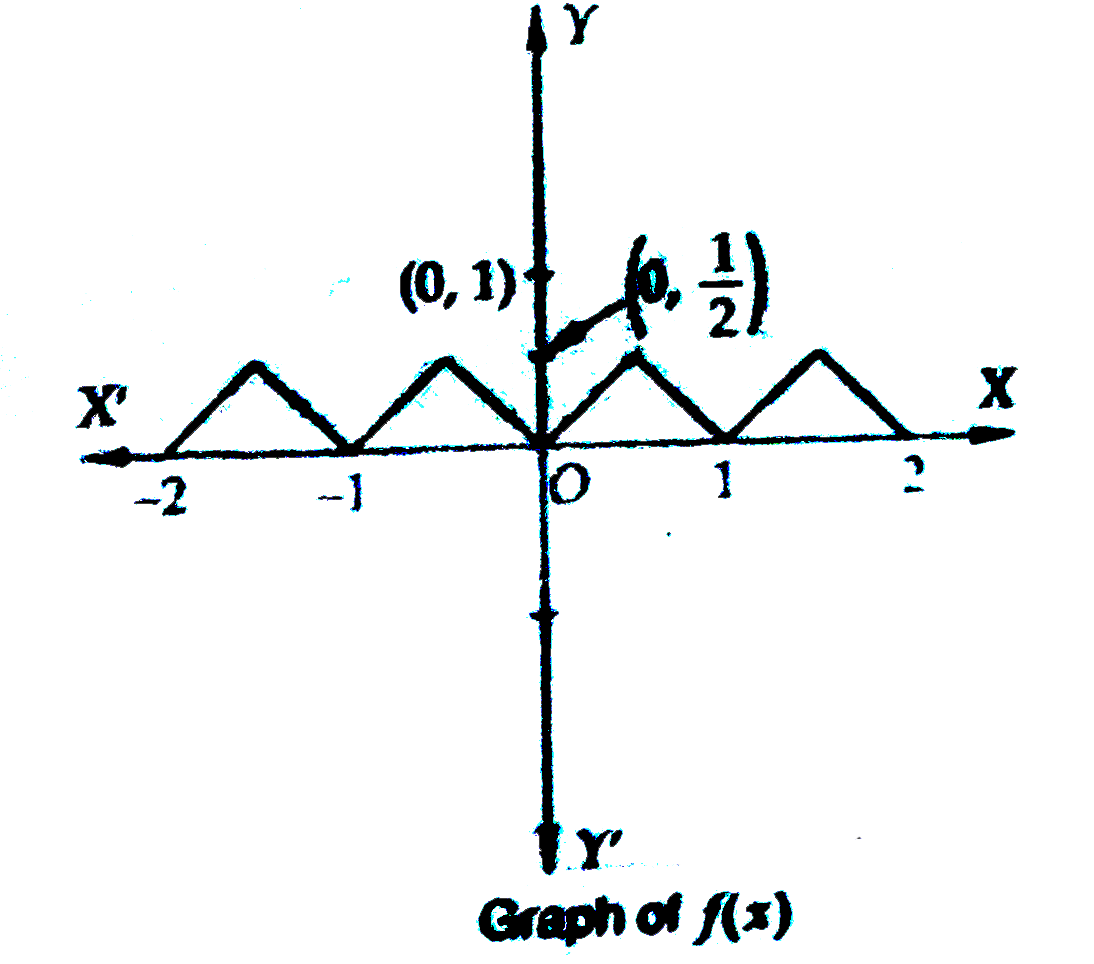
2∫−2f(x)dx=41∫0f(x)dx=4×12×1×12=1
|
Topper's Solved these Questions
- DEFINITE INTEGRALSBOOK - OBJECTIVE RD SHARMACHAPTER - DEFINITE INTEGRALSEXERCISE - Section II - Assertion Reason Type
12 Videos
- DEFINITE INTEGRALSBOOK - OBJECTIVE RD SHARMACHAPTER - DEFINITE INTEGRALSEXERCISE - Exercise
147 Videos
- DEFINITE INTEGRALSBOOK - OBJECTIVE RD SHARMACHAPTER - DEFINITE INTEGRALSEXERCISE - Chapter Test 2
60 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMACHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise
86 Videos
- DERIVATIVE AS A RATE MEASURER BOOK - OBJECTIVE RD SHARMACHAPTER - DERIVATIVE AS A RATE MEASURER EXERCISE - Exercise
26 Videos
Similar Questions
Knowledge Check
The value of ∫20x[x2+1]dx, where [x] is the greatest integer less than or equal to x is
A2B8/3C4Dnone of theseIf f(x)=|x−1|−[x], where [x] is the greatest integer less than or equal to x, then
Alimx→0[f(x)]=0Blimx→0[f(x)]=1Climx→0[f(x)x] does not existDlimx→0[f(x)x] existsIf I=∫1−1{[x2]+log(2+x2−x)}dx where [x] denotes the greatest integer less than or equal to x, the I equals
A-2B-1C0D1
∫1520[x−1]dx= where [x] denotes the greatest integer less than or equal to x
View SolutionIf f(x)=|x−1|−[x] (where [x] is greatest integer less than or equal to x) then.
View SolutionLet f(x)=x2−9x+20x−[x] where [x] denotes greatest integer less than or equal to x), then
View SolutionThe value of the integral ∫2−2sin2x−2[xπ]+12dx (where [x] denotes the greatest integer less then or equal to x) is
View SolutionThe function f(x)={x2[1x2]x≠00x=0 where [x] represents the greatest integer less than or equal to x
View Solution
