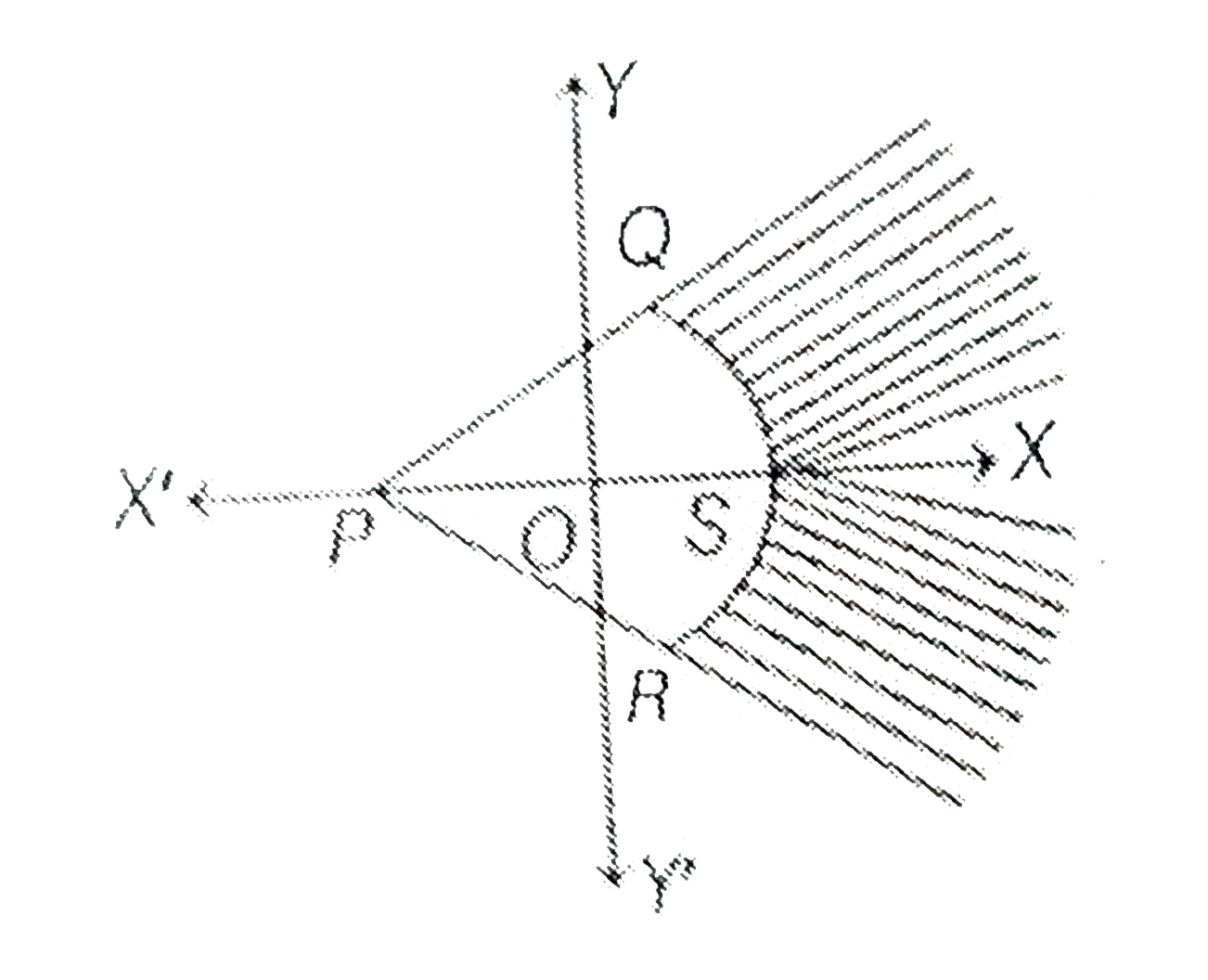
The shaded region, where P=(−1,0),Q=(−1+√2,√2)R=(−1+√2,−√2,S=(1,0) is represented by Figure |z+1|>2,∣arg(z+1)<π4 |z+1|⟨2,∣arg(z+1)<π2 |z+1|>2,∣arg(z+1)⟩π4 |z+1|⟨2,∣arg(z+1)>π2
|z+1|>2,|arg(z+1)|<π4
|z+1|<2,|arg(z+1)|<π4
|z−1|>2,|arg(z+1)|>π4
|z−1|<2,|arg(z+1)|>π2
The correct Answer is:A
We have,
PQ=PR=PS
∴ QR is an arc of the circle centred at P(−1,0) and radius 2 units.
Also, ∠QPS=∠RPS=π4
Therefore, any point z in the shaded region lies outside the circle |z+1|=2 and the line joining z and P(−1,0) makes angle less than 45∘ with X-axis on its either side.
Hence, |z+1|>2 and |arg(z+1)|<π4.

Topper's Solved these Questions
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Section II - Assertion Reason Type
15 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Exercise
131 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test
59 Videos
- CIRCLESBOOK - OBJECTIVE RD SHARMACHAPTER - CIRCLESEXERCISE - Chapter Test
55 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMACHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise
86 Videos
Similar Questions
The shaded region ,where P=(−1,0)Q=(−1+√2,√2)
R=(−1+√2,−√2),S=1(1,0) is represent by
View SolutionThe equation z(¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯z+1+i√3)+¯z(z+1+i√3)=0 represents a circle with
View SolutionProve that :
tan−1[√1+z+√1−z√1+z−√1−z]=π4+12cos−1z
View SolutionThe equation z2−i|z−1|2=0, where i=√−1,has.
View SolutionIf ∣∣∣z−4z∣∣∣=2 then the greatest value of |z| is (A) √5−1 (B) √5+1 (C) √5 (D) 2
View SolutionIf z=π4(1+i)4(1−√πi√π+i+√π−i1+√πi),then(|z|amp(z)) equal
View SolutionIf z+√2|z+1|+i=0andz=x+iy, then
View SolutionIf z=(1+i√3)24i(1−i√3) is a complex number then a. arg(z)=π4 b. arg(z)=π2 c. |z|=12 d. |z|=2
View SolutionIf |z1|=2,(1−i)z2+(1+i)¯z2=8√2, (z1,z2 are complex variables) then the minimum value of |z1−z2|,is
View SolutionWhat is locus of z if ∣∣ ∣∣z−1−sin−1(1√3)∣∣ ∣∣+∣∣ ∣∣z+cos−1(1√3)−π2∣∣ ∣∣=1?
View Solution
