A compound made of particles A, B, and C forms p lattice. In the lattice, ions A occupy the lattice points and ions B and C occuphy the alternate TVs. If all the ions along one of the body diagonals are removed, then formula of the compound is
A3.75B3C3
A3.75B3C4
A3B3.75C3
A3B3C3.75
The correct Answer is:A
To solve the problem, we need to analyze the information given about the compound made of particles A, B, and C that forms a cubic close-packed (ccp) lattice. Let's break down the solution step by step.
Step 1: Determine the number of A ions in the ccp lattice
In a cubic close-packed (ccp) lattice, also known as face-centered cubic (FCC), the number of atoms per unit cell is 4. This is because:
- There are 8 corner atoms, each contributing 18 to the unit cell.
- There are 6 face-centered atoms, each contributing 12 to the unit cell.
Calculating the total:
Total A atoms=8×18+6×12=1+3=4
Thus, there are 4 A ions in the unit cell.
Step 2: Determine the number of tetrahedral voids
In a ccp lattice, there are 8 tetrahedral voids per unit cell. However, since the problem states that ions B and C occupy alternate tetrahedral voids, we need to consider only half of these voids:
Number of tetrahedral voids occupied=82=4
So, there are 4 tetrahedral voids occupied by B and C.
Step 3: Analyze the removal of ions along the body diagonal
When all the ions along one body diagonal are removed, we need to consider how many A ions are affected. In a ccp lattice, the body diagonal passes through:
- 2 corner atoms (A)
- 1 face-centered atom (A)
Thus, the total number of A ions removed is:
Removed A ions=2 (from corners)+1 (from face center)=3
Step 4: Calculate the remaining A ions
Initially, there were 4 A ions. After removing 3 A ions:
Remaining A ions=4−3=1
Step 5: Calculate the remaining B and C ions
Since one tetrahedral void is removed when the body diagonal is removed, one of the ions (either B or C) occupying that void will also be removed. Initially, there were 4 ions of B and 4 ions of C:
- After removing one tetrahedral void, we have:
Remaining B ions=4−1=3
Remaining C ions=4 (unchanged)
Step 6: Write the formula of the compound
Now we can write the formula of the compound based on the remaining ions:
Formula=A1B3C4
Final Answer
The final formula of the compound after the removal of the ions along the body diagonal is:
Formula=A1B3C4
In a cubic close-packed (ccp) lattice, also known as face-centered cubic (FCC), the number of atoms per unit cell is 4. This is because:
- There are 8 corner atoms, each contributing 18 to the unit cell.
- There are 6 face-centered atoms, each contributing 12 to the unit cell.
Total A atoms=8×18+6×12=1+3=4
Thus, there are 4 A ions in the unit cell.
In a ccp lattice, there are 8 tetrahedral voids per unit cell. However, since the problem states that ions B and C occupy alternate tetrahedral voids, we need to consider only half of these voids:
Number of tetrahedral voids occupied=82=4
So, there are 4 tetrahedral voids occupied by B and C.
When all the ions along one body diagonal are removed, we need to consider how many A ions are affected. In a ccp lattice, the body diagonal passes through:
- 2 corner atoms (A)
- 1 face-centered atom (A)
Removed A ions=2 (from corners)+1 (from face center)=3
Initially, there were 4 A ions. After removing 3 A ions:
Remaining A ions=4−3=1
Since one tetrahedral void is removed when the body diagonal is removed, one of the ions (either B or C) occupying that void will also be removed. Initially, there were 4 ions of B and 4 ions of C:
- After removing one tetrahedral void, we have:
Remaining B ions=4−1=3
Remaining C ions=4 (unchanged)
Now we can write the formula of the compound based on the remaining ions:
Formula=A1B3C4
The final formula of the compound after the removal of the ions along the body diagonal is:
Formula=A1B3C4
Topper's Solved these Questions
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Solved Examples13videos
SOLID STATE
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercises (Linked Comprehension)13videos
SOLID STATE
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDSEXERCISE - SUBJECTIVE TYPE4videos
REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDS
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLUTIONSEXERCISE - Ex 2.3 (Objective)9videos
SOLUTIONS
Similar Questions
A compound made of particles A, B, and C forms p lattice. Ions A are at lattice points, B occupy TVsC occupy OVs. If all the ions along one of the edge axis are removed, then formula of the compound is
View SolutionA2B has antifluorite structure (B forms FCC lattice and A occupies tetrahedral voids).If all ions along any one body diagonal are removed, then new formula of compound will be :
View Solution
Knowledge Check
- Question 1 - Select One
In a face centered cubic lattice, atom (A) occupies the corner positions and atom (B) occupies the face centre positions. If one atom of (B) is missing from one of the face centered points, the formula of the compound is :
AA2B5BA2B3CAB2DA2B
A crystal is made of particles X,Y and Z.X form fcc packing . Y occupies all the octahedral void of X and Z occupies all the tetrahedral voids of X . If all the particles along one body diagonal are removed then the formula of the crystal would be:
View SolutionA crystal is made of particles X and Y.X form fcc packing and Y occupies all the octahedral voids . If all the particles along one body diagonal are removed then the formula of the crystal would de :
View SolutionIn a crystalline solid, atoms of X form fcc packing and atoms of Y occupy all octahedral voids. If all the atoms along one body diagonal are removed, what is the simplest formula of the crystalline lattice solid?
View SolutionIn a face centred cubic lattice, atom A occupies the corner positions and atom B occupies the face centred positions. If one atom of B is missin from one of the face centred points,, the formula of the compound is :
View SolutionA face centred cubic is made up of two types of atoms A and B in which A occupies the corner positions and B occupies the face centres. If atoms along one of the body diagonal are removed, empirical formula of remainning solid will be
View SolutionA compound made of particles A and B. A forms fcc packing and B occupies all the OVs. If all the particles along the plane as shown in the figure below are removeed, then the simplest formula of the compound is
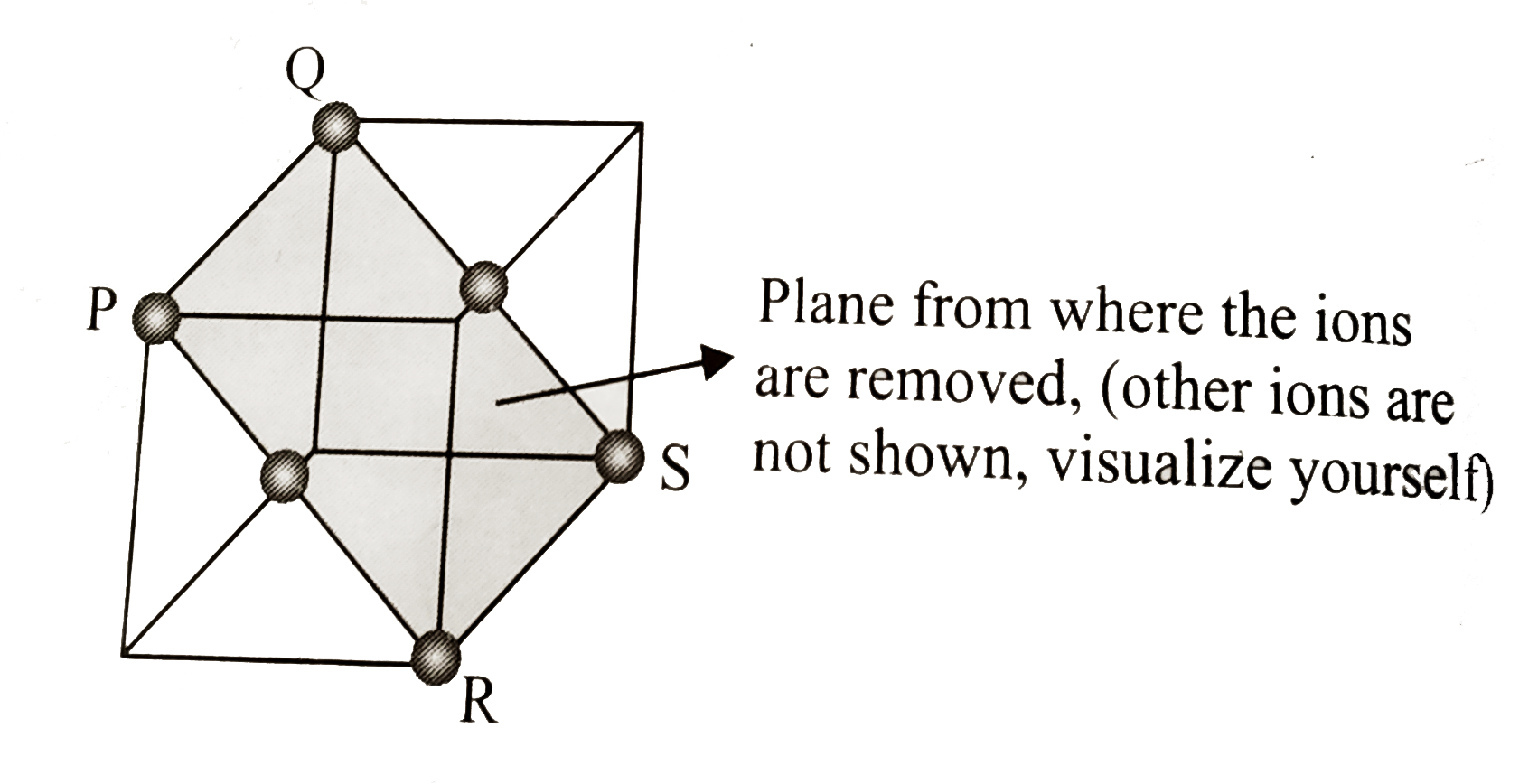 View Solution
View SolutionIn a face centered cubic lattice, atom (A) occupies the corner positions and atom (B) occupies the face centre positions. If one atom of (B) is missing from one of the face centered points, the formula of the compound is :
View Solution

