A radioactive substance consists of two distinct having equal number of atoms initially. The mean products in both cases are stable. A plot is made of total number of radioactive nuclei as a function of time. Which of the following figures best represents the form of this plot?
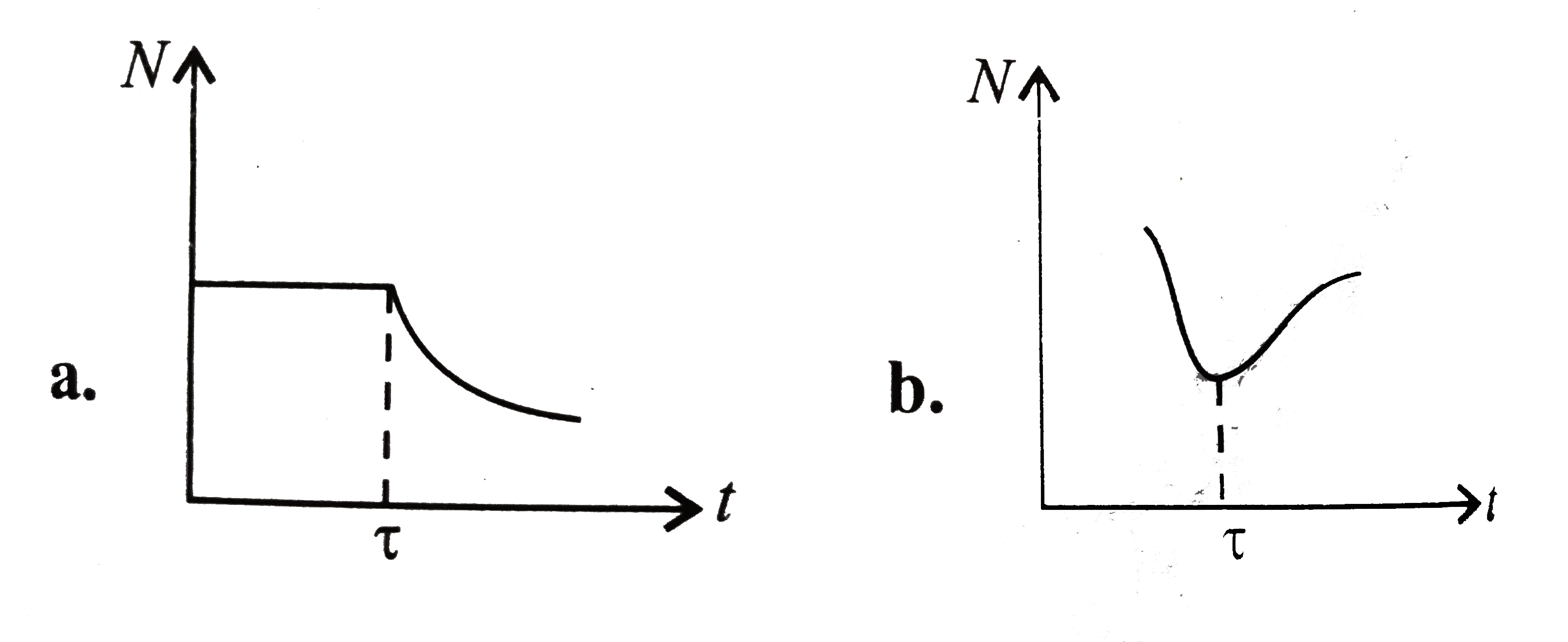
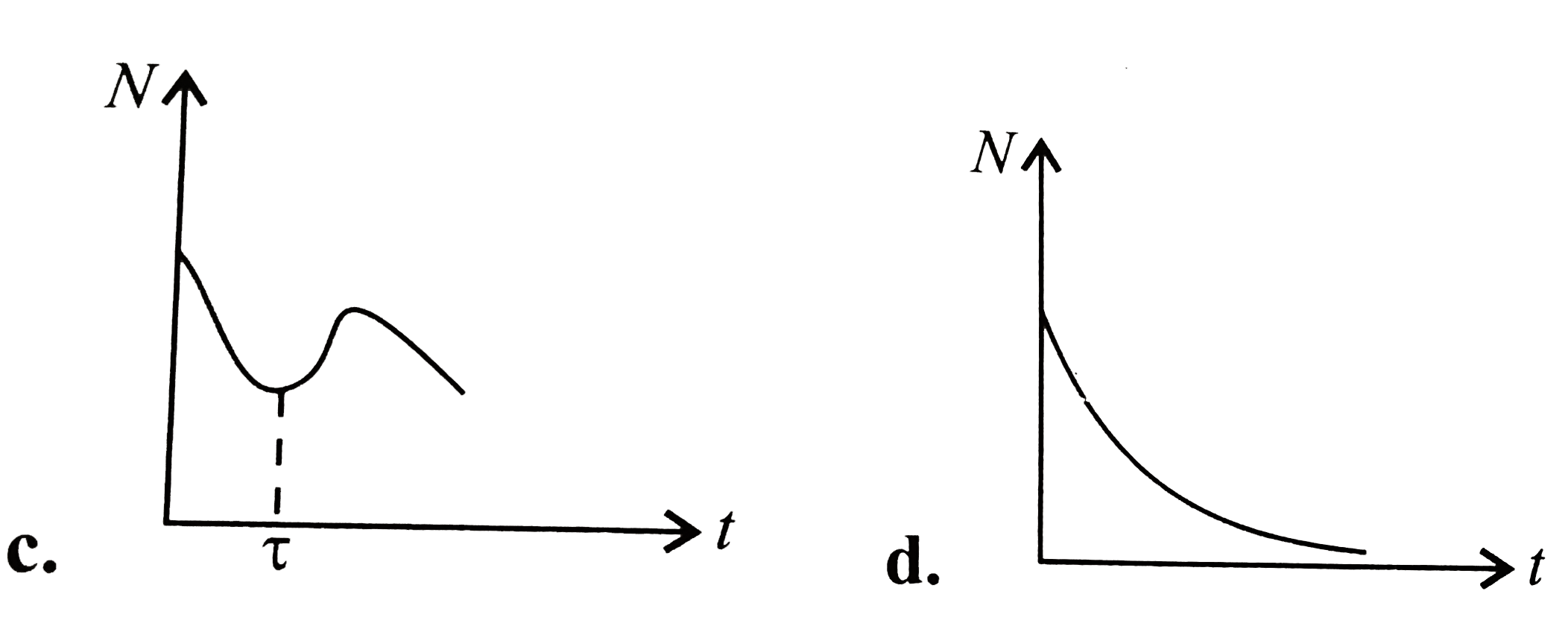
The decrease of radioactive element (parent element) follows the exponential functions.
Topper's Solved these Questions
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Solved Example18videos
NUCLEAR CHEMISTRY
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Ex6.1 Objective15videos
NUCLEAR CHEMISTRY
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NCERT BASED EXERCISEEXERCISE - Nuclear Chemistry (NCERT Exercise)29videos
NCERT BASED EXERCISE
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ORGANIC COMPOUNDS WITH FUNCTIONAL GROUPEXERCISE - Archives Analytical And Descriptive24videos
ORGANIC COMPOUNDS WITH FUNCTIONAL GROUP
Similar Questions
A radioactive sample consists of two distinct species having equal number of atoms initially. The mean life of one species is τ and that of the other is 5τ. The decay products in both cases are stable. A plot is made of the total number of radioactive nuclei as a function of time. Which of the following figure best represents the form of this plot?
(a), (b), (c), (d)
 View Solution
View SolutionIn a radioactive disintegration, the ratio of initial number of atoms to the number of atoms present at an instant of time equal to its mean life is
View Solution
Knowledge Check
- Question 1 - Select One
A new fitness class was started at a chain of fitness clubs owned by the same company. The scatter plot above shows the total number of people attending the class during the first 5 months in which the class was offered. The line of best fit is drawn. If n is the number of the month, which of the following functions could represent the equation of the graph's line of best fit?
Af(x)=300n+125Bf(n)=300+125nCf(n)=400+150nDf(n)=200n+300
Two radioactive substances X and Y initially contain equal number of atoms. Their half-lives are 1 hour and 2 hours respectively. Calculate the ratio of their rates of disintegration after four hours.
View SolutionTwo radioactive substances X and Y initially contain an equal number of atoms. Their half-lives are 1 hour and 2 hours respectively. Then the ratio of their rates of disintergration after two hours is
View SolutionTwo radioactive substance X and Y initially contain equal number of nuclei. X has a half life of 1 hour and Y has a half-life of 2 hours. After 2 hours the ratio of activity of X to that of y is
View SolutionTwo radioactive substances have half-lives T and 2T. Initially, they have equal number of nuclei. After time t=4T, the ratio of their number of nuclei is x and the ratio of their activity is y. Then,
View SolutionTwo radioactive samples 1 and 2 have equal number of nuclei initially. They have halg-lives of 10 seconds and 20 seconds. The ratio of number of nuclei of 1 and 2 at t=60 seconds is :
View SolutionThere are two radioactive substance A and B. Decay consant of B is two times that of A. Initially, both have equal number of nuceli. After n half-lives of A,rates of disintegaration of both are equal. The value of n is .
View SolutionThe half - line period a radioactive element X is same as the mean life time of another radioactive element Y. Initially both of them have the same number of atoms. Then:
View Solution

